
Question Number 3370 by Filup last updated on 12/Dec/15
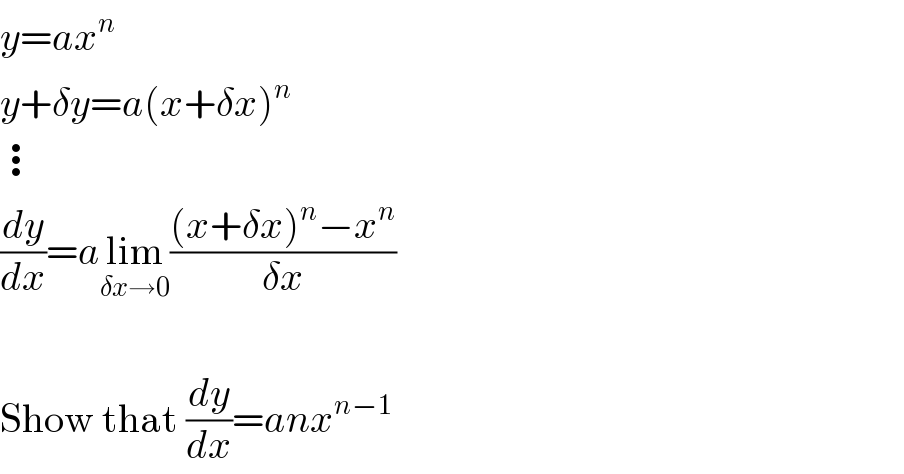
$${y}={ax}^{{n}} \\ $$$${y}+\delta{y}={a}\left({x}+\delta{x}\right)^{{n}} \\ $$$$\vdots \\ $$$$\frac{{dy}}{{dx}}={a}\underset{\delta{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{\left({x}+\delta{x}\right)^{{n}} −{x}^{{n}} }{\delta{x}} \\ $$$$ \\ $$$$\mathrm{Show}\:\mathrm{that}\:\frac{{dy}}{{dx}}={anx}^{{n}−\mathrm{1}} \\ $$
Answered by Yozzi last updated on 12/Dec/15
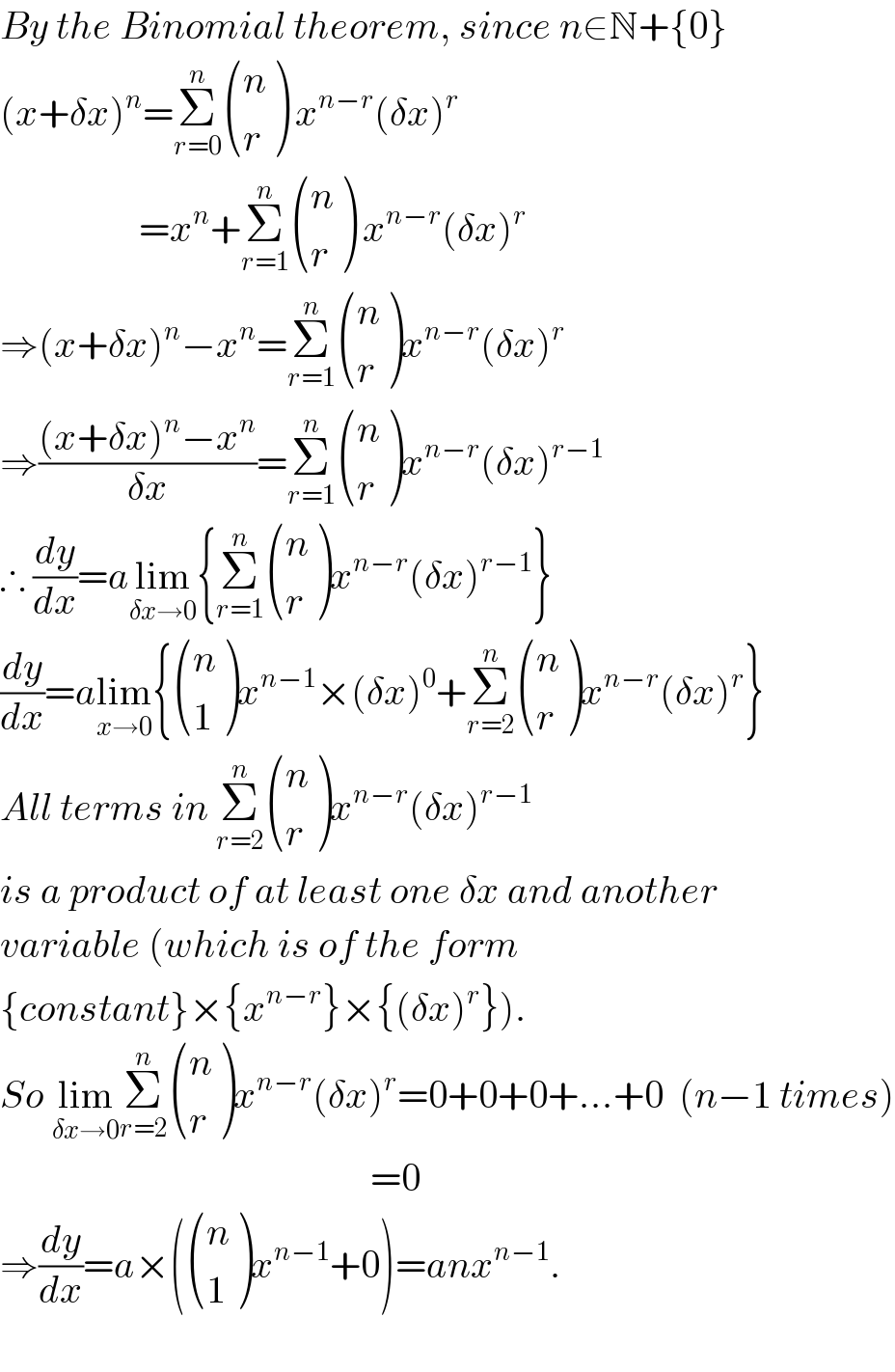
$${By}\:{the}\:{Binomial}\:{theorem},\:{since}\:{n}\in\mathbb{N}+\left\{\mathrm{0}\right\} \\ $$$$\left({x}+\delta{x}\right)^{{n}} =\underset{{r}=\mathrm{0}} {\overset{{n}} {\sum}}\begin{pmatrix}{{n}}\\{{r}}\end{pmatrix}\:{x}^{{n}−{r}} \left(\delta{x}\right)^{{r}} \:\:\: \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:={x}^{{n}} +\underset{{r}=\mathrm{1}} {\overset{{n}} {\sum}}\begin{pmatrix}{{n}}\\{{r}}\end{pmatrix}\:{x}^{{n}−{r}} \left(\delta{x}\right)^{{r}} \\ $$$$\Rightarrow\left({x}+\delta{x}\right)^{{n}} −{x}^{{n}} =\underset{{r}=\mathrm{1}} {\overset{{n}} {\sum}}\begin{pmatrix}{{n}}\\{{r}}\end{pmatrix}{x}^{{n}−{r}} \left(\delta{x}\right)^{{r}} \\ $$$$\Rightarrow\frac{\left({x}+\delta{x}\right)^{{n}} −{x}^{{n}} }{\delta{x}}=\underset{{r}=\mathrm{1}} {\overset{{n}} {\sum}}\begin{pmatrix}{{n}}\\{{r}}\end{pmatrix}{x}^{{n}−{r}} \left(\delta{x}\right)^{{r}−\mathrm{1}} \\ $$$$\therefore\:\frac{{dy}}{{dx}}={a}\underset{\delta{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\left\{\underset{{r}=\mathrm{1}} {\overset{{n}} {\sum}}\begin{pmatrix}{{n}}\\{{r}}\end{pmatrix}{x}^{{n}−{r}} \left(\delta{x}\right)^{{r}−\mathrm{1}} \right\} \\ $$$$\frac{{dy}}{{dx}}={a}\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\left\{\begin{pmatrix}{{n}}\\{\mathrm{1}}\end{pmatrix}{x}^{{n}−\mathrm{1}} ×\left(\delta{x}\right)^{\mathrm{0}} +\underset{{r}=\mathrm{2}} {\overset{{n}} {\sum}}\begin{pmatrix}{{n}}\\{{r}}\end{pmatrix}{x}^{{n}−{r}} \left(\delta{x}\right)^{{r}} \right\} \\ $$$${All}\:{terms}\:{in}\:\underset{{r}=\mathrm{2}} {\overset{{n}} {\sum}}\begin{pmatrix}{{n}}\\{{r}}\end{pmatrix}{x}^{{n}−{r}} \left(\delta{x}\right)^{{r}−\mathrm{1}} \\ $$$${is}\:{a}\:{product}\:{of}\:{at}\:{least}\:{one}\:\delta{x}\:{and}\:{another} \\ $$$${variable}\:\left({which}\:{is}\:{of}\:{the}\:{form}\:\right. \\ $$$$\left.\left\{{constant}\right\}×\left\{{x}^{{n}−{r}} \right\}×\left\{\left(\delta{x}\right)^{{r}} \right\}\right). \\ $$$${So}\:\underset{\delta{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\underset{{r}=\mathrm{2}} {\overset{{n}} {\sum}}\begin{pmatrix}{{n}}\\{{r}}\end{pmatrix}{x}^{{n}−{r}} \left(\delta{x}\right)^{{r}} =\mathrm{0}+\mathrm{0}+\mathrm{0}+...+\mathrm{0}\:\:\left({n}−\mathrm{1}\:{times}\right) \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:=\mathrm{0} \\ $$$$\Rightarrow\frac{{dy}}{{dx}}={a}×\left(\begin{pmatrix}{{n}}\\{\mathrm{1}}\end{pmatrix}{x}^{{n}−\mathrm{1}} +\mathrm{0}\right)={anx}^{{n}−\mathrm{1}} . \\ $$$$ \\ $$
Commented by Filup last updated on 12/Dec/15

$$\mathrm{nice}!\:\mathrm{i}\:\mathrm{thought}\:\mathrm{it}\:\mathrm{was}\:\mathrm{along}\:\mathrm{that}\:\mathrm{line} \\ $$$$\mathrm{of}\:\mathrm{thought}!\:\mathrm{thanks}\:<\mathrm{3} \\ $$
