Question and Answers Forum

All Questions      Topic List

None Questions

Previous in All Question      Next in All Question      

Previous in None      Next in None      

Question Number 139799 by Study last updated on 01/May/21

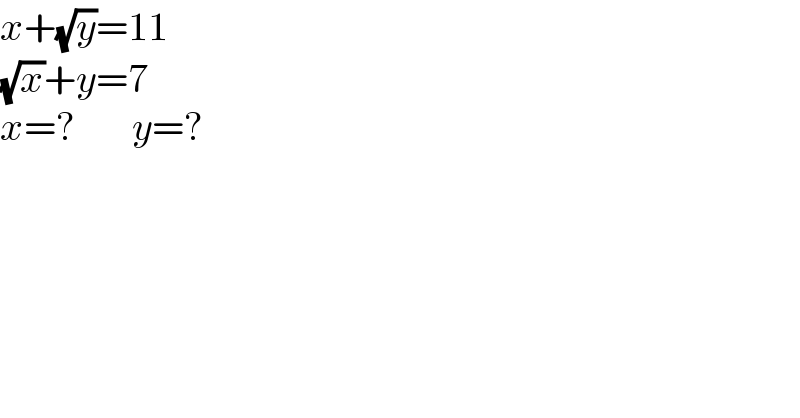
x+(√y)=11 (√x)+y=7 x=? y=?

$${x}+\sqrt{{y}}=\mathrm{11} \\ $$$$\sqrt{{x}}+{y}=\mathrm{7} \\ $$$${x}=?\:\:\:\:\:\:\:{y}=? \\ $$

Commented by MJS_new last updated on 01/May/21

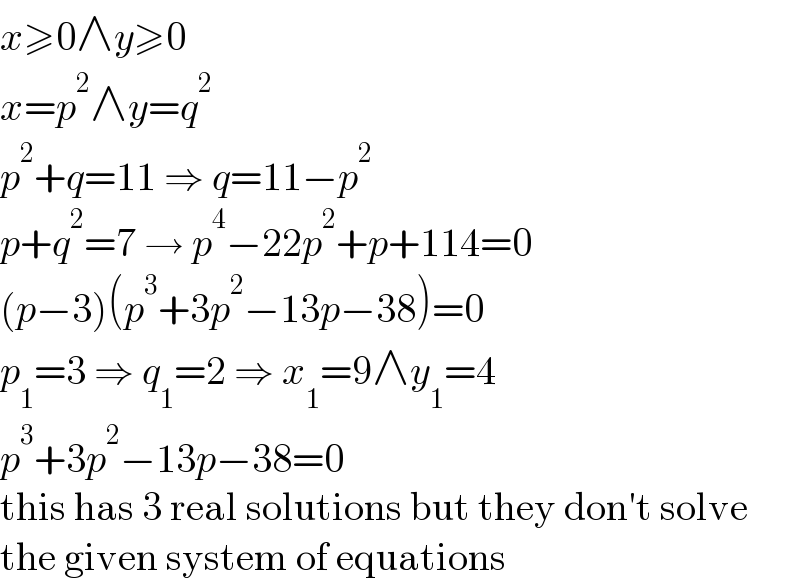
x≥0∧y≥0 x=p^2 ∧y=q^2 p^2 +q=11 ⇒ q=11−p^2 p+q^2 =7 → p^4 −22p^2 +p+114=0 (p−3)(p^3 +3p^2 −13p−38)=0 p_1 =3 ⇒ q_1 =2 ⇒ x_1 =9∧y_1 =4 p^3 +3p^2 −13p−38=0 this has 3 real solutions but they don′t solve the given system of equations

$${x}\geqslant\mathrm{0}\wedge{y}\geqslant\mathrm{0} \\ $$$${x}={p}^{\mathrm{2}} \wedge{y}={q}^{\mathrm{2}} \\ $$$${p}^{\mathrm{2}} +{q}=\mathrm{11}\:\Rightarrow\:{q}=\mathrm{11}−{p}^{\mathrm{2}} \\ $$$${p}+{q}^{\mathrm{2}} =\mathrm{7}\:\rightarrow\:{p}^{\mathrm{4}} −\mathrm{22}{p}^{\mathrm{2}} +{p}+\mathrm{114}=\mathrm{0} \\ $$$$\left({p}−\mathrm{3}\right)\left({p}^{\mathrm{3}} +\mathrm{3}{p}^{\mathrm{2}} −\mathrm{13}{p}−\mathrm{38}\right)=\mathrm{0} \\ $$$${p}_{\mathrm{1}} =\mathrm{3}\:\Rightarrow\:{q}_{\mathrm{1}} =\mathrm{2}\:\Rightarrow\:{x}_{\mathrm{1}} =\mathrm{9}\wedge{y}_{\mathrm{1}} =\mathrm{4} \\ $$$${p}^{\mathrm{3}} +\mathrm{3}{p}^{\mathrm{2}} −\mathrm{13}{p}−\mathrm{38}=\mathrm{0} \\ $$$$\mathrm{this}\:\mathrm{has}\:\mathrm{3}\:\mathrm{real}\:\mathrm{solutions}\:\mathrm{but}\:\mathrm{they}\:\mathrm{don}'\mathrm{t}\:\mathrm{solve} \\ $$$$\mathrm{the}\:\mathrm{given}\:\mathrm{system}\:\mathrm{of}\:\mathrm{equations} \\ $$

Answered by bramlexs22 last updated on 01/May/21

{ ((x=9)),((y=4)) :}

$$\begin{cases}{\mathrm{x}=\mathrm{9}}\\{\mathrm{y}=\mathrm{4}}\end{cases} \\ $$

Commented by Study last updated on 01/May/21

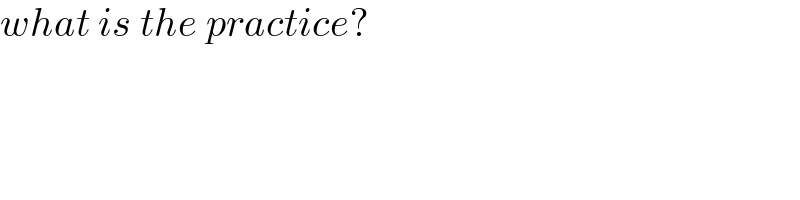
what is the practice?

$${what}\:{is}\:{the}\:{practice}? \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com