
Question Number 167492 by mathlove last updated on 18/Mar/22
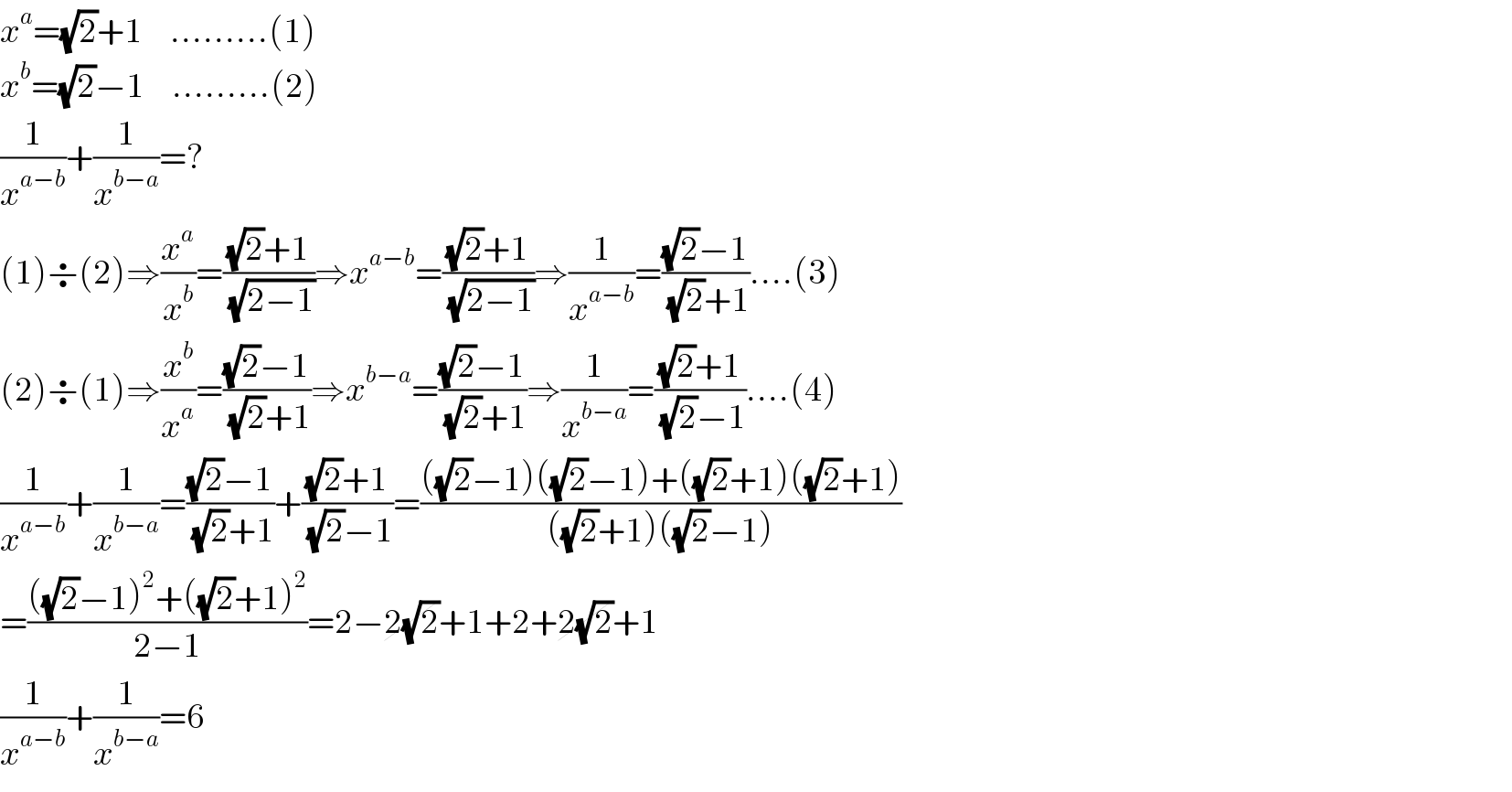
$${x}^{{a}} =\sqrt{\mathrm{2}}+\mathrm{1}\:\:\:\:\:.........\left(\mathrm{1}\right)\: \\ $$$${x}^{{b}} =\sqrt{\mathrm{2}}−\mathrm{1}\:\:\:\:\:.........\left(\mathrm{2}\right) \\ $$$$\frac{\mathrm{1}}{{x}^{{a}−{b}} }+\frac{\mathrm{1}}{{x}^{{b}−{a}} }=? \\ $$$$\left(\mathrm{1}\right)\boldsymbol{\div}\left(\mathrm{2}\right)\Rightarrow\frac{{x}^{{a}} }{{x}^{{b}} }=\frac{\sqrt{\mathrm{2}}+\mathrm{1}}{\:\sqrt{\mathrm{2}−\mathrm{1}}}\Rightarrow{x}^{{a}−{b}} =\frac{\sqrt{\mathrm{2}}+\mathrm{1}}{\:\sqrt{\mathrm{2}−\mathrm{1}}}\Rightarrow\frac{\mathrm{1}}{{x}^{{a}−{b}} }=\frac{\sqrt{\mathrm{2}}−\mathrm{1}}{\:\sqrt{\mathrm{2}}+\mathrm{1}}....\left(\mathrm{3}\right) \\ $$$$\left(\mathrm{2}\right)\boldsymbol{\div}\left(\mathrm{1}\right)\Rightarrow\frac{{x}^{{b}} }{{x}^{{a}} }=\frac{\sqrt{\mathrm{2}}−\mathrm{1}}{\:\sqrt{\mathrm{2}}+\mathrm{1}}\Rightarrow{x}^{{b}−{a}} =\frac{\sqrt{\mathrm{2}}−\mathrm{1}}{\:\sqrt{\mathrm{2}}+\mathrm{1}}\Rightarrow\frac{\mathrm{1}}{{x}^{{b}−{a}} }=\frac{\sqrt{\mathrm{2}}+\mathrm{1}}{\:\sqrt{\mathrm{2}}−\mathrm{1}}....\left(\mathrm{4}\right) \\ $$$$\frac{\mathrm{1}}{{x}^{{a}−{b}} }+\frac{\mathrm{1}}{{x}^{{b}−{a}} }=\frac{\sqrt{\mathrm{2}}−\mathrm{1}}{\:\sqrt{\mathrm{2}}+\mathrm{1}}+\frac{\sqrt{\mathrm{2}}+\mathrm{1}}{\:\sqrt{\mathrm{2}}−\mathrm{1}}=\frac{\left(\sqrt{\mathrm{2}}−\mathrm{1}\right)\left(\sqrt{\mathrm{2}}−\mathrm{1}\right)+\left(\sqrt{\mathrm{2}}+\mathrm{1}\right)\left(\sqrt{\mathrm{2}}+\mathrm{1}\right)}{\left(\sqrt{\mathrm{2}}+\mathrm{1}\right)\left(\sqrt{\mathrm{2}}−\mathrm{1}\right)} \\ $$$$=\frac{\left(\sqrt{\mathrm{2}}−\mathrm{1}\right)^{\mathrm{2}} +\left(\sqrt{\mathrm{2}}+\mathrm{1}\right)^{\mathrm{2}} }{\mathrm{2}−\mathrm{1}}=\mathrm{2}−\cancel{\mathrm{2}\sqrt{\mathrm{2}}}+\mathrm{1}+\mathrm{2}+\cancel{\mathrm{2}\sqrt{\mathrm{2}}}+\mathrm{1} \\ $$$$\frac{\mathrm{1}}{{x}^{{a}−{b}} }+\frac{\mathrm{1}}{{x}^{{b}−{a}} }=\mathrm{6} \\ $$
Answered by Rasheed.Sindhi last updated on 18/Mar/22
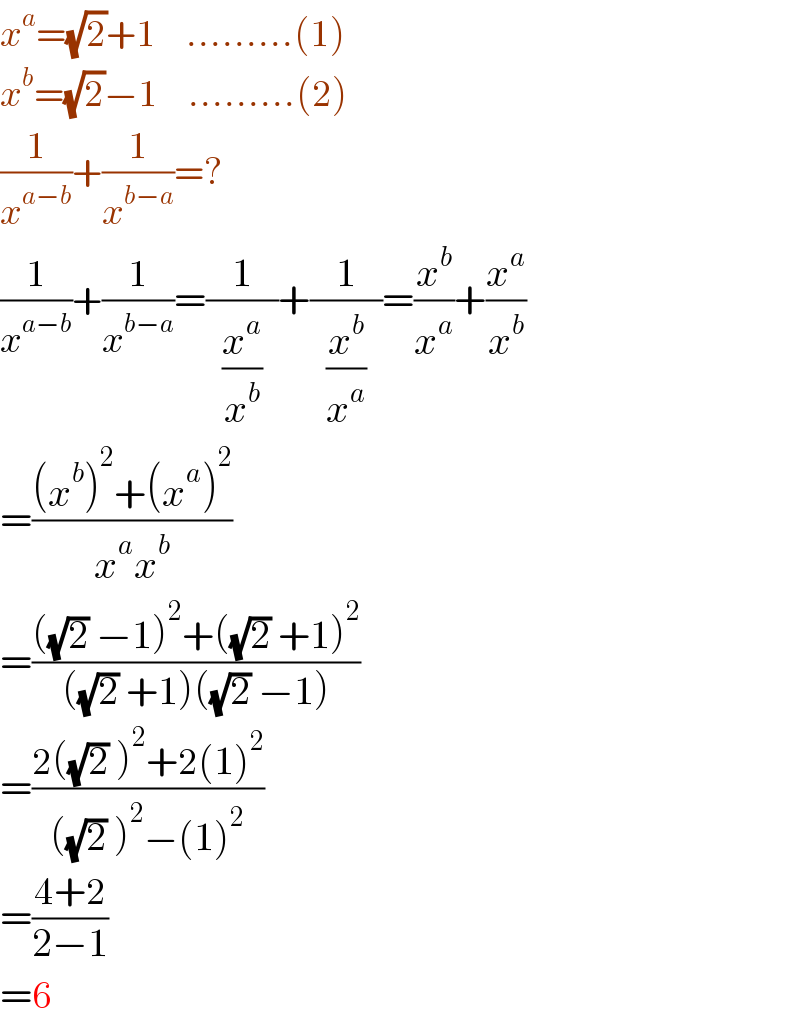
$${x}^{{a}} =\sqrt{\mathrm{2}}+\mathrm{1}\:\:\:\:\:.........\left(\mathrm{1}\right)\: \\ $$$${x}^{{b}} =\sqrt{\mathrm{2}}−\mathrm{1}\:\:\:\:\:.........\left(\mathrm{2}\right) \\ $$$$\frac{\mathrm{1}}{{x}^{{a}−{b}} }+\frac{\mathrm{1}}{{x}^{{b}−{a}} }=? \\ $$$$\frac{\mathrm{1}}{{x}^{{a}−{b}} }+\frac{\mathrm{1}}{{x}^{{b}−{a}} }=\frac{\mathrm{1}}{\:\:\frac{{x}^{{a}} }{{x}^{{b}} }\:\:}+\frac{\mathrm{1}}{\:\:\frac{{x}^{{b}} }{{x}^{{a}} }\:\:}=\frac{{x}^{{b}} }{{x}^{{a}} }+\frac{{x}^{{a}} }{{x}^{{b}} } \\ $$$$=\frac{\left({x}^{{b}} \right)^{\mathrm{2}} +\left({x}^{{a}} \right)^{\mathrm{2}} }{{x}^{{a}} {x}^{{b}} } \\ $$$$=\frac{\left(\sqrt{\mathrm{2}}\:−\mathrm{1}\right)^{\mathrm{2}} +\left(\sqrt{\mathrm{2}}\:+\mathrm{1}\right)^{\mathrm{2}} }{\left(\sqrt{\mathrm{2}}\:+\mathrm{1}\right)\left(\sqrt{\mathrm{2}}\:−\mathrm{1}\right)} \\ $$$$=\frac{\mathrm{2}\left(\sqrt{\mathrm{2}}\:\right)^{\mathrm{2}} +\mathrm{2}\left(\mathrm{1}\right)^{\mathrm{2}} }{\left(\sqrt{\mathrm{2}}\:\right)^{\mathrm{2}} −\left(\mathrm{1}\right)^{\mathrm{2}} } \\ $$$$=\frac{\mathrm{4}+\mathrm{2}}{\mathrm{2}−\mathrm{1}} \\ $$$$=\mathrm{6} \\ $$
