Question and Answers Forum

All Questions      Topic List

Integration Questions

Previous in All Question      Next in All Question      

Previous in Integration      Next in Integration      

Question Number 11982 by tawa last updated on 08/Apr/17

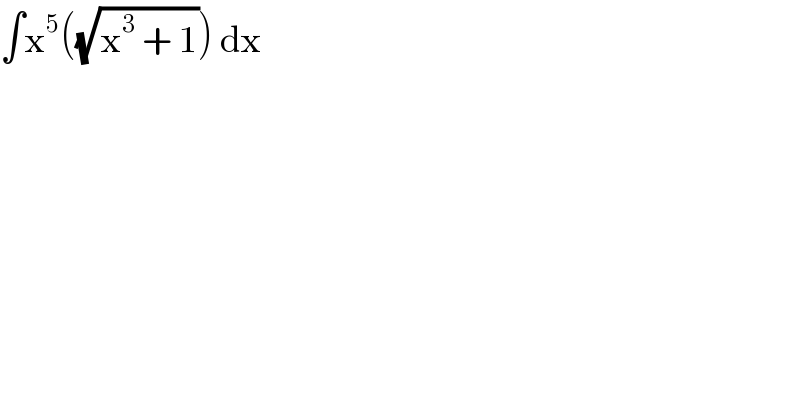
∫x^5 ((√(x^3 + 1))) dx

$$\int\mathrm{x}^{\mathrm{5}} \left(\sqrt{\mathrm{x}^{\mathrm{3}} \:+\:\mathrm{1}}\right)\:\mathrm{dx} \\ $$

Answered by ajfour last updated on 08/Apr/17

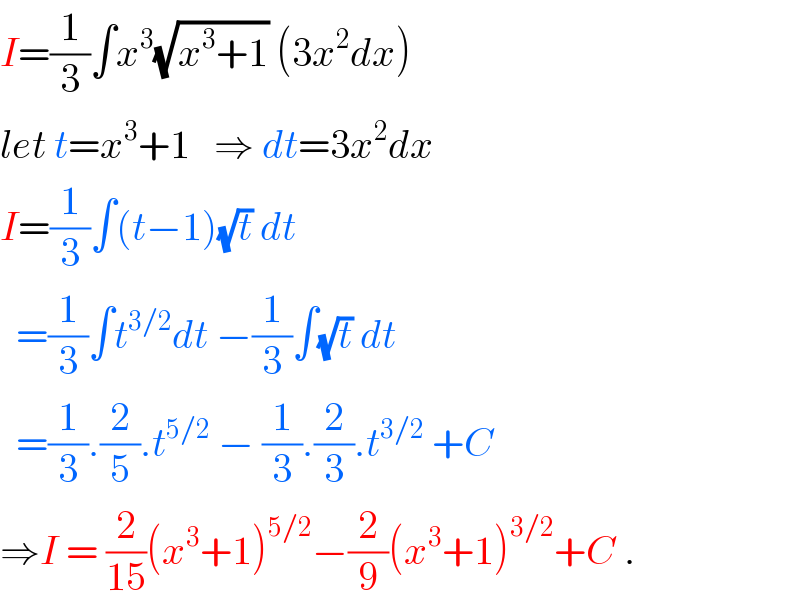
I=(1/3)∫x^3 (√(x^3 +1)) (3x^2 dx) let t=x^3 +1 ⇒ dt=3x^2 dx I=(1/3)∫(t−1)(√t) dt =(1/3)∫t^(3/2) dt −(1/3)∫(√t) dt =(1/3).(2/5).t^(5/2) − (1/3).(2/3).t^(3/2) +C ⇒I = (2/(15))(x^3 +1)^(5/2) −(2/9)(x^3 +1)^(3/2) +C .

$${I}=\frac{\mathrm{1}}{\mathrm{3}}\int{x}^{\mathrm{3}} \sqrt{{x}^{\mathrm{3}} +\mathrm{1}}\:\left(\mathrm{3}{x}^{\mathrm{2}} {dx}\right) \\ $$$${let}\:{t}={x}^{\mathrm{3}} +\mathrm{1}\:\:\:\Rightarrow\:{dt}=\mathrm{3}{x}^{\mathrm{2}} {dx} \\ $$$${I}=\frac{\mathrm{1}}{\mathrm{3}}\int\left({t}−\mathrm{1}\right)\sqrt{{t}}\:{dt} \\ $$$$\:\:=\frac{\mathrm{1}}{\mathrm{3}}\int{t}^{\mathrm{3}/\mathrm{2}} {dt}\:−\frac{\mathrm{1}}{\mathrm{3}}\int\sqrt{{t}}\:{dt} \\ $$$$\:\:=\frac{\mathrm{1}}{\mathrm{3}}.\frac{\mathrm{2}}{\mathrm{5}}.{t}^{\mathrm{5}/\mathrm{2}} \:−\:\frac{\mathrm{1}}{\mathrm{3}}.\frac{\mathrm{2}}{\mathrm{3}}.{t}^{\mathrm{3}/\mathrm{2}} \:+{C} \\ $$$$\Rightarrow{I}\:=\:\frac{\mathrm{2}}{\mathrm{15}}\left({x}^{\mathrm{3}} +\mathrm{1}\right)^{\mathrm{5}/\mathrm{2}} −\frac{\mathrm{2}}{\mathrm{9}}\left({x}^{\mathrm{3}} +\mathrm{1}\right)^{\mathrm{3}/\mathrm{2}} +{C}\:. \\ $$

Commented by tawa last updated on 08/Apr/17

God bless you sir.

$$\mathrm{God}\:\mathrm{bless}\:\mathrm{you}\:\mathrm{sir}. \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com