
Question Number 204056 by hardmath last updated on 05/Feb/24
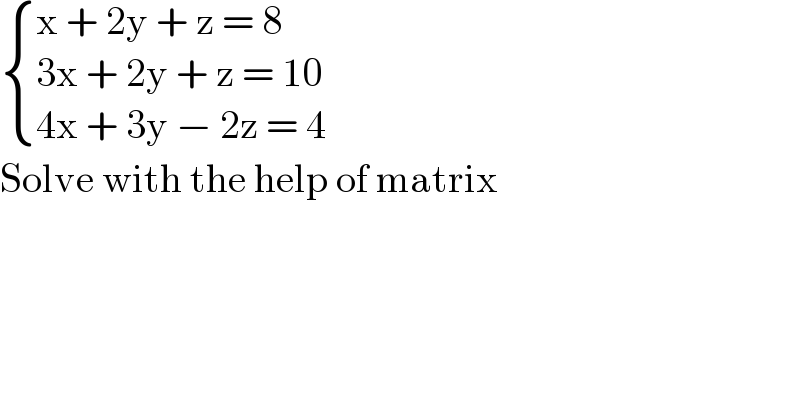
$$\begin{cases}{\mathrm{x}\:+\:\mathrm{2y}\:+\:\mathrm{z}\:=\:\mathrm{8}}\\{\mathrm{3x}\:+\:\mathrm{2y}\:+\:\mathrm{z}\:=\:\mathrm{10}}\\{\mathrm{4x}\:+\:\mathrm{3y}\:−\:\mathrm{2z}\:=\:\mathrm{4}}\end{cases} \\ $$$$\mathrm{Solve}\:\mathrm{with}\:\mathrm{the}\:\mathrm{help}\:\mathrm{of}\:\mathrm{matrix} \\ $$
Answered by deleteduser1 last updated on 05/Feb/24
![[(1,2,1),(3,2,1),(4,3,(−2)) ] [(x),(y),(z) ]= [(8),((10)),(4) ]⇒_(R_3 −4R_1 ) ^(R_2 −3R_1 ) [(1,2,1,8),(0,(−4),(−2),(−14)),(0,(−5),(−6),(−28)) ] ⇒_(4R_3 −5R_2 ) ^(2R_1 +R_2 ) [(2,0,0,2),(0,(−4),(−2),(−14)),(0,0,(−14),(−42)) ]⇒^(−7R_2 +R_3 ) [(2,0,0,∣,2),(0,(28),0,∣,(56)),(0,0,(−14),∣,(−42)) ] ⇒2x+0y+0z=2⇒x=1;0x+28y+0z=56⇒y=2 0x+0y−14z=−42⇒z=3⇒(x,y,z)=(1,2,3).](Q204060.png)
$$\begin{bmatrix}{\mathrm{1}}&{\mathrm{2}}&{\mathrm{1}}\\{\mathrm{3}}&{\mathrm{2}}&{\mathrm{1}}\\{\mathrm{4}}&{\mathrm{3}}&{−\mathrm{2}}\end{bmatrix}\begin{bmatrix}{{x}}\\{{y}}\\{{z}}\end{bmatrix}=\begin{bmatrix}{\mathrm{8}}\\{\mathrm{10}}\\{\mathrm{4}}\end{bmatrix}\underset{{R}_{\mathrm{3}} −\mathrm{4}{R}_{\mathrm{1}} } {\overset{{R}_{\mathrm{2}} −\mathrm{3}{R}_{\mathrm{1}} } {\Rightarrow}}\begin{bmatrix}{\mathrm{1}}&{\mathrm{2}}&{\mathrm{1}}&{\mathrm{8}}\\{\mathrm{0}}&{−\mathrm{4}}&{−\mathrm{2}}&{−\mathrm{14}}\\{\mathrm{0}}&{−\mathrm{5}}&{−\mathrm{6}}&{−\mathrm{28}}\end{bmatrix} \\ $$$$\underset{\mathrm{4}{R}_{\mathrm{3}} −\mathrm{5}{R}_{\mathrm{2}} } {\overset{\mathrm{2}{R}_{\mathrm{1}} +{R}_{\mathrm{2}} } {\Rightarrow}}\begin{bmatrix}{\mathrm{2}}&{\mathrm{0}}&{\mathrm{0}}&{\mathrm{2}}\\{\mathrm{0}}&{−\mathrm{4}}&{−\mathrm{2}}&{−\mathrm{14}}\\{\mathrm{0}}&{\mathrm{0}}&{−\mathrm{14}}&{−\mathrm{42}}\end{bmatrix}\overset{−\mathrm{7}{R}_{\mathrm{2}} +{R}_{\mathrm{3}} } {\Rightarrow}\begin{bmatrix}{\mathrm{2}}&{\mathrm{0}}&{\mathrm{0}}&{\mid}&{\mathrm{2}}\\{\mathrm{0}}&{\mathrm{28}}&{\mathrm{0}}&{\mid}&{\mathrm{56}}\\{\mathrm{0}}&{\mathrm{0}}&{−\mathrm{14}}&{\mid}&{−\mathrm{42}}\end{bmatrix} \\ $$$$\Rightarrow\mathrm{2}{x}+\mathrm{0}{y}+\mathrm{0}{z}=\mathrm{2}\Rightarrow{x}=\mathrm{1};\mathrm{0}{x}+\mathrm{28}{y}+\mathrm{0}{z}=\mathrm{56}\Rightarrow{y}=\mathrm{2} \\ $$$$\mathrm{0}{x}+\mathrm{0}{y}−\mathrm{14}{z}=−\mathrm{42}\Rightarrow{z}=\mathrm{3}\Rightarrow\left({x},{y},{z}\right)=\left(\mathrm{1},\mathrm{2},\mathrm{3}\right). \\ $$
Answered by MikeH last updated on 05/Feb/24
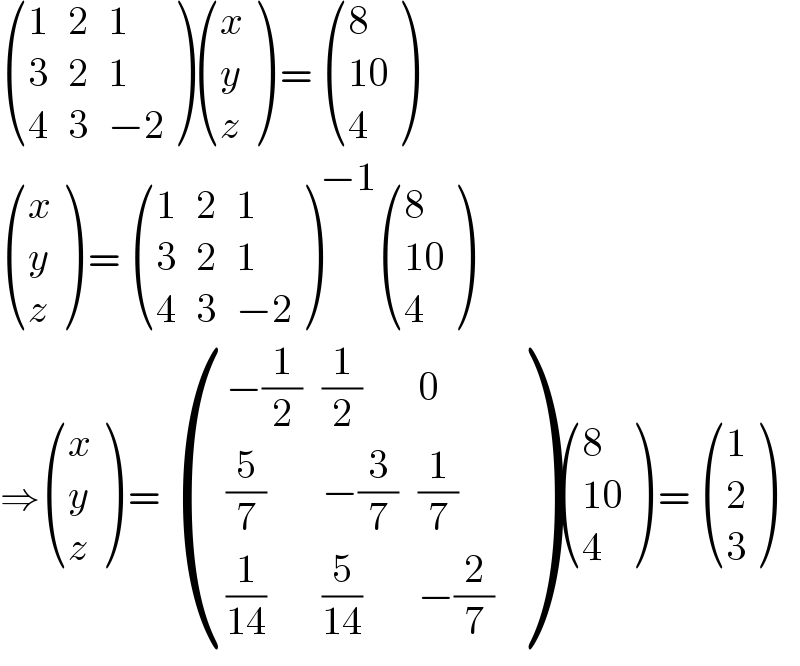
$$\begin{pmatrix}{\mathrm{1}}&{\mathrm{2}}&{\mathrm{1}}\\{\mathrm{3}}&{\mathrm{2}}&{\mathrm{1}}\\{\mathrm{4}}&{\mathrm{3}}&{−\mathrm{2}}\end{pmatrix}\begin{pmatrix}{{x}}\\{{y}}\\{{z}}\end{pmatrix}\:=\:\begin{pmatrix}{\mathrm{8}}\\{\mathrm{10}}\\{\mathrm{4}}\end{pmatrix} \\ $$$$\begin{pmatrix}{{x}}\\{{y}}\\{{z}}\end{pmatrix}\:=\:\begin{pmatrix}{\mathrm{1}}&{\mathrm{2}}&{\mathrm{1}}\\{\mathrm{3}}&{\mathrm{2}}&{\mathrm{1}}\\{\mathrm{4}}&{\mathrm{3}}&{−\mathrm{2}}\end{pmatrix}^{−\mathrm{1}} \begin{pmatrix}{\mathrm{8}}\\{\mathrm{10}}\\{\mathrm{4}}\end{pmatrix}\: \\ $$$$\Rightarrow\begin{pmatrix}{{x}}\\{{y}}\\{{z}}\end{pmatrix}\:=\:\begin{pmatrix}{−\frac{\mathrm{1}}{\mathrm{2}}}&{\frac{\mathrm{1}}{\mathrm{2}}}&{\mathrm{0}}\\{\frac{\mathrm{5}}{\mathrm{7}}}&{−\frac{\mathrm{3}}{\mathrm{7}}}&{\frac{\mathrm{1}}{\mathrm{7}}}\\{\frac{\mathrm{1}}{\mathrm{14}}}&{\frac{\mathrm{5}}{\mathrm{14}}}&{−\frac{\mathrm{2}}{\mathrm{7}}}\end{pmatrix}\begin{pmatrix}{\mathrm{8}}\\{\mathrm{10}}\\{\mathrm{4}}\end{pmatrix}\:=\:\begin{pmatrix}{\mathrm{1}}\\{\mathrm{2}}\\{\mathrm{3}}\end{pmatrix} \\ $$
