
Question Number 166723 by cortano1 last updated on 26/Feb/22
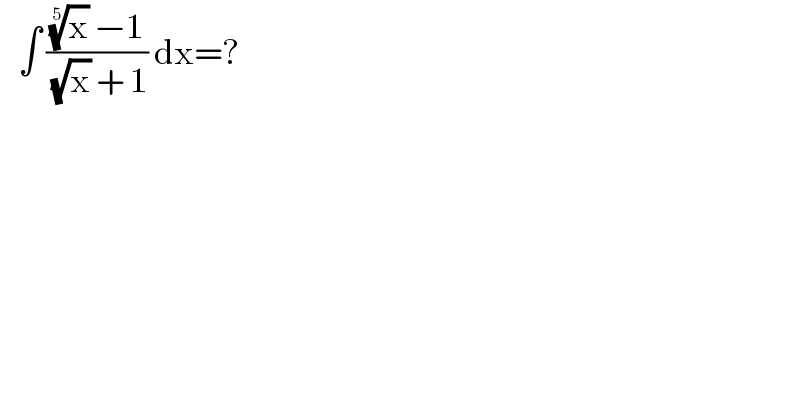
$$\:\:\:\int\:\frac{\sqrt[{\mathrm{5}}]{\mathrm{x}}\:−\mathrm{1}}{\:\sqrt{\mathrm{x}}\:+\:\mathrm{1}}\:\mathrm{dx}=? \\ $$
Answered by MJS_new last updated on 26/Feb/22
![∫((x^(1/5) −1)/(x^(1/2) +1))dx= [t=x^(1/10) → dx=10t^9 dt] =10∫((t^9 (t^2 −1))/(t^5 +1))dt=10∫((t^9 (t−1))/(t^4 −t^3 +t^2 −t+1))dt= =10∫((t^9 (t−1))/((t^2 −((1+(√5))/2)t+1)(t^2 −((1−(√5))/2)t+1)))dt= =I_1 +I_2 +I_3 +I_4 +I_5 with I_1 =10∫(t^6 −t^4 −t)dt=((10)/7)t^7 −2t^5 −5t^2 I_2 =∫(((5−(√5))t−(√5))/(t^2 −((1+(√5))/2)t+1))dt=((5−(√5))/2)ln (t^2 −((1+(√5))/2)t+1) I_3 =∫(((5+(√5))t+(√5))/(t^2 −((1−(√5))/2)t+1))dt=((5+(√5))/2)ln (t^2 −((1−(√5))/2)t+1) I_4 =(√5)∫(dt/(t^2 −((1+(√5))/2)t+1))=(√(2(5+(√5))))arctan (((√(10(5+(√5))))(t−((1+(√5))/4)))/5) I_5 =−(√5)∫(dt/(t^2 −((1−(√5))/2)t+1))=−(√(2(5−(√5))))arctan (((√(10(5−(√5))))(t−((1−(√5))/4)))/5) ...](Q166748.png)
$$\int\frac{{x}^{\mathrm{1}/\mathrm{5}} −\mathrm{1}}{{x}^{\mathrm{1}/\mathrm{2}} +\mathrm{1}}{dx}= \\ $$$$\:\:\:\:\:\left[{t}={x}^{\mathrm{1}/\mathrm{10}} \:\rightarrow\:{dx}=\mathrm{10}{t}^{\mathrm{9}} {dt}\right] \\ $$$$=\mathrm{10}\int\frac{{t}^{\mathrm{9}} \left({t}^{\mathrm{2}} −\mathrm{1}\right)}{{t}^{\mathrm{5}} +\mathrm{1}}{dt}=\mathrm{10}\int\frac{{t}^{\mathrm{9}} \left({t}−\mathrm{1}\right)}{{t}^{\mathrm{4}} −{t}^{\mathrm{3}} +{t}^{\mathrm{2}} −{t}+\mathrm{1}}{dt}= \\ $$$$=\mathrm{10}\int\frac{{t}^{\mathrm{9}} \left({t}−\mathrm{1}\right)}{\left({t}^{\mathrm{2}} −\frac{\mathrm{1}+\sqrt{\mathrm{5}}}{\mathrm{2}}{t}+\mathrm{1}\right)\left({t}^{\mathrm{2}} −\frac{\mathrm{1}−\sqrt{\mathrm{5}}}{\mathrm{2}}{t}+\mathrm{1}\right)}{dt}= \\ $$$$={I}_{\mathrm{1}} +{I}_{\mathrm{2}} +{I}_{\mathrm{3}} +{I}_{\mathrm{4}} +{I}_{\mathrm{5}} \\ $$$$\mathrm{with} \\ $$$${I}_{\mathrm{1}} =\mathrm{10}\int\left({t}^{\mathrm{6}} −{t}^{\mathrm{4}} −{t}\right){dt}=\frac{\mathrm{10}}{\mathrm{7}}{t}^{\mathrm{7}} −\mathrm{2}{t}^{\mathrm{5}} −\mathrm{5}{t}^{\mathrm{2}} \\ $$$${I}_{\mathrm{2}} =\int\frac{\left(\mathrm{5}−\sqrt{\mathrm{5}}\right){t}−\sqrt{\mathrm{5}}}{{t}^{\mathrm{2}} −\frac{\mathrm{1}+\sqrt{\mathrm{5}}}{\mathrm{2}}{t}+\mathrm{1}}{dt}=\frac{\mathrm{5}−\sqrt{\mathrm{5}}}{\mathrm{2}}\mathrm{ln}\:\left({t}^{\mathrm{2}} −\frac{\mathrm{1}+\sqrt{\mathrm{5}}}{\mathrm{2}}{t}+\mathrm{1}\right) \\ $$$${I}_{\mathrm{3}} =\int\frac{\left(\mathrm{5}+\sqrt{\mathrm{5}}\right){t}+\sqrt{\mathrm{5}}}{{t}^{\mathrm{2}} −\frac{\mathrm{1}−\sqrt{\mathrm{5}}}{\mathrm{2}}{t}+\mathrm{1}}{dt}=\frac{\mathrm{5}+\sqrt{\mathrm{5}}}{\mathrm{2}}\mathrm{ln}\:\left({t}^{\mathrm{2}} −\frac{\mathrm{1}−\sqrt{\mathrm{5}}}{\mathrm{2}}{t}+\mathrm{1}\right) \\ $$$${I}_{\mathrm{4}} =\sqrt{\mathrm{5}}\int\frac{{dt}}{{t}^{\mathrm{2}} −\frac{\mathrm{1}+\sqrt{\mathrm{5}}}{\mathrm{2}}{t}+\mathrm{1}}=\sqrt{\mathrm{2}\left(\mathrm{5}+\sqrt{\mathrm{5}}\right)}\mathrm{arctan}\:\frac{\sqrt{\mathrm{10}\left(\mathrm{5}+\sqrt{\mathrm{5}}\right)}\left({t}−\frac{\mathrm{1}+\sqrt{\mathrm{5}}}{\mathrm{4}}\right)}{\mathrm{5}} \\ $$$${I}_{\mathrm{5}} =−\sqrt{\mathrm{5}}\int\frac{{dt}}{{t}^{\mathrm{2}} −\frac{\mathrm{1}−\sqrt{\mathrm{5}}}{\mathrm{2}}{t}+\mathrm{1}}=−\sqrt{\mathrm{2}\left(\mathrm{5}−\sqrt{\mathrm{5}}\right)}\mathrm{arctan}\:\frac{\sqrt{\mathrm{10}\left(\mathrm{5}−\sqrt{\mathrm{5}}\right)}\left({t}−\frac{\mathrm{1}−\sqrt{\mathrm{5}}}{\mathrm{4}}\right)}{\mathrm{5}} \\ $$$$... \\ $$
