
Question Number 34044 by NECx last updated on 29/Apr/18
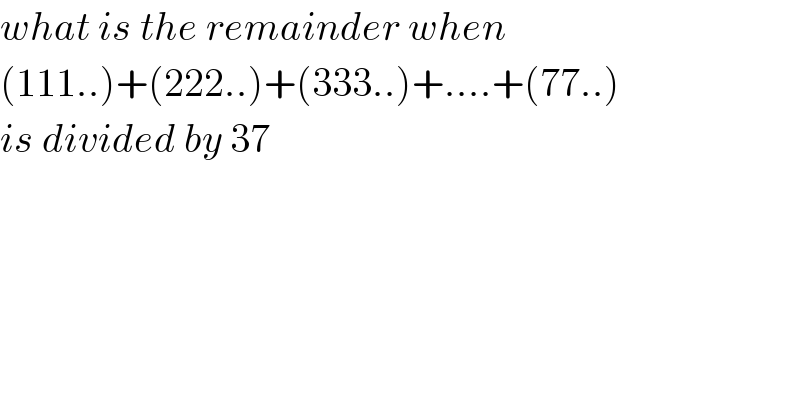
$${what}\:{is}\:{the}\:{remainder}\:{when}\: \\ $$$$\left(\mathrm{111}..\right)+\left(\mathrm{222}..\right)+\left(\mathrm{333}..\right)+....+\left(\mathrm{77}..\right) \\ $$$${is}\:{divided}\:{by}\:\mathrm{37} \\ $$
Commented by Rasheed.Sindhi last updated on 30/Apr/18

$$\mathrm{N}=\left(\mathrm{111}..\right)+\left(\mathrm{222}..\right)+\left(\mathrm{333}..\right)+....+\left(\mathrm{77}..\right) \\ $$$$ \\ $$$$=\mathrm{1}\left(\mathrm{111}...\right)+\mathrm{2}\left(\mathrm{111}..\right)+\mathrm{3}\left(\mathrm{111}...\right)...+\mathrm{7}\left(\mathrm{111}..\right) \\ $$$$=\left(\mathrm{1}+\mathrm{2}+\mathrm{3}+...+\mathrm{7}\right)\left(\mathrm{11}...\right) \\ $$$$=\mathrm{28}×\mathrm{111}... \\ $$$$\mathrm{Let}\:\mathrm{111}...\mathrm{is}\:\mathrm{of}\:\mathrm{n}-\mathrm{digits}\:\mathrm{number}. \\ $$$$\mathrm{For}\:\mathrm{n}=\mathrm{1} \\ $$$$\mathrm{N}=\mathrm{28}.\mathrm{1}=\mathrm{28},\: \\ $$$$\mathrm{If}\:\mathrm{28}\:\mathrm{is}\:\mathrm{divided}\:\mathrm{by}\:\mathrm{37}\:\mathrm{the}\:\mathrm{remainder}\:\mathrm{is}\:\mathrm{28} \\ $$$$....... \\ $$$$.... \\ $$$$\mathrm{If}\:\mathrm{n}=\mathrm{3k},\mathrm{the}\:\mathrm{remainder}\:\mathrm{is}\:\:\mathrm{0} \\ $$$$\mathrm{If}\:\mathrm{n}=\mathrm{3k}+\mathrm{1},\mathrm{the}\:\mathrm{remainder}\:\mathrm{is}\:\mathrm{28} \\ $$$$\mathrm{If}\:\mathrm{n}=\mathrm{3k}+\mathrm{2},\mathrm{the}\:\mathrm{remainder}\:\mathrm{is}\:\mathrm{12} \\ $$$$\mathrm{The}\:\mathrm{remainders}\::\:\mathrm{0},\mathrm{28},\mathrm{12} \\ $$
Commented by NECx last updated on 30/Apr/18
$${thank}\:{you}\:{so}\:{much}\:{buddy} \\ $$
Answered by tanmay.chaudhury50@gmail.com last updated on 30/Apr/18

$$\left(\mathrm{111}...\right)+\left(\mathrm{222}...\right)+\left(\mathrm{333}...\right)+....+\left(\mathrm{777}...\right) \\ $$$$\left(\mathrm{111}...\right)+\mathrm{2}\left(\mathrm{111}...\right)+\mathrm{3}\left(\mathrm{111}...\right)+...+\mathrm{7}\left(\mathrm{111}...\right) \\ $$$${let}\:{value}\:{of}\:\left(\mathrm{111}...\right)\:{say}={t} \\ $$$${t}\left(\mathrm{1}+\mathrm{2}+\mathrm{3}+...+\mathrm{7}\right) \\ $$$${t}\left(\mathrm{28}\right) \\ $$$${now}\:{let}\:{us}\:{find}\:{the}\:{value}\:{of}\:\left(\mathrm{111}...{upto}\:{n}\:\right)\:{then} \\ $$$${put}\:{n}\rightarrow\infty \\ $$$$\left(\mathrm{111}...{upto}\:{n}\:{terms}\right) \\ $$$$\mathrm{1}+\mathrm{10}+\mathrm{10}^{\mathrm{2}} +\mathrm{10}^{\mathrm{3}} +.... \\ $$$$\frac{\left(\mathrm{10}^{{n}} −\mathrm{1}\right)}{\mathrm{10}−\mathrm{1}} \\ $$$$\mathrm{28}{t} \\ $$$$\left(\mathrm{37}−\mathrm{9}\right){t} \\ $$$$\left(\mathrm{37}−\mathrm{9}\right)\frac{\left(\mathrm{10}^{{n}} −\mathrm{1}\right)}{\mathrm{9}} \\ $$$$\frac{\mathrm{37}}{\mathrm{9}}\left(\mathrm{10}^{{n}} −\mathrm{1}\right)+\left(\mathrm{1}−\mathrm{10}^{{n}} \right) \\ $$$${when}\:{devided}\:{by}\:\mathrm{37}\:{first}\:{part}\:{is}\:{divisble}\:{by}\:\mathrm{37} \\ $$$${remaining}\:{is}\:{remainder}\:{under}\:{the}\:{condktion}\:{n}\rightarrow\infty \\ $$$${pls}\:{recheck}\:{the}\:{question}\:{pls} \\ $$$$ \\ $$
Commented by NECx last updated on 30/Apr/18
$${wow}....\:{lemme}\:{check}\:{it}..{Really} \\ $$$${I}\:{love}\:{what}\:{you}\:{just}\:{did}.{Thanks} \\ $$
Commented by Rasheed.Sindhi last updated on 30/Apr/18
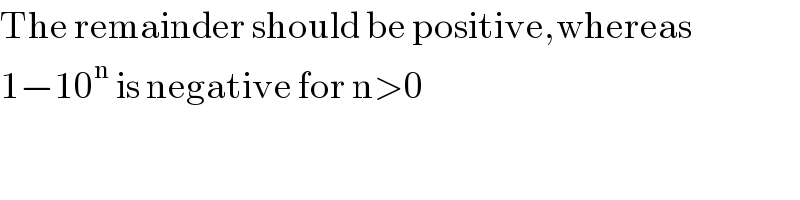
$$\mathrm{The}\:\mathrm{remainder}\:\mathrm{should}\:\mathrm{be}\:\mathrm{positive},\mathrm{whereas} \\ $$$$\mathrm{1}−\mathrm{10}^{\mathrm{n}} \:\mathrm{is}\:\mathrm{negative}\:\mathrm{for}\:\mathrm{n}>\mathrm{0} \\ $$
Answered by 5a3k last updated on 30/Apr/18
![[(111..)+(222..)+(333..)+...+(777..)] ((37[3+6+9+...+21])/(37)) 3+6+9+....+21 7/2[2×3+(7−1)3] 84](Q34077.png)
$$\left[\left(\mathrm{111}..\right)+\left(\mathrm{222}..\right)+\left(\mathrm{333}..\right)+...+\left(\mathrm{777}..\right)\right] \\ $$$$\frac{\mathrm{37}\left[\mathrm{3}+\mathrm{6}+\mathrm{9}+...+\mathrm{21}\right]}{\mathrm{37}} \\ $$$$\mathrm{3}+\mathrm{6}+\mathrm{9}+....+\mathrm{21} \\ $$$$\mathrm{7}/\mathrm{2}\left[\mathrm{2}×\mathrm{3}+\left(\mathrm{7}−\mathrm{1}\right)\mathrm{3}\right] \\ $$$$\mathrm{84} \\ $$
Commented by NECx last updated on 30/Apr/18

$${not}\:{clear}\:{please} \\ $$
