Question and Answers Forum

All Questions      Topic List

Algebra Questions

Previous in All Question      Next in All Question      

Previous in Algebra      Next in Algebra      

Question Number 125669 by mathocean1 last updated on 12/Dec/20

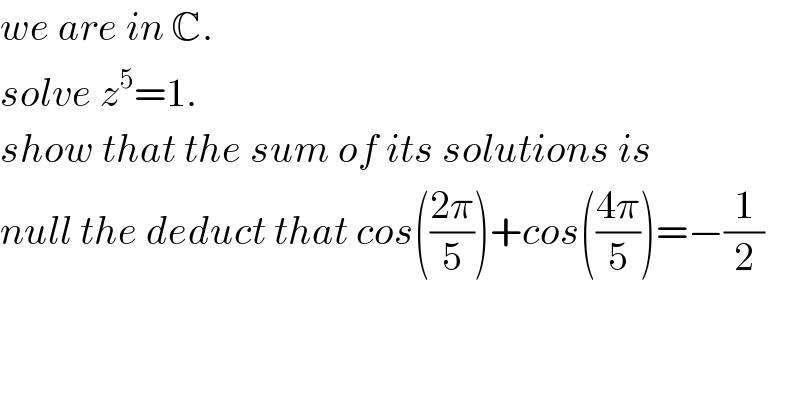
we are in C. solve z^5 =1. show that the sum of its solutions is null the deduct that cos(((2π)/5))+cos(((4π)/5))=−(1/2)

$${we}\:{are}\:{in}\:\mathbb{C}. \\ $$$${solve}\:{z}^{\mathrm{5}} =\mathrm{1}. \\ $$$${show}\:{that}\:{the}\:{sum}\:{of}\:{its}\:{solutions}\:{is} \\ $$$${null}\:{the}\:{deduct}\:{that}\:{cos}\left(\frac{\mathrm{2}\pi}{\mathrm{5}}\right)+{cos}\left(\frac{\mathrm{4}\pi}{\mathrm{5}}\right)=−\frac{\mathrm{1}}{\mathrm{2}} \\ $$

Answered by mr W last updated on 13/Dec/20

z^5 +0z^4 −1=0 Σ_(k=0) ^4 z_k =0. z_k =cos ((2kπ)/5)+i sin ((2kπ)/5) Σz_k =(1+cos ((2π)/5)+cos ((4π)/5)+cos ((6π)/5)+cos ((8π)/5))+i (9+sin ((2π)/5)+sin ((4π)/5)+sin ((6π)/5)+sin ((8π)/5))=0 1+cos ((2π)/5)+cos ((4π)/5)+cos ((6π)/5)+cos ((8π)/5)=0 1+cos ((2π)/5)+cos ((4π)/5)+cos (2π−((4π)/5))+cos (2π−((2π)/5))=0 1+cos ((2π)/5)+cos ((4π)/5)+cos ((4π)/5)+cos ((2π)/5)=0 1+2(cos ((2π)/5)+cos ((4π)/5))=0 ⇒cos ((2π)/5)+cos ((4π)/5)=−(1/2)

$${z}^{\mathrm{5}} +\mathrm{0}{z}^{\mathrm{4}} −\mathrm{1}=\mathrm{0} \\ $$$$\underset{{k}=\mathrm{0}} {\overset{\mathrm{4}} {\sum}}{z}_{{k}} =\mathrm{0}. \\ $$$${z}_{{k}} =\mathrm{cos}\:\frac{\mathrm{2}{k}\pi}{\mathrm{5}}+{i}\:\mathrm{sin}\:\frac{\mathrm{2}{k}\pi}{\mathrm{5}} \\ $$$$\Sigma{z}_{{k}} =\left(\mathrm{1}+\mathrm{cos}\:\frac{\mathrm{2}\pi}{\mathrm{5}}+\mathrm{cos}\:\frac{\mathrm{4}\pi}{\mathrm{5}}+\mathrm{cos}\:\frac{\mathrm{6}\pi}{\mathrm{5}}+\mathrm{cos}\:\frac{\mathrm{8}\pi}{\mathrm{5}}\right)+{i}\:\left(\mathrm{9}+\mathrm{sin}\:\frac{\mathrm{2}\pi}{\mathrm{5}}+\mathrm{sin}\:\frac{\mathrm{4}\pi}{\mathrm{5}}+\mathrm{sin}\:\frac{\mathrm{6}\pi}{\mathrm{5}}+\mathrm{sin}\:\frac{\mathrm{8}\pi}{\mathrm{5}}\right)=\mathrm{0} \\ $$$$\mathrm{1}+\mathrm{cos}\:\frac{\mathrm{2}\pi}{\mathrm{5}}+\mathrm{cos}\:\frac{\mathrm{4}\pi}{\mathrm{5}}+\mathrm{cos}\:\frac{\mathrm{6}\pi}{\mathrm{5}}+\mathrm{cos}\:\frac{\mathrm{8}\pi}{\mathrm{5}}=\mathrm{0} \\ $$$$\mathrm{1}+\mathrm{cos}\:\frac{\mathrm{2}\pi}{\mathrm{5}}+\mathrm{cos}\:\frac{\mathrm{4}\pi}{\mathrm{5}}+\mathrm{cos}\:\left(\mathrm{2}\pi−\frac{\mathrm{4}\pi}{\mathrm{5}}\right)+\mathrm{cos}\:\left(\mathrm{2}\pi−\frac{\mathrm{2}\pi}{\mathrm{5}}\right)=\mathrm{0} \\ $$$$\mathrm{1}+\mathrm{cos}\:\frac{\mathrm{2}\pi}{\mathrm{5}}+\mathrm{cos}\:\frac{\mathrm{4}\pi}{\mathrm{5}}+\mathrm{cos}\:\frac{\mathrm{4}\pi}{\mathrm{5}}+\mathrm{cos}\:\frac{\mathrm{2}\pi}{\mathrm{5}}=\mathrm{0} \\ $$$$\mathrm{1}+\mathrm{2}\left(\mathrm{cos}\:\frac{\mathrm{2}\pi}{\mathrm{5}}+\mathrm{cos}\:\frac{\mathrm{4}\pi}{\mathrm{5}}\right)=\mathrm{0} \\ $$$$\Rightarrow\mathrm{cos}\:\frac{\mathrm{2}\pi}{\mathrm{5}}+\mathrm{cos}\:\frac{\mathrm{4}\pi}{\mathrm{5}}=−\frac{\mathrm{1}}{\mathrm{2}} \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com