
Question Number 116976 by bobhans last updated on 08/Oct/20
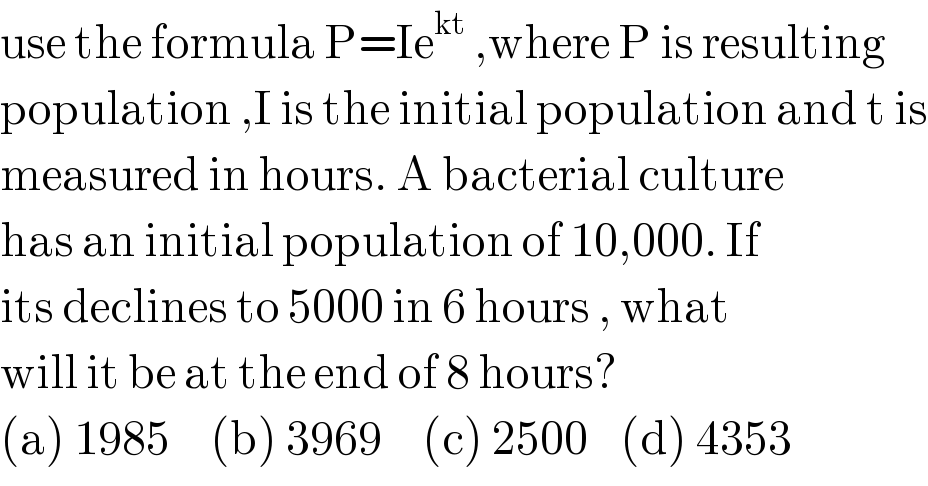
$$\mathrm{use}\:\mathrm{the}\:\mathrm{formula}\:\mathrm{P}=\mathrm{Ie}^{\mathrm{kt}} \:,\mathrm{where}\:\mathrm{P}\:\mathrm{is}\:\mathrm{resulting} \\ $$$$\mathrm{population}\:,\mathrm{I}\:\mathrm{is}\:\mathrm{the}\:\mathrm{initial}\:\mathrm{population}\:\mathrm{and}\:\mathrm{t}\:\mathrm{is} \\ $$$$\mathrm{measured}\:\mathrm{in}\:\mathrm{hours}.\:\mathrm{A}\:\mathrm{bacterial}\:\mathrm{culture} \\ $$$$\mathrm{has}\:\mathrm{an}\:\mathrm{initial}\:\mathrm{population}\:\mathrm{of}\:\mathrm{10},\mathrm{000}.\:\mathrm{If} \\ $$$$\mathrm{its}\:\mathrm{declines}\:\mathrm{to}\:\mathrm{5000}\:\mathrm{in}\:\mathrm{6}\:\mathrm{hours}\:,\:\mathrm{what}\: \\ $$$$\mathrm{will}\:\mathrm{it}\:\mathrm{be}\:\mathrm{at}\:\mathrm{the}\:\mathrm{end}\:\mathrm{of}\:\mathrm{8}\:\mathrm{hours}? \\ $$$$\left(\mathrm{a}\right)\:\mathrm{1985}\:\:\:\:\:\left(\mathrm{b}\right)\:\mathrm{3969}\:\:\:\:\:\left(\mathrm{c}\right)\:\mathrm{2500}\:\:\:\:\left(\mathrm{d}\right)\:\mathrm{4353} \\ $$
Answered by bobhans last updated on 08/Oct/20
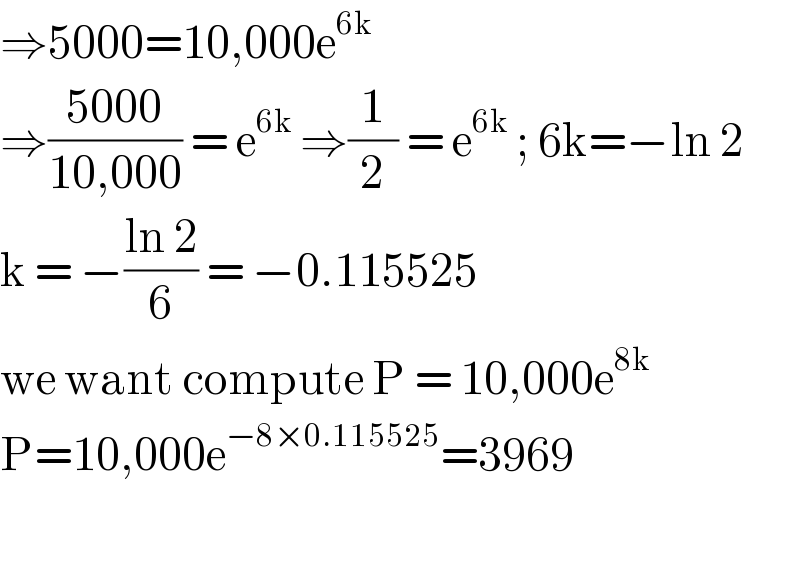
$$\Rightarrow\mathrm{5000}=\mathrm{10},\mathrm{000e}^{\mathrm{6k}} \\ $$$$\Rightarrow\frac{\mathrm{5000}}{\mathrm{10},\mathrm{000}}\:=\:\mathrm{e}^{\mathrm{6k}} \:\Rightarrow\frac{\mathrm{1}}{\mathrm{2}}\:=\:\mathrm{e}^{\mathrm{6k}} \:;\:\mathrm{6k}=−\mathrm{ln}\:\mathrm{2} \\ $$$$\mathrm{k}\:=\:−\frac{\mathrm{ln}\:\mathrm{2}}{\mathrm{6}}\:=\:−\mathrm{0}.\mathrm{115525} \\ $$$$\mathrm{we}\:\mathrm{want}\:\mathrm{compute}\:\mathrm{P}\:=\:\mathrm{10},\mathrm{000e}^{\mathrm{8k}} \\ $$$$\mathrm{P}=\mathrm{10},\mathrm{000e}^{−\mathrm{8}×\mathrm{0}.\mathrm{115525}} =\mathrm{3969} \\ $$$$ \\ $$
Answered by Rio Michael last updated on 08/Oct/20
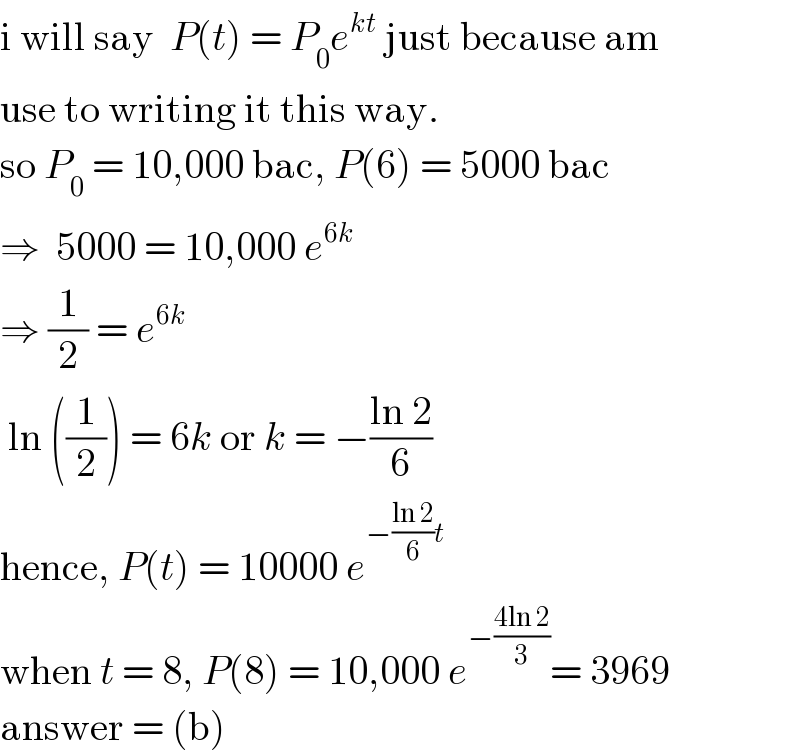
$$\mathrm{i}\:\mathrm{will}\:\mathrm{say}\:\:{P}\left({t}\right)\:=\:{P}_{\mathrm{0}} {e}^{{kt}} \:\mathrm{just}\:\mathrm{because}\:\mathrm{am} \\ $$$$\mathrm{use}\:\mathrm{to}\:\mathrm{writing}\:\mathrm{it}\:\mathrm{this}\:\mathrm{way}. \\ $$$$\mathrm{so}\:{P}_{\mathrm{0}} \:=\:\mathrm{10},\mathrm{000}\:\mathrm{bac},\:{P}\left(\mathrm{6}\right)\:=\:\mathrm{5000}\:\mathrm{bac} \\ $$$$\Rightarrow\:\:\mathrm{5000}\:=\:\mathrm{10},\mathrm{000}\:{e}^{\mathrm{6}{k}} \\ $$$$\Rightarrow\:\frac{\mathrm{1}}{\mathrm{2}}\:=\:{e}^{\mathrm{6}{k}} \\ $$$$\:\mathrm{ln}\:\left(\frac{\mathrm{1}}{\mathrm{2}}\right)\:=\:\mathrm{6}{k}\:\mathrm{or}\:{k}\:=\:−\frac{\mathrm{ln}\:\mathrm{2}}{\mathrm{6}} \\ $$$$\mathrm{hence},\:{P}\left({t}\right)\:=\:\mathrm{10000}\:{e}^{−\frac{\mathrm{ln}\:\mathrm{2}}{\mathrm{6}}{t}} \\ $$$$\mathrm{when}\:{t}\:=\:\mathrm{8},\:{P}\left(\mathrm{8}\right)\:=\:\mathrm{10},\mathrm{000}\:{e}^{−\frac{\mathrm{4ln}\:\mathrm{2}}{\mathrm{3}}} =\:\mathrm{3969} \\ $$$$\mathrm{answer}\:=\:\left(\mathrm{b}\right) \\ $$
