Question and Answers Forum

All Questions      Topic List

Others Questions

Previous in All Question      Next in All Question      

Previous in Others      Next in Others      

Question Number 168399 by Mastermind last updated on 09/Apr/22

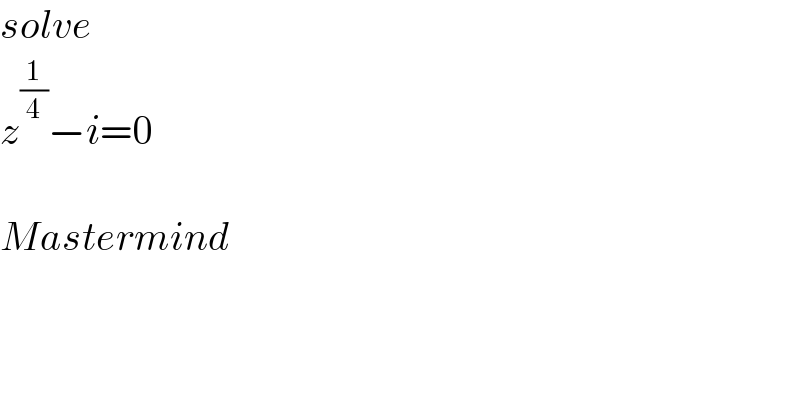
solve z^(1/4) −i=0 Mastermind

$${solve} \\ $$$${z}^{\frac{\mathrm{1}}{\mathrm{4}}} −{i}=\mathrm{0} \\ $$$$ \\ $$$${Mastermind} \\ $$

Answered by nurtani last updated on 10/Apr/22

i=(√(−1))⇒ i^2 =−1 z^(1/4) −i=0 ⇒ z^(1/4) =i ⇒ z=i^4 ⇒ z = (i^2 )^2 ⇒ z = (−1)^2 = 1

$${i}=\sqrt{−\mathrm{1}}\Rightarrow\:{i}^{\mathrm{2}} =−\mathrm{1} \\ $$$${z}^{\frac{\mathrm{1}}{\mathrm{4}}} −{i}=\mathrm{0}\:\Rightarrow\:{z}^{\frac{\mathrm{1}}{\mathrm{4}}} ={i} \\ $$$$\Rightarrow\:{z}={i}^{\mathrm{4}} \:\Rightarrow\:{z}\:=\:\left({i}^{\mathrm{2}} \right)^{\mathrm{2}} \:\Rightarrow\:{z}\:=\:\left(−\mathrm{1}\right)^{\mathrm{2}} \:=\:\mathrm{1} \\ $$

Commented by Mastermind last updated on 10/Apr/22

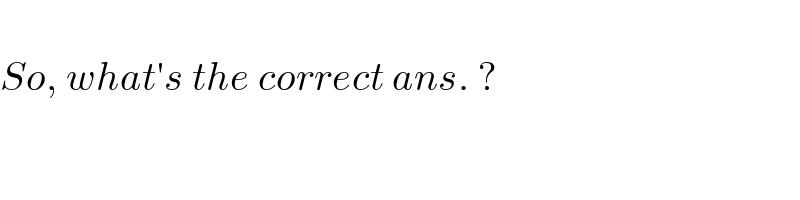
So, what′s the correct ans. ?

$$ \\ $$$${So},\:{what}'{s}\:{the}\:{correct}\:{ans}.\:? \\ $$

Commented by MJS_new last updated on 10/Apr/22

the correct answer is: it has no solution

$$\mathrm{the}\:\mathrm{correct}\:\mathrm{answer}\:\mathrm{is}:\:\mathrm{it}\:\mathrm{has}\:\mathrm{no}\:\mathrm{solution} \\ $$

Commented by MJS_new last updated on 10/Apr/22

but 1^(1/4) =1≠i exponentiating an equation might introduce false solutions z^(1/4) =i has no solution. similar z^(1/2) =−1 has no solution.

$$\mathrm{but}\:\mathrm{1}^{\mathrm{1}/\mathrm{4}} =\mathrm{1}\neq\mathrm{i} \\ $$$$\mathrm{exponentiating}\:\mathrm{an}\:\mathrm{equation}\:\mathrm{might}\:\mathrm{introduce} \\ $$$$\mathrm{false}\:\mathrm{solutions} \\ $$$${z}^{\mathrm{1}/\mathrm{4}} =\mathrm{i}\:\mathrm{has}\:\mathrm{no}\:\mathrm{solution}. \\ $$$$\mathrm{similar}\:{z}^{\mathrm{1}/\mathrm{2}} =−\mathrm{1}\:\mathrm{has}\:\mathrm{no}\:\mathrm{solution}. \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com