Question and Answers Forum

All Questions      Topic List

Integration Questions

Previous in All Question      Next in All Question      

Previous in Integration      Next in Integration      

Question Number 33759 by 33 last updated on 24/Apr/18

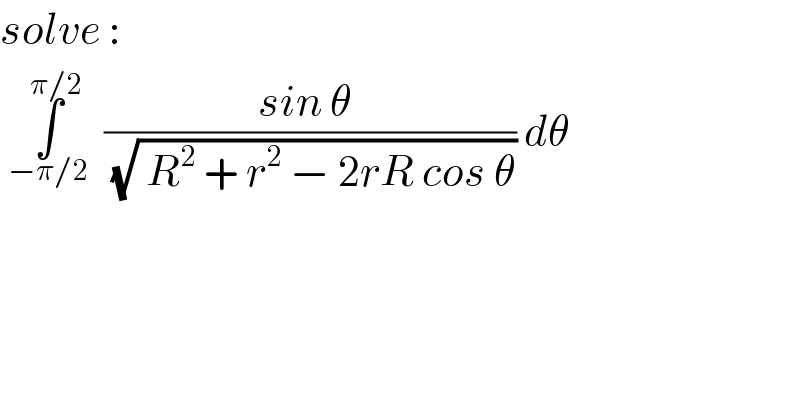
solve : ∫_(−π/2) ^(π/2) ((sin θ )/(√( R^2 + r^2 − 2rR cos θ))) dθ

$${solve}\::\: \\ $$$$\:\underset{−\pi/\mathrm{2}} {\overset{\pi/\mathrm{2}} {\int}}\:\:\frac{{sin}\:\theta\:}{\sqrt{\:{R}^{\mathrm{2}} \:+\:{r}^{\mathrm{2}} \:−\:\mathrm{2}{rR}\:{cos}\:\theta}}\:{d}\theta \\ $$

Commented by MJS last updated on 24/Apr/18

∫((cos θ )/(√( R^2 + r^2 − 2rRcos θ)))dθ= R^2 +r^2 =p −2rR=q =∫((cos θ)/(√(p+qcos θ)))dθ= thanks to my friend Prof. H.E. cos θ=(1/q)(p+qcos θ)−(p/q) =∫(((1/q)(p+qcos θ)−(p/q))/(√(p+qcos θ)))dθ= =(1/q)∫((p+qcos θ)/(√(p+qcos θ)))dθ−(p/q)∫(1/(√(p+qcos θ)))dθ= =(1/q)∫(√(p+qcos θ))dθ−(p/q)∫(1/(√(p+qcos θ)))dθ= sin^2 (θ/2)=((1−cos θ)/2) cos θ=1−2sin^2 (θ/2) p+qcos θ=p+q−2qsin^2 (θ/2)= =(p+q)(1−((2q)/(p+q))sin^2 (θ/2)) =((√(p+q))/q)∫(√((1−((2q)/(p+q))sin^2 (θ/2))))dθ−((p(√(p+q)))/(q(p+q)))∫(1/(√((1−((2q)/(p+q))sin^2 (θ/2)))))dθ u=(x/2) → dx=2du =((2(√(p+q)))/q)∫(√((1−((2q)/(p+q))sin^2 u)))du−((2p(√(p+q)))/(q(p+q)))∫(1/(√((1−((2q)/(p+q))sin^2 u))))du= the first is an elliptic integral of the 2^(nd) kind, the second one of the 1^(st) kind the notation follows, but it′s beyond my understanding... =((2(√(p+q)))/q)E(u∣((2q)/(p+q)))−((2p(√(p+q)))/(q(p+q)))F(u∣((2q)/(p+q)))= u=(x/2) =((2(√(p+q)))/q)(E((x/2)∣((2q)/(p+q)))−(p/(p+q))F((x/2)∣((2q)/(p+q))))

$$\int\frac{\mathrm{cos}\:\theta\:}{\sqrt{\:{R}^{\mathrm{2}} \:+\:{r}^{\mathrm{2}} \:−\:\mathrm{2}{rR}\mathrm{cos}\:\theta}}{d}\theta= \\ $$$$\:\:\:\:\:\:\:\:\:\:{R}^{\mathrm{2}} +{r}^{\mathrm{2}} ={p} \\ $$$$\:\:\:\:\:\:\:\:\:\:−\mathrm{2}{rR}={q} \\ $$$$=\int\frac{\mathrm{cos}\:\theta}{\sqrt{{p}+{q}\mathrm{cos}\:\theta}}{d}\theta= \\ $$$$\:\:\:\:\:\:\:\:\:\:\mathrm{thanks}\:\mathrm{to}\:\mathrm{my}\:\mathrm{friend}\:\mathrm{Prof}.\:\mathrm{H}.\mathrm{E}. \\ $$$$\:\:\:\:\:\:\:\:\:\:\mathrm{cos}\:\theta=\frac{\mathrm{1}}{{q}}\left({p}+{q}\mathrm{cos}\:\theta\right)−\frac{{p}}{{q}} \\ $$$$=\int\frac{\frac{\mathrm{1}}{{q}}\left({p}+{q}\mathrm{cos}\:\theta\right)−\frac{{p}}{{q}}}{\sqrt{{p}+{q}\mathrm{cos}\:\theta}}{d}\theta= \\ $$$$=\frac{\mathrm{1}}{{q}}\int\frac{{p}+{q}\mathrm{cos}\:\theta}{\sqrt{{p}+{q}\mathrm{cos}\:\theta}}{d}\theta−\frac{{p}}{{q}}\int\frac{\mathrm{1}}{\sqrt{{p}+{q}\mathrm{cos}\:\theta}}{d}\theta= \\ $$$$=\frac{\mathrm{1}}{{q}}\int\sqrt{{p}+{q}\mathrm{cos}\:\theta}{d}\theta−\frac{{p}}{{q}}\int\frac{\mathrm{1}}{\sqrt{{p}+{q}\mathrm{cos}\:\theta}}{d}\theta= \\ $$$$\:\:\:\:\:\:\:\:\:\:\mathrm{sin}^{\mathrm{2}} \:\frac{\theta}{\mathrm{2}}=\frac{\mathrm{1}−\mathrm{cos}\:\theta}{\mathrm{2}} \\ $$$$\:\:\:\:\:\:\:\:\:\:\mathrm{cos}\:\theta=\mathrm{1}−\mathrm{2sin}^{\mathrm{2}} \:\frac{\theta}{\mathrm{2}} \\ $$$$\:\:\:\:\:\:\:\:\:\:{p}+{q}\mathrm{cos}\:\theta={p}+{q}−\mathrm{2}{q}\mathrm{sin}^{\mathrm{2}} \:\frac{\theta}{\mathrm{2}}= \\ $$$$\:\:\:\:\:\:\:\:\:\:=\left({p}+{q}\right)\left(\mathrm{1}−\frac{\mathrm{2}{q}}{{p}+{q}}\mathrm{sin}^{\mathrm{2}} \:\frac{\theta}{\mathrm{2}}\right) \\ $$$$=\frac{\sqrt{{p}+{q}}}{{q}}\int\sqrt{\left(\mathrm{1}−\frac{\mathrm{2}{q}}{{p}+{q}}\mathrm{sin}^{\mathrm{2}} \:\frac{\theta}{\mathrm{2}}\right)}{d}\theta−\frac{{p}\sqrt{{p}+{q}}}{{q}\left({p}+{q}\right)}\int\frac{\mathrm{1}}{\sqrt{\left(\mathrm{1}−\frac{\mathrm{2}{q}}{{p}+{q}}\mathrm{sin}^{\mathrm{2}} \:\frac{\theta}{\mathrm{2}}\right)}}{d}\theta \\ $$$$\:\:\:\:\:\:\:\:\:\:{u}=\frac{{x}}{\mathrm{2}}\:\rightarrow\:{dx}=\mathrm{2}{du} \\ $$$$=\frac{\mathrm{2}\sqrt{{p}+{q}}}{{q}}\int\sqrt{\left(\mathrm{1}−\frac{\mathrm{2}{q}}{{p}+{q}}\mathrm{sin}^{\mathrm{2}} \:{u}\right)}{du}−\frac{\mathrm{2}{p}\sqrt{{p}+{q}}}{{q}\left({p}+{q}\right)}\int\frac{\mathrm{1}}{\sqrt{\left(\mathrm{1}−\frac{\mathrm{2}{q}}{{p}+{q}}\mathrm{sin}^{\mathrm{2}} \:{u}\right)}}{du}= \\ $$$$\:\:\:\:\:\:\:\:\:\:\mathrm{the}\:\mathrm{first}\:\mathrm{is}\:\mathrm{an}\:\mathrm{elliptic}\:\mathrm{integral}\:\mathrm{of}\:\mathrm{the} \\ $$$$\:\:\:\:\:\:\:\:\:\:\mathrm{2}^{\mathrm{nd}} \:\mathrm{kind},\:\mathrm{the}\:\mathrm{second}\:\mathrm{one}\:\mathrm{of}\:\mathrm{the}\:\mathrm{1}^{\mathrm{st}} \:\mathrm{kind} \\ $$$$\:\:\:\:\:\:\:\:\:\:\mathrm{the}\:\mathrm{notation}\:\mathrm{follows},\:\mathrm{but}\:\mathrm{it}'\mathrm{s}\:\mathrm{beyond} \\ $$$$\:\:\:\:\:\:\:\:\:\:\mathrm{my}\:\mathrm{understanding}... \\ $$$$=\frac{\mathrm{2}\sqrt{\mathrm{p}+\mathrm{q}}}{\mathrm{q}}{E}\left({u}\mid\frac{\mathrm{2}{q}}{{p}+{q}}\right)−\frac{\mathrm{2}{p}\sqrt{{p}+{q}}}{{q}\left({p}+{q}\right)}{F}\left({u}\mid\frac{\mathrm{2}{q}}{{p}+{q}}\right)= \\ $$$$\:\:\:\:\:\:\:\:\:\:{u}=\frac{{x}}{\mathrm{2}} \\ $$$$=\frac{\mathrm{2}\sqrt{{p}+{q}}}{{q}}\left({E}\left(\frac{{x}}{\mathrm{2}}\mid\frac{\mathrm{2}{q}}{{p}+{q}}\right)−\frac{{p}}{{p}+{q}}{F}\left(\frac{{x}}{\mathrm{2}}\mid\frac{\mathrm{2}{q}}{{p}+{q}}\right)\right) \\ $$

Commented by prof Abdo imad last updated on 24/Apr/18

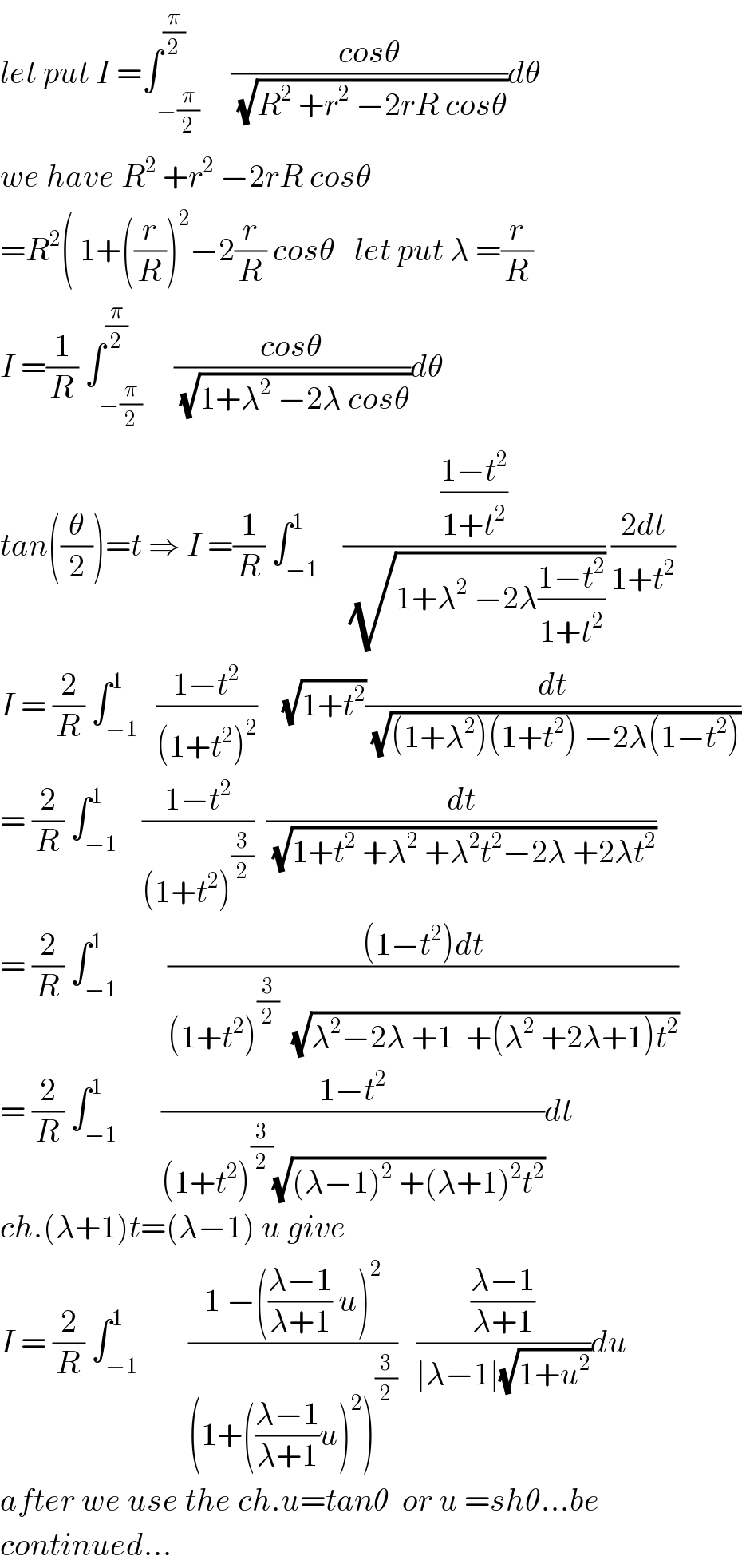
let put I =∫_(−(π/2)) ^(π/2) ((cosθ)/(√(R^2 +r^2 −2rR cosθ)))dθ we have R^2 +r^2 −2rR cosθ =R^2 ( 1+((r/R))^2 −2(r/R) cosθ let put λ =(r/R) I =(1/R) ∫_(−(π/2)) ^(π/2) ((cosθ)/(√(1+λ^2 −2λ cosθ)))dθ tan((θ/2))=t ⇒ I =(1/R) ∫_(−1) ^1 (((1−t^2 )/(1+t^2 ))/(√(1+λ^2 −2λ((1−t^2 )/(1+t^2 ))))) ((2dt)/(1+t^2 )) I = (2/R) ∫_(−1) ^1 ((1−t^2 )/((1+t^2 )^2 )) (√(1+t^2 ))(dt/(√((1+λ^2 )(1+t^2 ) −2λ(1−t^2 )))) = (2/R) ∫_(−1) ^1 ((1−t^2 )/((1+t^2 )^(3/2) )) (dt/(√(1+t^2 +λ^2 +λ^2 t^2 −2λ +2λt^2 ))) = (2/R) ∫_(−1) ^1 (((1−t^2 )dt)/((1+t^2 )^(3/2) (√(λ^2 −2λ +1 +(λ^2 +2λ+1)t^2 )))) = (2/R) ∫_(−1) ^1 ((1−t^2 )/((1+t^2 )^(3/2) (√((λ−1)^2 +(λ+1)^2 t^2 ))))dt ch.(λ+1)t=(λ−1) u give I = (2/R) ∫_(−1) ^1 ((1 −(((λ−1)/(λ+1)) u)^2 )/((1+(((λ−1)/(λ+1))u)^2 )^(3/2) )) (((λ−1)/(λ+1))/(∣λ−1∣(√(1+u^2 ))))du after we use the ch.u=tanθ or u =shθ...be continued...

$${let}\:{put}\:{I}\:=\int_{−\frac{\pi}{\mathrm{2}}} ^{\frac{\pi}{\mathrm{2}}} \:\:\:\:\frac{{cos}\theta}{\sqrt{{R}^{\mathrm{2}} \:+{r}^{\mathrm{2}} \:−\mathrm{2}{rR}\:{cos}\theta}}{d}\theta \\ $$$${we}\:{have}\:{R}^{\mathrm{2}} \:+{r}^{\mathrm{2}} \:−\mathrm{2}{rR}\:{cos}\theta \\ $$$$={R}^{\mathrm{2}} \left(\:\mathrm{1}+\left(\frac{{r}}{{R}}\right)^{\mathrm{2}} −\mathrm{2}\frac{{r}}{{R}}\:{cos}\theta\:\:\:{let}\:{put}\:\lambda\:=\frac{{r}}{{R}}\right. \\ $$$${I}\:=\frac{\mathrm{1}}{{R}}\:\int_{−\frac{\pi}{\mathrm{2}}} ^{\frac{\pi}{\mathrm{2}}} \:\:\:\:\frac{{cos}\theta}{\sqrt{\mathrm{1}+\lambda^{\mathrm{2}} \:−\mathrm{2}\lambda\:{cos}\theta}}{d}\theta \\ $$$${tan}\left(\frac{\theta}{\mathrm{2}}\right)={t}\:\Rightarrow\:{I}\:=\frac{\mathrm{1}}{{R}}\:\int_{−\mathrm{1}} ^{\mathrm{1}} \:\:\:\frac{\frac{\mathrm{1}−{t}^{\mathrm{2}} }{\mathrm{1}+{t}^{\mathrm{2}} }}{\sqrt{\mathrm{1}+\lambda^{\mathrm{2}} \:−\mathrm{2}\lambda\frac{\mathrm{1}−{t}^{\mathrm{2}} }{\mathrm{1}+{t}^{\mathrm{2}} }}}\:\frac{\mathrm{2}{dt}}{\mathrm{1}+{t}^{\mathrm{2}} } \\ $$$${I}\:=\:\frac{\mathrm{2}}{{R}}\:\int_{−\mathrm{1}} ^{\mathrm{1}} \:\:\frac{\mathrm{1}−{t}^{\mathrm{2}} }{\left(\mathrm{1}+{t}^{\mathrm{2}} \right)^{\mathrm{2}} }\:\:\:\:\sqrt{\mathrm{1}+{t}^{\mathrm{2}} }\frac{{dt}}{\sqrt{\left(\mathrm{1}+\lambda^{\mathrm{2}} \right)\left(\mathrm{1}+{t}^{\mathrm{2}} \right)\:−\mathrm{2}\lambda\left(\mathrm{1}−{t}^{\mathrm{2}} \right)}} \\ $$$$=\:\frac{\mathrm{2}}{{R}}\:\int_{−\mathrm{1}} ^{\mathrm{1}} \:\:\:\frac{\mathrm{1}−{t}^{\mathrm{2}} }{\left(\mathrm{1}+{t}^{\mathrm{2}} \right)^{\frac{\mathrm{3}}{\mathrm{2}}} }\:\:\frac{{dt}}{\sqrt{\mathrm{1}+{t}^{\mathrm{2}} \:+\lambda^{\mathrm{2}} \:+\lambda^{\mathrm{2}} {t}^{\mathrm{2}} −\mathrm{2}\lambda\:+\mathrm{2}\lambda{t}^{\mathrm{2}} }} \\ $$$$=\:\frac{\mathrm{2}}{{R}}\:\int_{−\mathrm{1}} ^{\mathrm{1}} \:\:\:\:\:\:\:\frac{\left(\mathrm{1}−{t}^{\mathrm{2}} \right){dt}}{\left(\mathrm{1}+{t}^{\mathrm{2}} \right)^{\frac{\mathrm{3}}{\mathrm{2}}} \:\:\sqrt{\lambda^{\mathrm{2}} −\mathrm{2}\lambda\:+\mathrm{1}\:\:+\left(\lambda^{\mathrm{2}} \:+\mathrm{2}\lambda+\mathrm{1}\right){t}^{\mathrm{2}} }} \\ $$$$=\:\frac{\mathrm{2}}{{R}}\:\int_{−\mathrm{1}} ^{\mathrm{1}} \:\:\:\:\:\:\frac{\mathrm{1}−{t}^{\mathrm{2}} }{\left(\mathrm{1}+{t}^{\mathrm{2}} \right)^{\frac{\mathrm{3}}{\mathrm{2}}} \sqrt{\left(\lambda−\mathrm{1}\right)^{\mathrm{2}} \:+\left(\lambda+\mathrm{1}\right)^{\mathrm{2}} {t}^{\mathrm{2}} }}{dt} \\ $$$${ch}.\left(\lambda+\mathrm{1}\right){t}=\left(\lambda−\mathrm{1}\right)\:{u}\:{give} \\ $$$${I}\:=\:\frac{\mathrm{2}}{{R}}\:\int_{−\mathrm{1}} ^{\mathrm{1}} \:\:\:\:\:\:\:\frac{\mathrm{1}\:−\left(\frac{\lambda−\mathrm{1}}{\lambda+\mathrm{1}}\:{u}\right)^{\mathrm{2}} }{\left(\mathrm{1}+\left(\frac{\lambda−\mathrm{1}}{\lambda+\mathrm{1}}{u}\right)^{\mathrm{2}} \right)^{\frac{\mathrm{3}}{\mathrm{2}}} }\:\:\:\frac{\frac{\lambda−\mathrm{1}}{\lambda+\mathrm{1}}}{\mid\lambda−\mathrm{1}\mid\sqrt{\mathrm{1}+{u}^{\mathrm{2}} }}{du} \\ $$$${after}\:{we}\:{use}\:{the}\:{ch}.{u}={tan}\theta\:\:{or}\:{u}\:={sh}\theta...{be} \\ $$$${continued}... \\ $$

Commented by 33 last updated on 24/Apr/18

To sir MJS , sir we cannot consider a, b and c as constants as they are dependent on cos θ . and you do not know a b c as a function of α and vice versa.

$${To}\:{sir}\:{MJS}\:,\:{sir}\:{we}\:{cannot} \\ $$$${consider}\:{a},\:{b}\:{and}\:{c}\:\:\:{as}\: \\ $$$${constants}\:{as}\:{they}\:{are}\: \\ $$$${dependent}\:{on}\:{cos}\:\theta\:. \\ $$$${and}\:{you}\:{do}\:{not}\:{know} \\ $$$${a}\:{b}\:{c}\:\:\:{as}\:{a}\:{function}\:{of}\:\alpha\: \\ $$$${and}\:{vice}\:{versa}. \\ $$

Commented by MJS last updated on 24/Apr/18

you′re right

$$\mathrm{you}'\mathrm{re}\:\mathrm{right} \\ $$

Commented by MJS last updated on 24/Apr/18

...this leads to an elliptic integral and it might be impossible to exactly solve it

$$...\mathrm{this}\:\mathrm{leads}\:\mathrm{to}\:\mathrm{an}\:\mathrm{elliptic}\:\mathrm{integral}\:\mathrm{and}\:\mathrm{it} \\ $$$$\mathrm{might}\:\mathrm{be}\:\mathrm{impossible}\:\mathrm{to}\:\mathrm{exactly}\:\mathrm{solve}\:\mathrm{it} \\ $$

Commented by 33 last updated on 24/Apr/18

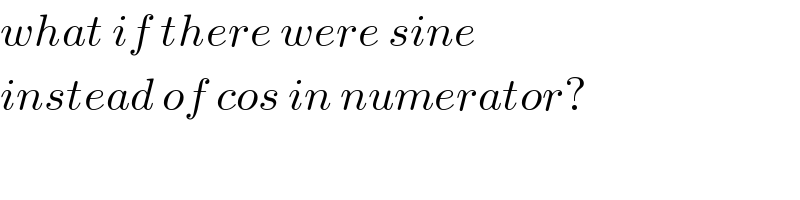
what if there were sine instead of cos in numerator?

$${what}\:{if}\:{there}\:{were}\:{sine}\: \\ $$$${instead}\:{of}\:{cos}\:{in}\:{numerator}? \\ $$

Commented by MJS last updated on 24/Apr/18

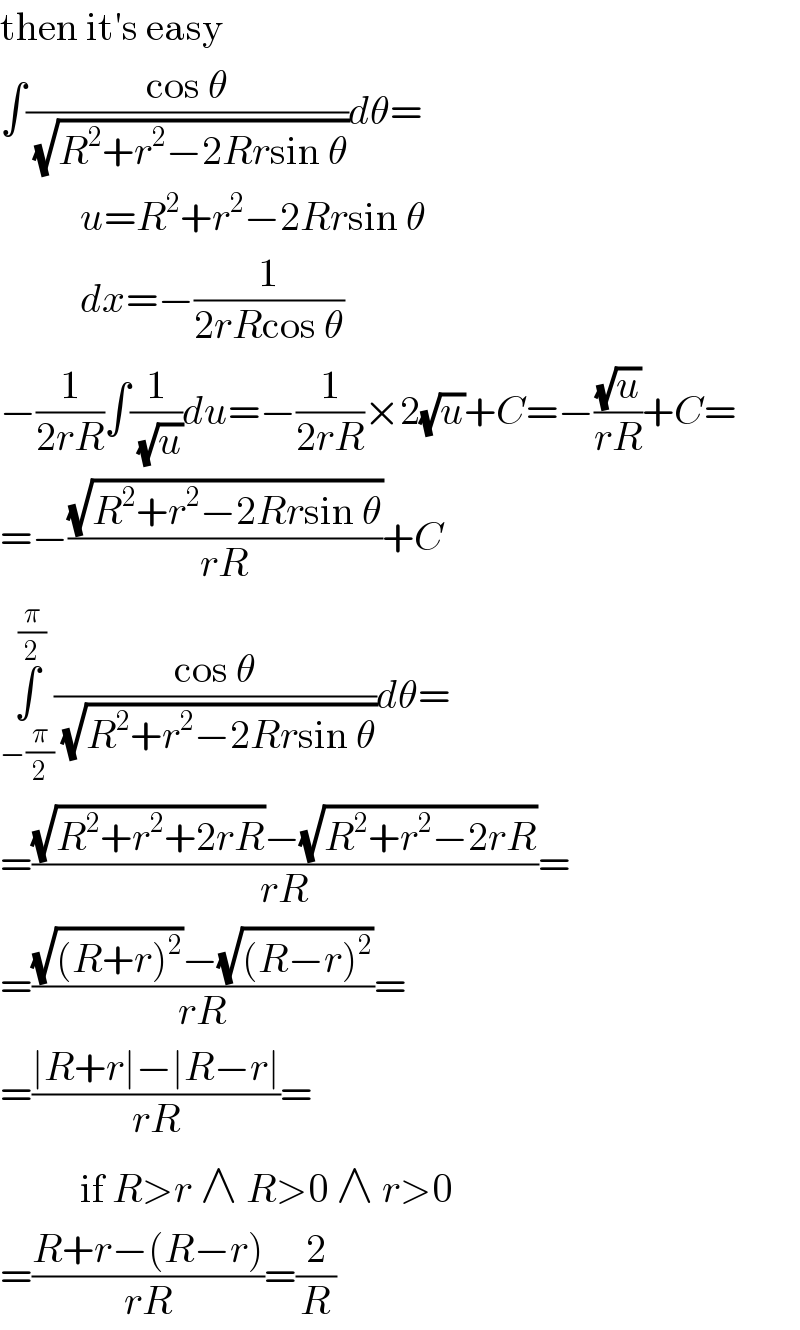
then it′s easy ∫((cos θ)/(√(R^2 +r^2 −2Rrsin θ)))dθ= u=R^2 +r^2 −2Rrsin θ dx=−(1/(2rRcos θ)) −(1/(2rR))∫(1/(√u))du=−(1/(2rR))×2(√u)+C=−((√u)/(rR))+C= =−((√(R^2 +r^2 −2Rrsin θ))/(rR))+C ∫_(−(π/2)) ^(π/2) ((cos θ)/(√(R^2 +r^2 −2Rrsin θ)))dθ= =(((√(R^2 +r^2 +2rR))−(√(R^2 +r^2 −2rR)))/(rR))= =(((√((R+r)^2 ))−(√((R−r)^2 )))/(rR))= =((∣R+r∣−∣R−r∣)/(rR))= if R>r ∧ R>0 ∧ r>0 =((R+r−(R−r))/(rR))=(2/R)

$$\mathrm{then}\:\mathrm{it}'\mathrm{s}\:\mathrm{easy} \\ $$$$\int\frac{\mathrm{cos}\:\theta}{\sqrt{{R}^{\mathrm{2}} +{r}^{\mathrm{2}} −\mathrm{2}{Rr}\mathrm{sin}\:\theta}}{d}\theta= \\ $$$$\:\:\:\:\:\:\:\:\:\:{u}={R}^{\mathrm{2}} +{r}^{\mathrm{2}} −\mathrm{2}{Rr}\mathrm{sin}\:\theta \\ $$$$\:\:\:\:\:\:\:\:\:\:{dx}=−\frac{\mathrm{1}}{\mathrm{2}{rR}\mathrm{cos}\:\theta} \\ $$$$−\frac{\mathrm{1}}{\mathrm{2}{rR}}\int\frac{\mathrm{1}}{\sqrt{{u}}}{du}=−\frac{\mathrm{1}}{\mathrm{2}{rR}}×\mathrm{2}\sqrt{{u}}+{C}=−\frac{\sqrt{{u}}}{{rR}}+{C}= \\ $$$$=−\frac{\sqrt{{R}^{\mathrm{2}} +{r}^{\mathrm{2}} −\mathrm{2}{Rr}\mathrm{sin}\:\theta}}{{rR}}+{C} \\ $$$$\underset{−\frac{\pi}{\mathrm{2}}} {\overset{\frac{\pi}{\mathrm{2}}} {\int}}\frac{\mathrm{cos}\:\theta}{\sqrt{{R}^{\mathrm{2}} +{r}^{\mathrm{2}} −\mathrm{2}{Rr}\mathrm{sin}\:\theta}}{d}\theta= \\ $$$$=\frac{\sqrt{{R}^{\mathrm{2}} +{r}^{\mathrm{2}} +\mathrm{2}{rR}}−\sqrt{{R}^{\mathrm{2}} +{r}^{\mathrm{2}} −\mathrm{2}{rR}}}{{rR}}= \\ $$$$=\frac{\sqrt{\left({R}+{r}\right)^{\mathrm{2}} }−\sqrt{\left({R}−{r}\right)^{\mathrm{2}} }}{{rR}}= \\ $$$$=\frac{\mid{R}+{r}\mid−\mid{R}−{r}\mid}{{rR}}= \\ $$$$\:\:\:\:\:\:\:\:\:\:\mathrm{if}\:{R}>{r}\:\wedge\:{R}>\mathrm{0}\:\wedge\:{r}>\mathrm{0} \\ $$$$=\frac{{R}+{r}−\left({R}−{r}\right)}{{rR}}=\frac{\mathrm{2}}{{R}} \\ $$

Commented by MJS last updated on 24/Apr/18

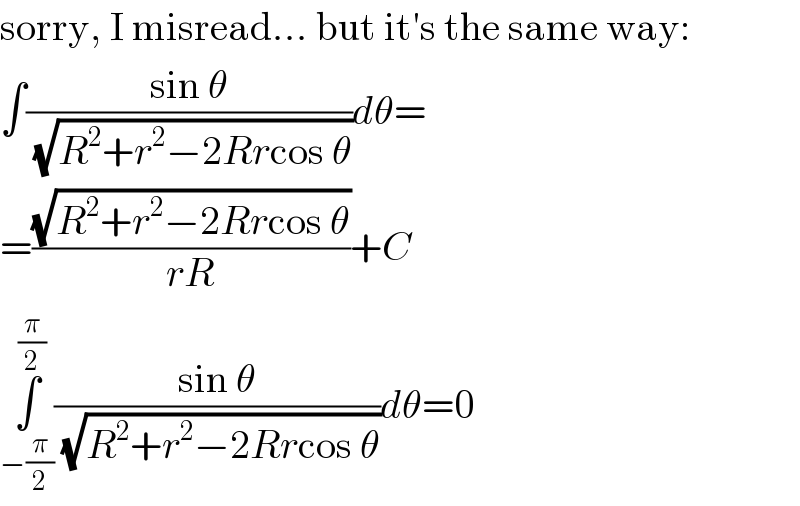
sorry, I misread... but it′s the same way: ∫((sin θ)/(√(R^2 +r^2 −2Rrcos θ)))dθ= =((√(R^2 +r^2 −2Rrcos θ))/(rR))+C ∫_(−(π/2)) ^(π/2) ((sin θ)/(√(R^2 +r^2 −2Rrcos θ)))dθ=0

$$\mathrm{sorry},\:\mathrm{I}\:\mathrm{misread}...\:\mathrm{but}\:\mathrm{it}'\mathrm{s}\:\mathrm{the}\:\mathrm{same}\:\mathrm{way}: \\ $$$$\int\frac{\mathrm{sin}\:\theta}{\sqrt{{R}^{\mathrm{2}} +{r}^{\mathrm{2}} −\mathrm{2}{Rr}\mathrm{cos}\:\theta}}{d}\theta= \\ $$$$=\frac{\sqrt{{R}^{\mathrm{2}} +{r}^{\mathrm{2}} −\mathrm{2}{Rr}\mathrm{cos}\:\theta}}{{rR}}+{C} \\ $$$$\underset{−\frac{\pi}{\mathrm{2}}} {\overset{\frac{\pi}{\mathrm{2}}} {\int}}\frac{\mathrm{sin}\:\theta}{\sqrt{{R}^{\mathrm{2}} +{r}^{\mathrm{2}} −\mathrm{2}{Rr}\mathrm{cos}\:\theta}}{d}\theta=\mathrm{0} \\ $$

Commented by prof Abdo imad last updated on 25/Apr/18

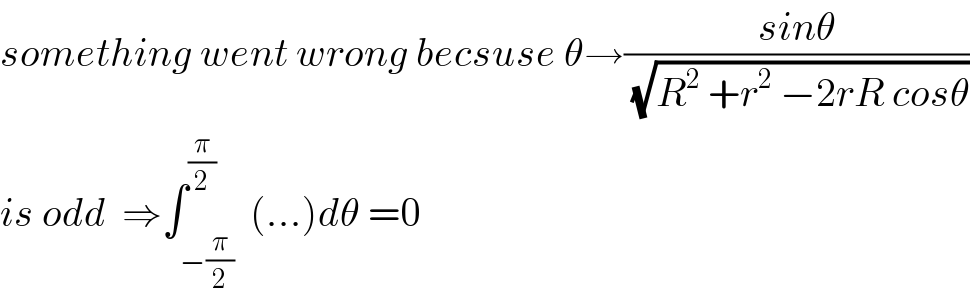
something went wrong becsuse θ→((sinθ)/(√(R^2 +r^2 −2rR cosθ))) is odd ⇒∫_(−(π/2)) ^(π/2) (...)dθ =0

$${something}\:{went}\:{wrong}\:{becsuse}\:\theta\rightarrow\frac{{sin}\theta}{\sqrt{{R}^{\mathrm{2}} \:+{r}^{\mathrm{2}} \:−\mathrm{2}{rR}\:{cos}\theta}} \\ $$$${is}\:{odd}\:\:\Rightarrow\int_{−\frac{\pi}{\mathrm{2}}} ^{\frac{\pi}{\mathrm{2}}} \:\left(...\right){d}\theta\:=\mathrm{0} \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com