
Question Number 86426 by M±th+et£s last updated on 28/Mar/20
![solve in R x^3 −5=[x]](Q86426.png)
$${solve}\:{in}\:{R} \\ $$$${x}^{\mathrm{3}} −\mathrm{5}=\left[{x}\right] \\ $$
Answered by mr W last updated on 28/Mar/20
![let x=n+δ (n+δ)^3 −5=[x]=n (n+δ)^3 −n=5 with n=0, LHS<1 with n=2, LHS≥6 ⇒n=1 (1+δ)^3 −1=5 (1+δ)^3 =6 δ=(6)^(1/3) −1 ⇒x=n+δ=(6)^(1/3) ≈1.81712](Q86434.png)
$${let}\:{x}={n}+\delta \\ $$$$\left({n}+\delta\right)^{\mathrm{3}} −\mathrm{5}=\left[{x}\right]={n} \\ $$$$\left({n}+\delta\right)^{\mathrm{3}} −{n}=\mathrm{5} \\ $$$${with}\:{n}=\mathrm{0},\:{LHS}<\mathrm{1} \\ $$$${with}\:{n}=\mathrm{2},\:{LHS}\geqslant\mathrm{6} \\ $$$$\Rightarrow{n}=\mathrm{1} \\ $$$$\left(\mathrm{1}+\delta\right)^{\mathrm{3}} −\mathrm{1}=\mathrm{5} \\ $$$$\left(\mathrm{1}+\delta\right)^{\mathrm{3}} =\mathrm{6} \\ $$$$\delta=\sqrt[{\mathrm{3}}]{\mathrm{6}}−\mathrm{1} \\ $$$$\Rightarrow{x}={n}+\delta=\sqrt[{\mathrm{3}}]{\mathrm{6}}\approx\mathrm{1}.\mathrm{81712} \\ $$
Commented by M±th+et£s last updated on 28/Mar/20

$${god}\:{bless}\:{you}\:{sir} \\ $$
Answered by MJS last updated on 28/Mar/20
![x^3 =5+[x] −8≤x^3 <−1 ⇒ 5+[x]=3 −1≤x^3 <0 ⇒ 5+[x]=4 0≤x^3 <1 ⇒ 5+[x]=5 1≤x^3 <8 ⇒ 5+[x]=6 ⇒ x^3 =6 ⇔ x=(6)^(1/3) 8≤x^3 <27 ⇒ 5+[x]=7](Q86432.png)
$${x}^{\mathrm{3}} =\mathrm{5}+\left[{x}\right] \\ $$$$−\mathrm{8}\leqslant{x}^{\mathrm{3}} <−\mathrm{1}\:\Rightarrow\:\mathrm{5}+\left[{x}\right]=\mathrm{3} \\ $$$$−\mathrm{1}\leqslant{x}^{\mathrm{3}} <\mathrm{0}\:\Rightarrow\:\mathrm{5}+\left[{x}\right]=\mathrm{4} \\ $$$$\mathrm{0}\leqslant{x}^{\mathrm{3}} <\mathrm{1}\:\Rightarrow\:\mathrm{5}+\left[{x}\right]=\mathrm{5} \\ $$$$\mathrm{1}\leqslant{x}^{\mathrm{3}} <\mathrm{8}\:\Rightarrow\:\mathrm{5}+\left[{x}\right]=\mathrm{6}\:\Rightarrow\:{x}^{\mathrm{3}} =\mathrm{6}\:\Leftrightarrow\:{x}=\sqrt[{\mathrm{3}}]{\mathrm{6}} \\ $$$$\mathrm{8}\leqslant{x}^{\mathrm{3}} <\mathrm{27}\:\Rightarrow\:\mathrm{5}+\left[{x}\right]=\mathrm{7} \\ $$$$ \\ $$
Commented by M±th+et£s last updated on 28/Mar/20

$${god}\:{bless}\:{you}\:{sir} \\ $$
Commented by M±th+et£s last updated on 28/Mar/20
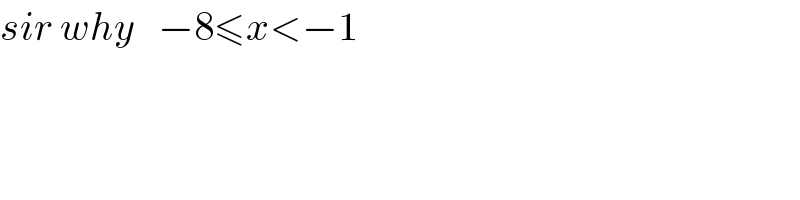
$${sir}\:{why}\:\:\:−\mathrm{8}\leqslant{x}<−\mathrm{1}\:\:\: \\ $$
Commented by M±th+et£s last updated on 28/Mar/20

$${sorry}\:{x}^{\mathrm{3}} \\ $$
Commented by MJS last updated on 28/Mar/20
![−8≤x^3 <−1 ⇔ −2≤x<−1 ⇒ [x]=−2 generally ∀a∈Z: a≤x<a+1 ⇒ [x]=a and a≤x<a+1 ⇔ a^3 ≤x^3 <(a+1)^3](Q86474.png)
$$−\mathrm{8}\leqslant{x}^{\mathrm{3}} <−\mathrm{1}\:\Leftrightarrow\:−\mathrm{2}\leqslant{x}<−\mathrm{1}\:\Rightarrow\:\left[{x}\right]=−\mathrm{2} \\ $$$$\mathrm{generally} \\ $$$$\forall{a}\in\mathbb{Z}:\:{a}\leqslant{x}<{a}+\mathrm{1}\:\Rightarrow\:\left[{x}\right]={a} \\ $$$$\mathrm{and}\:{a}\leqslant{x}<{a}+\mathrm{1}\:\Leftrightarrow\:{a}^{\mathrm{3}} \leqslant{x}^{\mathrm{3}} <\left({a}+\mathrm{1}\right)^{\mathrm{3}} \\ $$
Commented by M±th+et£s last updated on 28/Mar/20

$${thank}\:{you}\:{sir} \\ $$
