Question and Answers Forum

All Questions      Topic List

None Questions

Previous in All Question      Next in All Question      

Previous in None      Next in None      

Question Number 101658 by mathocean1 last updated on 03/Jul/20

solve in R x^3 −4x−1=0

$${solve}\:{in}\:\mathbb{R} \\ $$$${x}^{\mathrm{3}} −\mathrm{4}{x}−\mathrm{1}=\mathrm{0} \\ $$

Answered by 1549442205 last updated on 04/Jul/20

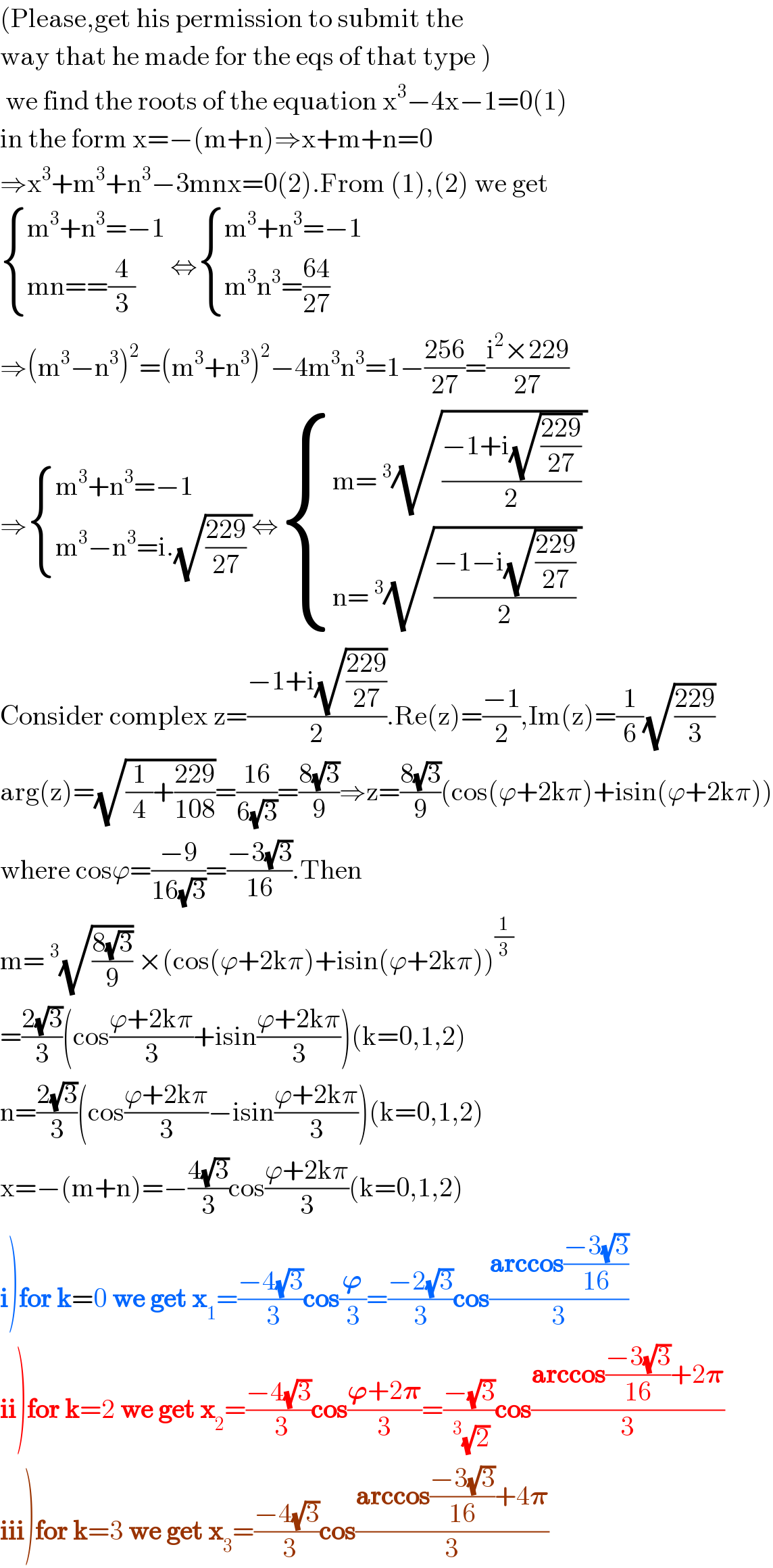
(Please,get his permission to submit the way that he made for the eqs of that type ) we find the roots of the equation x^3 −4x−1=0(1) in the form x=−(m+n)⇒x+m+n=0 ⇒x^3 +m^3 +n^3 −3mnx=0(2).From (1),(2) we get { ((m^3 +n^3 =−1)),((mn==(4/3))) :} ⇔ { ((m^3 +n^3 =−1)),((m^3 n^3 =((64)/(27)))) :} ⇒(m^3 −n^3 )^2 =(m^3 +n^3 )^2 −4m^3 n^3 =1−((256)/(27))=((i^2 ×229)/(27)) ⇒ { ((m^3 +n^3 =−1)),((m^3 −n^3 =i.(√(((229)/(27)) )))) :}⇔ { ((m=^3 (√(((−1+i(√((229)/(27))))/2) )))),((n=^3 (√(((−1−i(√((229)/(27))))/2) )))) :} Consider complex z=((−1+i(√((229)/(27))))/2).Re(z)=((−1)/2),Im(z)=(1/6)(√((229)/3)) arg(z)=(√((1/4)+((229)/(108))))=((16)/(6(√3)))=((8(√3))/9)⇒z=((8(√3))/9)(cos(ϕ+2kπ)+isin(ϕ+2kπ)) where cosϕ=((−9)/(16(√3)))=((−3(√3))/(16)).Then m=^3 (√((8(√3))/9)) ×(cos(ϕ+2kπ)+isin(ϕ+2kπ))^(1/3) =((2(√3))/3)(cos((ϕ+2kπ)/3)+isin((ϕ+2kπ)/3))(k=0,1,2) n=((2(√3))/3)(cos((ϕ+2kπ)/3)−isin((ϕ+2kπ)/3))(k=0,1,2) x=−(m+n)=−((4(√3))/3)cos((ϕ+2kπ)/3)(k=0,1,2) i)for k=0 we get x_1 =((−4(√3))/( 3))cos(𝛟/3)=((−2(√3))/3)cos((arccos((−3(√3))/(16)))/3) ii)for k=2 we get x_2 =((−4(√3))/( 3))cos((𝛟+2𝛑)/3)=((−(√3))/(^3 (√2)))cos((arccos((−3(√3))/(16))+2𝛑)/3) iii)for k=3 we get x_3 =((−4(√3))/( 3))cos((arccos((−3(√3))/(16))+4𝛑)/3)

$$\left(\mathrm{Please},\mathrm{get}\:\mathrm{his}\:\mathrm{permission}\:\mathrm{to}\:\mathrm{submit}\:\mathrm{the}\right. \\ $$$$\left.\mathrm{way}\:\mathrm{that}\:\mathrm{he}\:\mathrm{made}\:\mathrm{for}\:\mathrm{the}\:\mathrm{eqs}\:\mathrm{of}\:\mathrm{that}\:\mathrm{type}\:\right) \\ $$$$\:\mathrm{we}\:\mathrm{find}\:\mathrm{the}\:\mathrm{roots}\:\mathrm{of}\:\mathrm{the}\:\mathrm{equation}\:\mathrm{x}^{\mathrm{3}} −\mathrm{4x}−\mathrm{1}=\mathrm{0}\left(\mathrm{1}\right) \\ $$$$\mathrm{in}\:\mathrm{the}\:\mathrm{form}\:\mathrm{x}=−\left(\mathrm{m}+\mathrm{n}\right)\Rightarrow\mathrm{x}+\mathrm{m}+\mathrm{n}=\mathrm{0} \\ $$$$\Rightarrow\mathrm{x}^{\mathrm{3}} +\mathrm{m}^{\mathrm{3}} +\mathrm{n}^{\mathrm{3}} −\mathrm{3mnx}=\mathrm{0}\left(\mathrm{2}\right).\mathrm{From}\:\left(\mathrm{1}\right),\left(\mathrm{2}\right)\:\mathrm{we}\:\mathrm{get} \\ $$$$\begin{cases}{\mathrm{m}^{\mathrm{3}} +\mathrm{n}^{\mathrm{3}} =−\mathrm{1}}\\{\mathrm{mn}==\frac{\mathrm{4}}{\mathrm{3}}}\end{cases}\:\Leftrightarrow\begin{cases}{\mathrm{m}^{\mathrm{3}} +\mathrm{n}^{\mathrm{3}} =−\mathrm{1}}\\{\mathrm{m}^{\mathrm{3}} \mathrm{n}^{\mathrm{3}} =\frac{\mathrm{64}}{\mathrm{27}}}\end{cases} \\ $$$$\Rightarrow\left(\mathrm{m}^{\mathrm{3}} −\mathrm{n}^{\mathrm{3}} \right)^{\mathrm{2}} =\left(\mathrm{m}^{\mathrm{3}} +\mathrm{n}^{\mathrm{3}} \right)^{\mathrm{2}} −\mathrm{4m}^{\mathrm{3}} \mathrm{n}^{\mathrm{3}} =\mathrm{1}−\frac{\mathrm{256}}{\mathrm{27}}=\frac{\mathrm{i}^{\mathrm{2}} ×\mathrm{229}}{\mathrm{27}} \\ $$$$\Rightarrow\begin{cases}{\mathrm{m}^{\mathrm{3}} +\mathrm{n}^{\mathrm{3}} =−\mathrm{1}}\\{\mathrm{m}^{\mathrm{3}} −\mathrm{n}^{\mathrm{3}} =\mathrm{i}.\sqrt{\frac{\mathrm{229}}{\mathrm{27}}\:}}\end{cases}\Leftrightarrow\begin{cases}{\mathrm{m}=\:^{\mathrm{3}} \sqrt{\frac{−\mathrm{1}+\mathrm{i}\sqrt{\frac{\mathrm{229}}{\mathrm{27}}}}{\mathrm{2}}\:}}\\{\mathrm{n}=\:^{\mathrm{3}} \sqrt{\frac{−\mathrm{1}−\mathrm{i}\sqrt{\frac{\mathrm{229}}{\mathrm{27}}}}{\mathrm{2}}\:}}\end{cases} \\ $$$$\mathrm{Consider}\:\mathrm{complex}\:\mathrm{z}=\frac{−\mathrm{1}+\mathrm{i}\sqrt{\frac{\mathrm{229}}{\mathrm{27}}}}{\mathrm{2}}.\mathrm{Re}\left(\mathrm{z}\right)=\frac{−\mathrm{1}}{\mathrm{2}},\mathrm{Im}\left(\mathrm{z}\right)=\frac{\mathrm{1}}{\mathrm{6}}\sqrt{\frac{\mathrm{229}}{\mathrm{3}}} \\ $$$$\mathrm{arg}\left(\mathrm{z}\right)=\sqrt{\frac{\mathrm{1}}{\mathrm{4}}+\frac{\mathrm{229}}{\mathrm{108}}}=\frac{\mathrm{16}}{\mathrm{6}\sqrt{\mathrm{3}}}=\frac{\mathrm{8}\sqrt{\mathrm{3}}}{\mathrm{9}}\Rightarrow\mathrm{z}=\frac{\mathrm{8}\sqrt{\mathrm{3}}}{\mathrm{9}}\left(\mathrm{cos}\left(\varphi+\mathrm{2k}\pi\right)+\mathrm{isin}\left(\varphi+\mathrm{2k}\pi\right)\right) \\ $$$$\mathrm{where}\:\mathrm{cos}\varphi=\frac{−\mathrm{9}}{\mathrm{16}\sqrt{\mathrm{3}}}=\frac{−\mathrm{3}\sqrt{\mathrm{3}}}{\mathrm{16}}.\mathrm{Then} \\ $$$$\mathrm{m}=\:^{\mathrm{3}} \sqrt{\frac{\mathrm{8}\sqrt{\mathrm{3}}}{\mathrm{9}}}\:×\left(\mathrm{cos}\left(\varphi+\mathrm{2k}\pi\right)+\mathrm{isin}\left(\varphi+\mathrm{2k}\pi\right)\right)^{\frac{\mathrm{1}}{\mathrm{3}}} \\ $$$$=\frac{\mathrm{2}\sqrt{\mathrm{3}}}{\mathrm{3}}\left(\mathrm{cos}\frac{\varphi+\mathrm{2k}\pi}{\mathrm{3}}+\mathrm{isin}\frac{\varphi+\mathrm{2k}\pi}{\mathrm{3}}\right)\left(\mathrm{k}=\mathrm{0},\mathrm{1},\mathrm{2}\right) \\ $$$$\mathrm{n}=\frac{\mathrm{2}\sqrt{\mathrm{3}}}{\mathrm{3}}\left(\mathrm{cos}\frac{\varphi+\mathrm{2k}\pi}{\mathrm{3}}−\mathrm{isin}\frac{\varphi+\mathrm{2k}\pi}{\mathrm{3}}\right)\left(\mathrm{k}=\mathrm{0},\mathrm{1},\mathrm{2}\right) \\ $$$$\mathrm{x}=−\left(\mathrm{m}+\mathrm{n}\right)=−\frac{\mathrm{4}\sqrt{\mathrm{3}}}{\mathrm{3}}\mathrm{cos}\frac{\varphi+\mathrm{2k}\pi}{\mathrm{3}}\left(\mathrm{k}=\mathrm{0},\mathrm{1},\mathrm{2}\right) \\ $$$$\left.\boldsymbol{\mathrm{i}}\right)\boldsymbol{\mathrm{for}}\:\boldsymbol{\mathrm{k}}=\mathrm{0}\:\boldsymbol{\mathrm{we}}\:\boldsymbol{\mathrm{get}}\:\boldsymbol{\mathrm{x}}_{\mathrm{1}} =\frac{−\mathrm{4}\sqrt{\mathrm{3}}}{\:\mathrm{3}}\boldsymbol{\mathrm{cos}}\frac{\boldsymbol{\varphi}}{\mathrm{3}}=\frac{−\mathrm{2}\sqrt{\mathrm{3}}}{\mathrm{3}}\boldsymbol{\mathrm{cos}}\frac{\boldsymbol{\mathrm{arccos}}\frac{−\mathrm{3}\sqrt{\mathrm{3}}}{\mathrm{16}}}{\mathrm{3}} \\ $$$$\left.\boldsymbol{\mathrm{ii}}\right)\boldsymbol{\mathrm{for}}\:\boldsymbol{\mathrm{k}}=\mathrm{2}\:\boldsymbol{\mathrm{we}}\:\boldsymbol{\mathrm{get}}\:\boldsymbol{\mathrm{x}}_{\mathrm{2}} =\frac{−\mathrm{4}\sqrt{\mathrm{3}}}{\:\mathrm{3}}\boldsymbol{\mathrm{cos}}\frac{\boldsymbol{\varphi}+\mathrm{2}\boldsymbol{\pi}}{\mathrm{3}}=\frac{−\sqrt{\mathrm{3}}}{\:^{\mathrm{3}} \sqrt{\mathrm{2}}}\boldsymbol{\mathrm{cos}}\frac{\boldsymbol{\mathrm{arccos}}\frac{−\mathrm{3}\sqrt{\mathrm{3}}}{\mathrm{16}}+\mathrm{2}\boldsymbol{\pi}}{\mathrm{3}} \\ $$$$\left.\boldsymbol{\mathrm{iii}}\right)\boldsymbol{\mathrm{for}}\:\boldsymbol{\mathrm{k}}=\mathrm{3}\:\boldsymbol{\mathrm{we}}\:\boldsymbol{\mathrm{get}}\:\boldsymbol{\mathrm{x}}_{\mathrm{3}} =\frac{−\mathrm{4}\sqrt{\mathrm{3}}}{\:\mathrm{3}}\boldsymbol{\mathrm{cos}}\frac{\boldsymbol{\mathrm{arccos}}\frac{−\mathrm{3}\sqrt{\mathrm{3}}}{\mathrm{16}}+\mathrm{4}\boldsymbol{\pi}}{\mathrm{3}} \\ $$

Commented by 1549442205 last updated on 07/Jul/20

Great,thank you Sir.

$$\mathrm{Great},\mathrm{thank}\:\mathrm{you}\:\mathrm{Sir}. \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com