Question and Answers Forum

All Questions      Topic List

Algebra Questions

Previous in All Question      Next in All Question      

Previous in Algebra      Next in Algebra      

Question Number 177112 by mr W last updated on 01/Oct/22

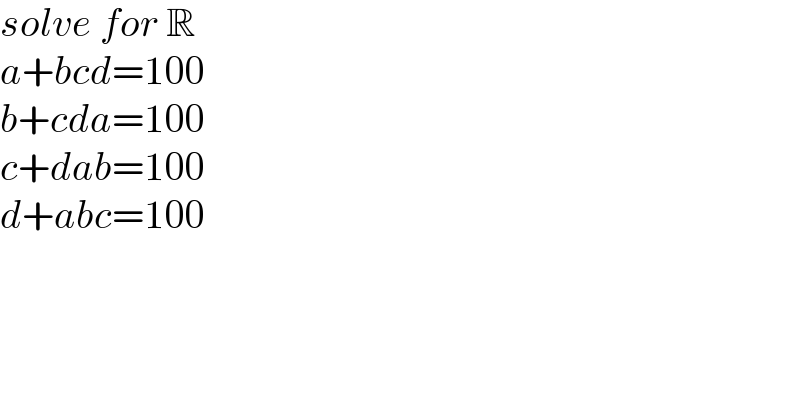
solve for R a+bcd=100 b+cda=100 c+dab=100 d+abc=100

$${solve}\:{for}\:\mathbb{R} \\ $$$${a}+{bcd}=\mathrm{100} \\ $$$${b}+{cda}=\mathrm{100} \\ $$$${c}+{dab}=\mathrm{100} \\ $$$${d}+{abc}=\mathrm{100} \\ $$

Answered by aleks041103 last updated on 01/Oct/22

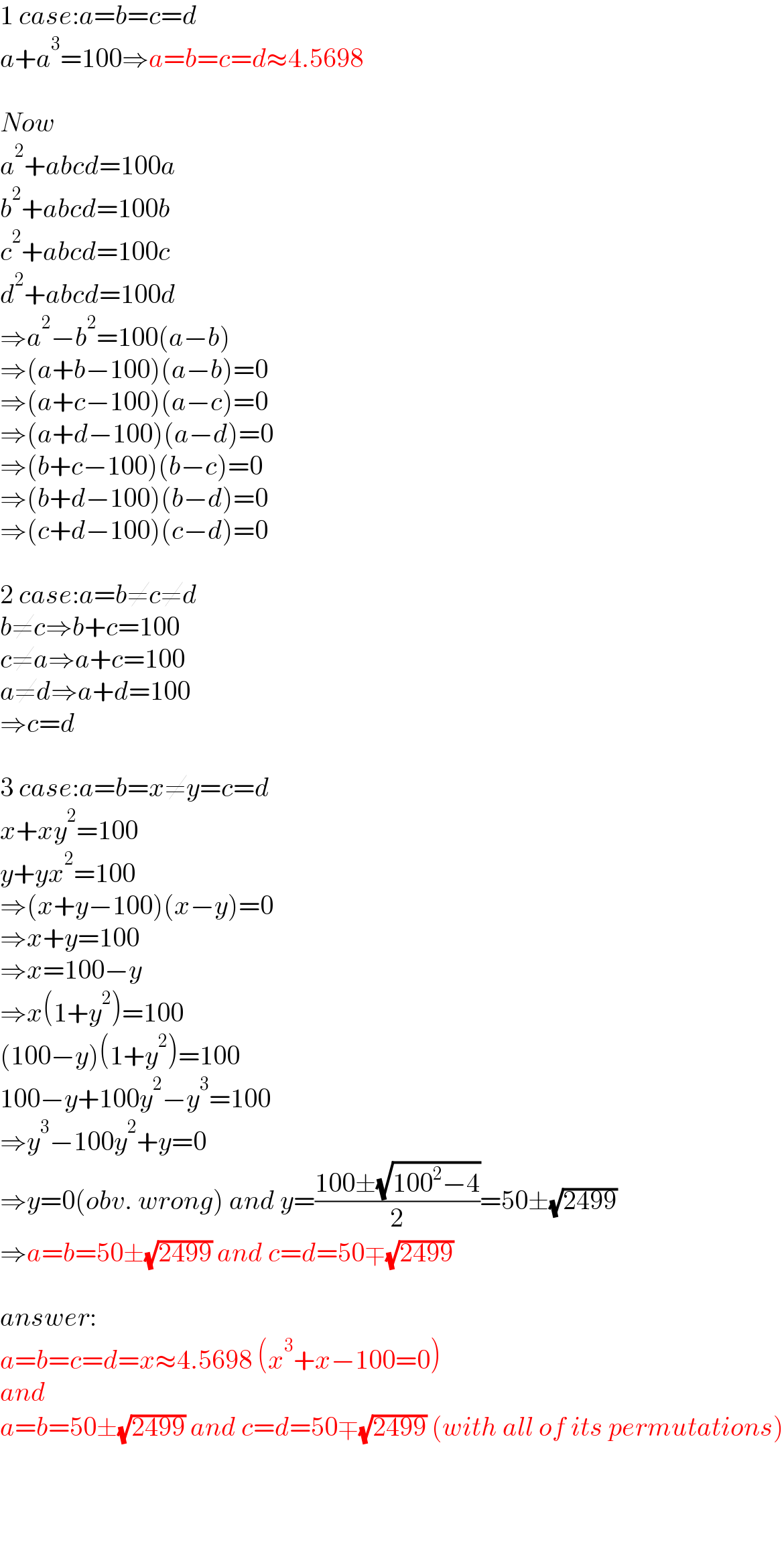
1 case:a=b=c=d a+a^3 =100⇒a=b=c=d≈4.5698 Now a^2 +abcd=100a b^2 +abcd=100b c^2 +abcd=100c d^2 +abcd=100d ⇒a^2 −b^2 =100(a−b) ⇒(a+b−100)(a−b)=0 ⇒(a+c−100)(a−c)=0 ⇒(a+d−100)(a−d)=0 ⇒(b+c−100)(b−c)=0 ⇒(b+d−100)(b−d)=0 ⇒(c+d−100)(c−d)=0 2 case:a=b≠c≠d b≠c⇒b+c=100 c≠a⇒a+c=100 a≠d⇒a+d=100 ⇒c=d 3 case:a=b=x≠y=c=d x+xy^2 =100 y+yx^2 =100 ⇒(x+y−100)(x−y)=0 ⇒x+y=100 ⇒x=100−y ⇒x(1+y^2 )=100 (100−y)(1+y^2 )=100 100−y+100y^2 −y^3 =100 ⇒y^3 −100y^2 +y=0 ⇒y=0(obv. wrong) and y=((100±(√(100^2 −4)))/2)=50±(√(2499)) ⇒a=b=50±(√(2499)) and c=d=50∓(√(2499)) answer: a=b=c=d=x≈4.5698 (x^3 +x−100=0) and a=b=50±(√(2499)) and c=d=50∓(√(2499)) (with all of its permutations)

$$\mathrm{1}\:{case}:{a}={b}={c}={d} \\ $$$${a}+{a}^{\mathrm{3}} =\mathrm{100}\Rightarrow{a}={b}={c}={d}\approx\mathrm{4}.\mathrm{5698} \\ $$$$ \\ $$$${Now} \\ $$$${a}^{\mathrm{2}} +{abcd}=\mathrm{100}{a} \\ $$$${b}^{\mathrm{2}} +{abcd}=\mathrm{100}{b} \\ $$$${c}^{\mathrm{2}} +{abcd}=\mathrm{100}{c} \\ $$$${d}^{\mathrm{2}} +{abcd}=\mathrm{100}{d} \\ $$$$\Rightarrow{a}^{\mathrm{2}} −{b}^{\mathrm{2}} =\mathrm{100}\left({a}−{b}\right) \\ $$$$\Rightarrow\left({a}+{b}−\mathrm{100}\right)\left({a}−{b}\right)=\mathrm{0} \\ $$$$\Rightarrow\left({a}+{c}−\mathrm{100}\right)\left({a}−{c}\right)=\mathrm{0} \\ $$$$\Rightarrow\left({a}+{d}−\mathrm{100}\right)\left({a}−{d}\right)=\mathrm{0} \\ $$$$\Rightarrow\left({b}+{c}−\mathrm{100}\right)\left({b}−{c}\right)=\mathrm{0} \\ $$$$\Rightarrow\left({b}+{d}−\mathrm{100}\right)\left({b}−{d}\right)=\mathrm{0} \\ $$$$\Rightarrow\left({c}+{d}−\mathrm{100}\right)\left({c}−{d}\right)=\mathrm{0} \\ $$$$ \\ $$$$\mathrm{2}\:{case}:{a}={b}\neq{c}\neq{d} \\ $$$${b}\neq{c}\Rightarrow{b}+{c}=\mathrm{100} \\ $$$${c}\neq{a}\Rightarrow{a}+{c}=\mathrm{100} \\ $$$${a}\neq{d}\Rightarrow{a}+{d}=\mathrm{100} \\ $$$$\Rightarrow{c}={d} \\ $$$$ \\ $$$$\mathrm{3}\:{case}:{a}={b}={x}\neq{y}={c}={d} \\ $$$${x}+{xy}^{\mathrm{2}} =\mathrm{100} \\ $$$${y}+{yx}^{\mathrm{2}} =\mathrm{100} \\ $$$$\Rightarrow\left({x}+{y}−\mathrm{100}\right)\left({x}−{y}\right)=\mathrm{0} \\ $$$$\Rightarrow{x}+{y}=\mathrm{100} \\ $$$$\Rightarrow{x}=\mathrm{100}−{y} \\ $$$$\Rightarrow{x}\left(\mathrm{1}+{y}^{\mathrm{2}} \right)=\mathrm{100} \\ $$$$\left(\mathrm{100}−{y}\right)\left(\mathrm{1}+{y}^{\mathrm{2}} \right)=\mathrm{100} \\ $$$$\mathrm{100}−{y}+\mathrm{100}{y}^{\mathrm{2}} −{y}^{\mathrm{3}} =\mathrm{100} \\ $$$$\Rightarrow{y}^{\mathrm{3}} −\mathrm{100}{y}^{\mathrm{2}} +{y}=\mathrm{0} \\ $$$$\Rightarrow{y}=\mathrm{0}\left({obv}.\:{wrong}\right)\:{and}\:{y}=\frac{\mathrm{100}\pm\sqrt{\mathrm{100}^{\mathrm{2}} −\mathrm{4}}}{\mathrm{2}}=\mathrm{50}\pm\sqrt{\mathrm{2499}} \\ $$$$\Rightarrow{a}={b}=\mathrm{50}\pm\sqrt{\mathrm{2499}}\:{and}\:{c}={d}=\mathrm{50}\mp\sqrt{\mathrm{2499}} \\ $$$$ \\ $$$${answer}: \\ $$$${a}={b}={c}={d}={x}\approx\mathrm{4}.\mathrm{5698}\:\left({x}^{\mathrm{3}} +{x}−\mathrm{100}=\mathrm{0}\right) \\ $$$${and} \\ $$$${a}={b}=\mathrm{50}\pm\sqrt{\mathrm{2499}}\:{and}\:{c}={d}=\mathrm{50}\mp\sqrt{\mathrm{2499}}\:\left({with}\:{all}\:{of}\:{its}\:{permutations}\right) \\ $$$$ \\ $$$$ \\ $$

Commented by mr W last updated on 01/Oct/22

thanks sir!

$${thanks}\:{sir}! \\ $$

Commented by Tawa11 last updated on 02/Oct/22

Great sirs

$$\mathrm{Great}\:\mathrm{sirs} \\ $$

Answered by Rasheed.Sindhi last updated on 02/Oct/22

solve for R ;a+bcd=100,b+cda=100, c+dab=100,d+abc=100_ (i)−(ii): a−cda−b+bcd=0 a(1−cd)−b(1−cd)=0 (a−b)(1−cd)=0 a=b ∣ cd=1 (i)+(ii): (a+b)(1+cd)=200 (a=b ∨ cd=1)∧ (a+b)(1+cd)=200 2a(1+1)=200 a=50 Similar way: (a=c ∨ bd=1) a=d ∣ bc=1 b=c ∣ ad=1 b=d ∣ ac=1 c=d ∣ ab=1 Similar way a=c ∣ bd=1...... a=d ∣ bc=1 b=c ∣ ad=1 b=d ∣ ac=1 c=d ∣ ab=1 a=b=c=d ....

$${solve}\:{for}\:\mathbb{R}\:;{a}+{bcd}=\mathrm{100},{b}+{cda}=\mathrm{100}, \\ $$$$\underline{{c}+{dab}=\mathrm{100},{d}+{abc}=\mathrm{100}_{} \:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:} \\ $$$$\left({i}\right)−\left({ii}\right): \\ $$$${a}−{cda}−{b}+{bcd}=\mathrm{0} \\ $$$${a}\left(\mathrm{1}−{cd}\right)−{b}\left(\mathrm{1}−{cd}\right)=\mathrm{0} \\ $$$$\left({a}−{b}\right)\left(\mathrm{1}−{cd}\right)=\mathrm{0} \\ $$$${a}={b}\:\mid\:\:{cd}=\mathrm{1} \\ $$$$\left({i}\right)+\left({ii}\right): \\ $$$$\left({a}+{b}\right)\left(\mathrm{1}+{cd}\right)=\mathrm{200} \\ $$$$\left({a}={b}\:\vee\:{cd}=\mathrm{1}\right)\wedge\:\left({a}+{b}\right)\left(\mathrm{1}+{cd}\right)=\mathrm{200} \\ $$$$\mathrm{2}{a}\left(\mathrm{1}+\mathrm{1}\right)=\mathrm{200} \\ $$$${a}=\mathrm{50} \\ $$$${Similar}\:{way}: \\ $$$$\left({a}={c}\:\vee\:{bd}=\mathrm{1}\right) \\ $$$${a}={d}\:\mid\:{bc}=\mathrm{1} \\ $$$${b}={c}\:\mid\:{ad}=\mathrm{1} \\ $$$${b}={d}\:\mid\:{ac}=\mathrm{1} \\ $$$${c}={d}\:\mid\:{ab}=\mathrm{1} \\ $$$${Similar}\:{way} \\ $$$${a}={c}\:\mid\:{bd}=\mathrm{1}...... \\ $$$${a}={d}\:\mid\:{bc}=\mathrm{1} \\ $$$${b}={c}\:\mid\:{ad}=\mathrm{1} \\ $$$${b}={d}\:\mid\:{ac}=\mathrm{1} \\ $$$${c}={d}\:\mid\:{ab}=\mathrm{1} \\ $$$${a}={b}={c}={d}\: \\ $$$$.... \\ $$

Commented by mr W last updated on 01/Oct/22

thanks sir!

$${thanks}\:{sir}! \\ $$

Answered by mr W last updated on 01/Oct/22

a^2 −100a+abcd=0 b^2 −100b+abcd=0 c^2 −100c+abcd=0 d^2 −100d+abcd=0 let k=abcd we see a,b,c,d are the roots of the uadratic e uation x^2 −100x+k=0. since a quadratic equation can have at most two different roots, we can only have following cases: case 1: two roots are equal, ⇒a,b,c,d are equal. a=b=c=d a^3 +a−100=0 ⇒a=((((√(202503))/9)+50))^(1/3) −((((√(202503))/9)−50))^(1/3) ≈4.56978 ⇒(a,b,c,d)=(4.56978,4.56978,4.56978,4.56978) case 2: two roots are different, a≠b=c=d (as example) a+b^3 =100 ...(i) b+ab^2 =100 ...(ii) (i)−(ii): (a−b)(1−b^2 )=0 ⇒1−b^2 =0 ⇒b=±1 ⇒a=100−b^3 =100∓1 i.e. b=1, a=99 or b=−1, a=101 ⇒(a,b,c,d)=(99,1,1,1) or (101,−1,−1,−1) and we can exchange a with b,c,d. case 3: two roots are different, a=b≠c=d (as example) a+ac^2 =100 ...(iii) c+a^2 c=100 ...(iv) (iii)−(iv): (a−c)(1−ac)=0 ⇒1−ac=0 ⇒ac=1 ⇒a+c=100 ⇒a,c are roots of z^2 −100z+1=0 a,c=50±(√(50^2 −1))=50±7(√(51)) ⇒(a,b,c,d)=(50+7(√(51)),50+7(√(51)),50−7(√(51)),50−7(√(51))) or (50−7(√(51)),50−7(√(51)),50+7(√(51)),50+7(√(51))) and we can exchange a, c with a,b or a,d.

$${a}^{\mathrm{2}} −\mathrm{100}{a}+{abcd}=\mathrm{0} \\ $$$${b}^{\mathrm{2}} −\mathrm{100}{b}+{abcd}=\mathrm{0} \\ $$$${c}^{\mathrm{2}} −\mathrm{100}{c}+{abcd}=\mathrm{0} \\ $$$${d}^{\mathrm{2}} −\mathrm{100}{d}+{abcd}=\mathrm{0} \\ $$$${let}\:{k}={abcd} \\ $$$${we}\:{see}\:{a},{b},{c},{d}\:{are}\:{the}\:{roots}\:{of}\:{the} \\ $$$$ {uadratic}\:{e} {uation}\:{x}^{\mathrm{2}} −\mathrm{100}{x}+{k}=\mathrm{0}. \\ $$$${since}\:{a}\:{quadratic}\:{equation}\:{can}\:{have} \\ $$$${at}\:{most}\:{two}\:{different}\:{roots},\:{we}\:{can} \\ $$$${only}\:{have}\:{following}\:{cases}: \\ $$$$ \\ $$$$\boldsymbol{{case}}\:\mathrm{1}:\:\boldsymbol{{two}}\:\boldsymbol{{roots}}\:\boldsymbol{{are}}\:\boldsymbol{{equal}}, \\ $$$$\Rightarrow{a},{b},{c},{d}\:{are}\:{equal}. \\ $$$${a}={b}={c}={d} \\ $$$${a}^{\mathrm{3}} +{a}−\mathrm{100}=\mathrm{0} \\ $$$$\Rightarrow{a}=\sqrt[{\mathrm{3}}]{\frac{\sqrt{\mathrm{202503}}}{\mathrm{9}}+\mathrm{50}}−\sqrt[{\mathrm{3}}]{\frac{\sqrt{\mathrm{202503}}}{\mathrm{9}}−\mathrm{50}}\: \\ $$$$\:\:\:\:\:\:\:\:\approx\mathrm{4}.\mathrm{56978} \\ $$$$\Rightarrow\left({a},{b},{c},{d}\right)=\left(\mathrm{4}.\mathrm{56978},\mathrm{4}.\mathrm{56978},\mathrm{4}.\mathrm{56978},\mathrm{4}.\mathrm{56978}\right)\: \\ $$$$ \\ $$$$\boldsymbol{{case}}\:\mathrm{2}:\:\boldsymbol{{two}}\:\boldsymbol{{roots}}\:\boldsymbol{{are}}\:\boldsymbol{{different}}, \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\boldsymbol{{a}}\neq\boldsymbol{{b}}=\boldsymbol{{c}}=\boldsymbol{{d}}\:\left({as}\:{example}\right) \\ $$$${a}+{b}^{\mathrm{3}} =\mathrm{100}\:\:\:...\left({i}\right) \\ $$$${b}+{ab}^{\mathrm{2}} =\mathrm{100}\:\:\:...\left({ii}\right) \\ $$$$\left({i}\right)−\left({ii}\right): \\ $$$$\left({a}−{b}\right)\left(\mathrm{1}−{b}^{\mathrm{2}} \right)=\mathrm{0} \\ $$$$\Rightarrow\mathrm{1}−{b}^{\mathrm{2}} =\mathrm{0}\: \\ $$$$\Rightarrow{b}=\pm\mathrm{1}\:\Rightarrow{a}=\mathrm{100}−{b}^{\mathrm{3}} =\mathrm{100}\mp\mathrm{1} \\ $$$${i}.{e}.\:{b}=\mathrm{1},\:{a}=\mathrm{99}\:{or}\:{b}=−\mathrm{1},\:{a}=\mathrm{101} \\ $$$$\Rightarrow\left({a},{b},{c},{d}\right)=\left(\mathrm{99},\mathrm{1},\mathrm{1},\mathrm{1}\right)\:{or}\:\left(\mathrm{101},−\mathrm{1},−\mathrm{1},−\mathrm{1}\right) \\ $$$${and}\:{we}\:{can}\:{exchange}\:{a}\:{with}\:{b},{c},{d}. \\ $$$$ \\ $$$$\boldsymbol{{case}}\:\mathrm{3}:\:\boldsymbol{{two}}\:\boldsymbol{{roots}}\:\boldsymbol{{are}}\:\boldsymbol{{different}}, \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\boldsymbol{{a}}=\boldsymbol{{b}}\neq\boldsymbol{{c}}=\boldsymbol{{d}}\:\left({as}\:{example}\right) \\ $$$${a}+{ac}^{\mathrm{2}} =\mathrm{100}\:\:\:...\left({iii}\right) \\ $$$${c}+{a}^{\mathrm{2}} {c}=\mathrm{100}\:\:\:...\left({iv}\right) \\ $$$$\left({iii}\right)−\left({iv}\right): \\ $$$$\left({a}−{c}\right)\left(\mathrm{1}−{ac}\right)=\mathrm{0} \\ $$$$\Rightarrow\mathrm{1}−{ac}=\mathrm{0} \\ $$$$\Rightarrow{ac}=\mathrm{1}\:\Rightarrow{a}+{c}=\mathrm{100} \\ $$$$\Rightarrow{a},{c}\:{are}\:{roots}\:{of}\:{z}^{\mathrm{2}} −\mathrm{100}{z}+\mathrm{1}=\mathrm{0} \\ $$$${a},{c}=\mathrm{50}\pm\sqrt{\mathrm{50}^{\mathrm{2}} −\mathrm{1}}=\mathrm{50}\pm\mathrm{7}\sqrt{\mathrm{51}} \\ $$$$\Rightarrow\left({a},{b},{c},{d}\right)=\left(\mathrm{50}+\mathrm{7}\sqrt{\mathrm{51}},\mathrm{50}+\mathrm{7}\sqrt{\mathrm{51}},\mathrm{50}−\mathrm{7}\sqrt{\mathrm{51}},\mathrm{50}−\mathrm{7}\sqrt{\mathrm{51}}\right)\:{or}\:\left(\mathrm{50}−\mathrm{7}\sqrt{\mathrm{51}},\mathrm{50}−\mathrm{7}\sqrt{\mathrm{51}},\mathrm{50}+\mathrm{7}\sqrt{\mathrm{51}},\mathrm{50}+\mathrm{7}\sqrt{\mathrm{51}}\right) \\ $$$${and}\:{we}\:{can}\:{exchange}\:{a},\:{c}\:{with}\:{a},{b}\:{or}\:{a},{d}. \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com