
Question Number 118338 by benjo_mathlover last updated on 17/Oct/20
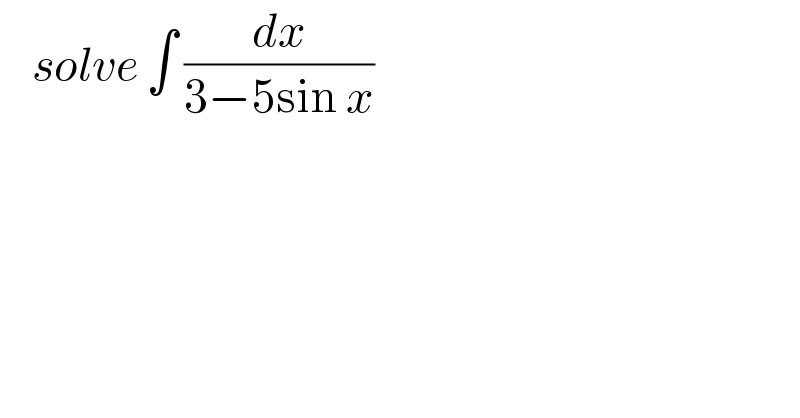
$$\:\:\:\:{solve}\:\int\:\frac{{dx}}{\mathrm{3}−\mathrm{5sin}\:{x}}\: \\ $$
Answered by Dwaipayan Shikari last updated on 17/Oct/20

$$\int\frac{{dx}}{\mathrm{3}−\mathrm{5}{sinx}} \\ $$$$=\mathrm{2}\int\frac{{dt}}{\mathrm{3}−\frac{\mathrm{10}{t}}{\left(\mathrm{1}+{t}^{\mathrm{2}} \right)}}.\frac{\mathrm{1}}{\mathrm{1}+{t}^{\mathrm{2}} }\:\:\:\:\:\:\:\:\:\:\:\:\:\:{t}={tan}\frac{{x}}{\mathrm{2}} \\ $$$$=\mathrm{2}\int\frac{{dt}}{\mathrm{3}+\mathrm{3}{t}^{\mathrm{2}} −\mathrm{10}{t}}=\frac{\mathrm{2}}{\mathrm{3}}\int\frac{{dt}}{\left({t}−\frac{\mathrm{5}}{\mathrm{3}}\right)^{\mathrm{2}} +\mathrm{1}−\frac{\mathrm{25}}{\mathrm{9}}} \\ $$$$=\frac{\mathrm{2}}{\mathrm{3}}\int\frac{{dt}}{\left({t}−\frac{\mathrm{5}}{\mathrm{3}}\right)^{\mathrm{2}} −\left(\frac{\mathrm{4}}{\mathrm{3}}\right)^{\mathrm{2}} } \\ $$$$=\frac{\mathrm{1}}{\mathrm{4}}{log}\left(\frac{{t}−\frac{\mathrm{5}}{\mathrm{3}}−\frac{\mathrm{4}}{\mathrm{3}}}{{t}−\frac{\mathrm{5}}{\mathrm{3}}+\frac{\mathrm{4}}{\mathrm{3}}}\right)=\frac{\mathrm{1}}{\mathrm{4}}{log}\left(\frac{\mathrm{3}{t}−\mathrm{9}}{\mathrm{3}{t}−\mathrm{1}}\right)=\frac{\mathrm{1}}{\mathrm{4}}{log}\left(\frac{\mathrm{3}{tan}\frac{{x}}{\mathrm{2}}−\mathrm{9}}{\mathrm{3}{tan}\frac{{x}}{\mathrm{2}}−\mathrm{1}}\right)+\mathrm{4} \\ $$
Commented by som(math1967) last updated on 17/Oct/20

$$\mathrm{Are}\:\mathrm{you}\:\mathrm{ex}−\mathrm{student}\:\mathrm{of} \\ $$$$\mathrm{Baranagar}\:\mathrm{R}.\mathrm{K}.\mathrm{Mission} \\ $$
Commented by Dwaipayan Shikari last updated on 17/Oct/20

$${No}\:{sir}.\:{I}\:{am}\:{currently}\:{studying}\:{on}\:{Jadavpur}\:{Vidyapith} \\ $$
Commented by som(math1967) last updated on 17/Oct/20

$$\mathrm{Ok} \\ $$
Commented by Dwaipayan Shikari last updated on 17/Oct/20
$${Are}\:{you}\:{from}\:{Bengal}\:{sir}? \\ $$
Commented by som(math1967) last updated on 17/Oct/20
$$\mathrm{yes}\:,\mathrm{kolkata}\:\mathrm{dunlop} \\ $$
Answered by som(math1967) last updated on 17/Oct/20

$$\int\frac{\mathrm{sec}^{\mathrm{2}} \frac{\mathrm{x}}{\mathrm{2}}\mathrm{dx}}{\mathrm{3sec}^{\mathrm{2}} \frac{\mathrm{x}}{\mathrm{2}}−\mathrm{5}×\mathrm{2sin}\frac{\mathrm{x}}{\mathrm{2}}\mathrm{cos}\frac{\mathrm{x}}{\mathrm{2}}\mathrm{sec}^{\mathrm{2}} \frac{\mathrm{x}}{\mathrm{2}}} \\ $$$$\int\frac{\mathrm{sec}^{\mathrm{2}} \frac{\mathrm{x}}{\mathrm{2}}\mathrm{dx}}{\mathrm{3}+\mathrm{3tan}^{\mathrm{2}} \frac{\mathrm{x}}{\mathrm{2}}−\mathrm{10tan}\frac{\mathrm{x}}{\mathrm{2}}} \\ $$$$\mathrm{let}\:\mathrm{tan}\frac{\mathrm{x}}{\mathrm{2}}=\mathrm{z} \\ $$$$\mathrm{sec}^{\mathrm{2}} \frac{\mathrm{x}}{\mathrm{2}}×\frac{\mathrm{1}}{\mathrm{2}}\mathrm{dx}=\mathrm{dz} \\ $$$$\mathrm{sec}^{\mathrm{2}} \frac{\mathrm{x}}{\mathrm{2}}\mathrm{dx}=\mathrm{2dz} \\ $$$$\mathrm{2}\int\frac{\mathrm{dz}}{\mathrm{3z}^{\mathrm{2}} −\mathrm{10z}+\mathrm{3}} \\ $$$$\frac{\mathrm{2}}{\mathrm{3}}\int\frac{\mathrm{dz}}{\mathrm{z}^{\mathrm{2}} −\frac{\mathrm{10z}}{\mathrm{3}}+\mathrm{1}} \\ $$$$\frac{\mathrm{2}}{\mathrm{3}}\int\frac{\mathrm{dz}}{\mathrm{z}^{\mathrm{2}} −\mathrm{2}.\mathrm{z}.\frac{\mathrm{5}}{\mathrm{3}}+\frac{\mathrm{25}}{\mathrm{9}}+\mathrm{1}−\frac{\mathrm{25}}{\mathrm{9}}} \\ $$$$\frac{\mathrm{2}}{\mathrm{3}}\int\frac{\mathrm{dz}}{\left(\mathrm{z}−\frac{\mathrm{5}}{\mathrm{3}}\right)^{\mathrm{2}} −\left(\frac{\mathrm{4}}{\mathrm{3}}\right)^{\mathrm{2}} } \\ $$$$\frac{\mathrm{2}}{\mathrm{3}}×\frac{\mathrm{3}}{\mathrm{2}×\mathrm{4}\:}\mathrm{ln}\mid\frac{\mathrm{z}−\frac{\mathrm{5}}{\mathrm{3}}−\frac{\mathrm{4}}{\mathrm{3}}}{\mathrm{z}−\frac{\mathrm{5}}{\mathrm{3}}+\frac{\mathrm{4}}{\mathrm{3}}}\mid+\mathrm{c} \\ $$$$\frac{\mathrm{1}}{\mathrm{4}}\mathrm{ln}\mid\frac{\mathrm{z}−\frac{\mathrm{9}}{\mathrm{3}}}{\mathrm{z}−\frac{\mathrm{1}}{\mathrm{3}}}\mid+\mathrm{c}\:\:\mathrm{ans} \\ $$$$\mathrm{z}=\mathrm{tan}\frac{\mathrm{x}}{\mathrm{2}} \\ $$
Answered by 1549442205PVT last updated on 17/Oct/20
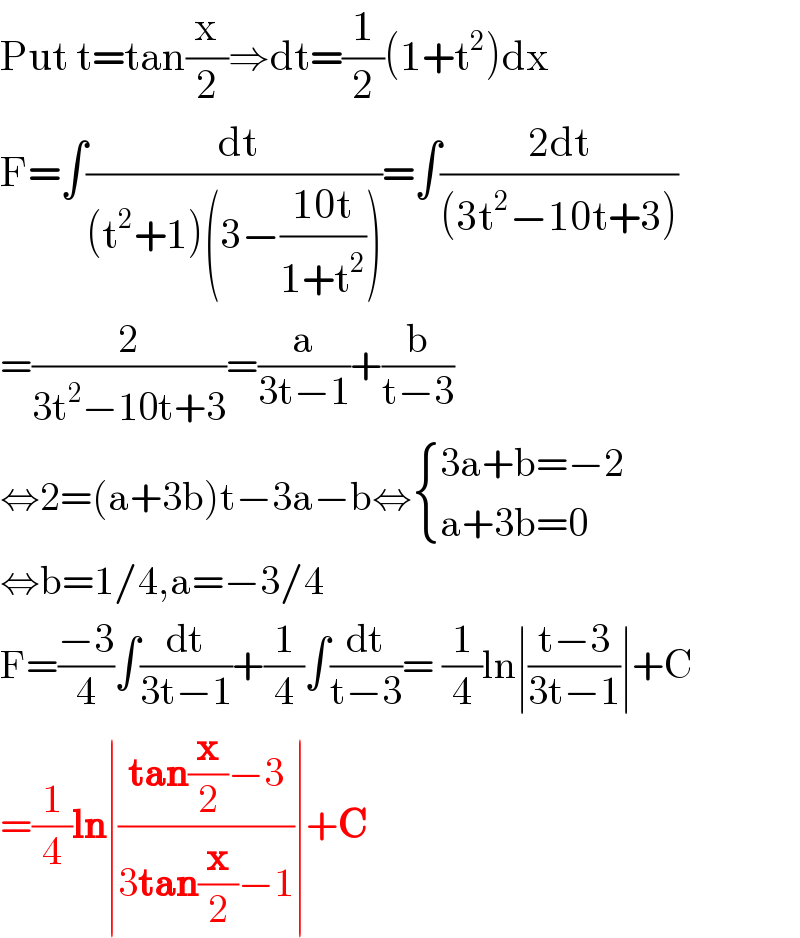
$$\mathrm{Put}\:\mathrm{t}=\mathrm{tan}\frac{\mathrm{x}}{\mathrm{2}}\Rightarrow\mathrm{dt}=\frac{\mathrm{1}}{\mathrm{2}}\left(\mathrm{1}+\mathrm{t}^{\mathrm{2}} \right)\mathrm{dx} \\ $$$$\mathrm{F}=\int\frac{\:\mathrm{dt}}{\left(\mathrm{t}^{\mathrm{2}} +\mathrm{1}\right)\left(\mathrm{3}−\frac{\mathrm{10t}}{\mathrm{1}+\mathrm{t}^{\mathrm{2}} }\right)}=\int\frac{\mathrm{2dt}}{\left(\mathrm{3t}^{\mathrm{2}} −\mathrm{10t}+\mathrm{3}\right)} \\ $$$$=\frac{\mathrm{2}}{\mathrm{3t}^{\mathrm{2}} −\mathrm{10t}+\mathrm{3}}=\frac{\mathrm{a}}{\mathrm{3t}−\mathrm{1}}+\frac{\mathrm{b}}{\mathrm{t}−\mathrm{3}} \\ $$$$\Leftrightarrow\mathrm{2}=\left(\mathrm{a}+\mathrm{3b}\right)\mathrm{t}−\mathrm{3a}−\mathrm{b}\Leftrightarrow\begin{cases}{\mathrm{3a}+\mathrm{b}=−\mathrm{2}}\\{\mathrm{a}+\mathrm{3b}=\mathrm{0}}\end{cases} \\ $$$$\Leftrightarrow\mathrm{b}=\mathrm{1}/\mathrm{4},\mathrm{a}=−\mathrm{3}/\mathrm{4} \\ $$$$\mathrm{F}=\frac{−\mathrm{3}}{\mathrm{4}}\int\frac{\mathrm{dt}}{\mathrm{3t}−\mathrm{1}}+\frac{\mathrm{1}}{\mathrm{4}}\int\frac{\mathrm{dt}}{\mathrm{t}−\mathrm{3}}=\:\frac{\mathrm{1}}{\mathrm{4}}\mathrm{ln}\mid\frac{\mathrm{t}−\mathrm{3}}{\mathrm{3t}−\mathrm{1}}\mid+\mathrm{C} \\ $$$$=\frac{\mathrm{1}}{\mathrm{4}}\boldsymbol{\mathrm{ln}}\mid\frac{\boldsymbol{\mathrm{tan}}\frac{\boldsymbol{\mathrm{x}}}{\mathrm{2}}−\mathrm{3}}{\mathrm{3}\boldsymbol{\mathrm{tan}}\frac{\boldsymbol{\mathrm{x}}}{\mathrm{2}}−\mathrm{1}}\mid+\boldsymbol{\mathrm{C}} \\ $$
