
Question Number 172020 by Mikenice last updated on 23/Jun/22

$${solve}: \\ $$$$\mathrm{2}^{{x}} =\mathrm{10}{x} \\ $$
Answered by mr W last updated on 23/Jun/22
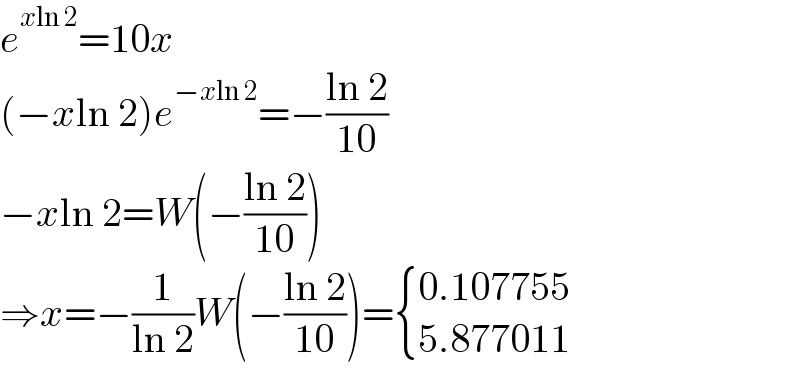
$${e}^{{x}\mathrm{ln}\:\mathrm{2}} =\mathrm{10}{x} \\ $$$$\left(−{x}\mathrm{ln}\:\mathrm{2}\right){e}^{−{x}\mathrm{ln}\:\mathrm{2}} =−\frac{\mathrm{ln}\:\mathrm{2}}{\mathrm{10}} \\ $$$$−{x}\mathrm{ln}\:\mathrm{2}={W}\left(−\frac{\mathrm{ln}\:\mathrm{2}}{\mathrm{10}}\right) \\ $$$$\Rightarrow{x}=−\frac{\mathrm{1}}{\mathrm{ln}\:\mathrm{2}}{W}\left(−\frac{\mathrm{ln}\:\mathrm{2}}{\mathrm{10}}\right)=\begin{cases}{\mathrm{0}.\mathrm{107755}}\\{\mathrm{5}.\mathrm{877011}}\end{cases} \\ $$
