
Question Number 196913 by ERLY last updated on 02/Sep/23
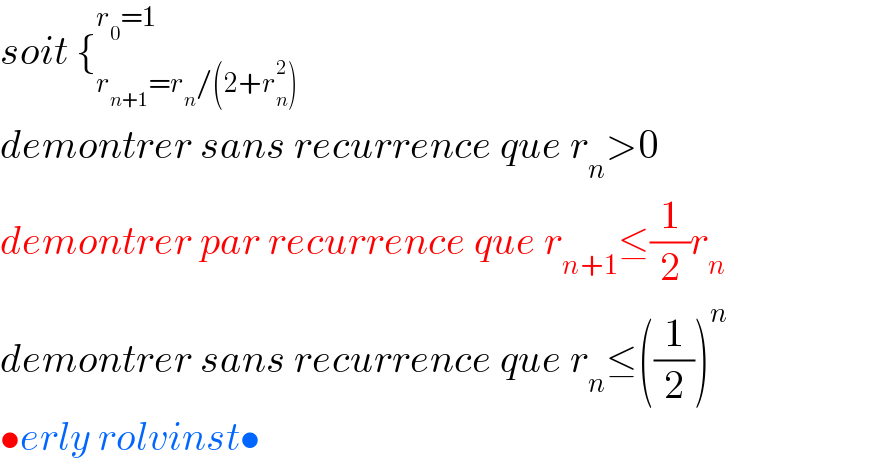
$${soit}\:\left\{_{{r}_{{n}+\mathrm{1}} ={r}_{{n}} /\left(\mathrm{2}+{r}_{{n}} ^{\mathrm{2}} \right)} ^{{r}_{\mathrm{0}} =\mathrm{1}} \right. \\ $$$${demontrer}\:{sans}\:{recurrence}\:{que}\:{r}_{{n}} >\mathrm{0} \\ $$$${demontrer}\:{par}\:{recurrence}\:{que}\:{r}_{{n}+\mathrm{1}} \leq\frac{\mathrm{1}}{\mathrm{2}}{r}_{{n}} \\ $$$${demontrer}\:{sans}\:{recurrence}\:{que}\:{r}_{{n}} \leq\left(\frac{\mathrm{1}}{\mathrm{2}}\right)^{{n}} \\ $$$$\bullet{erly}\:{rolvinst}\bullet \\ $$
Answered by aleks041103 last updated on 03/Oct/23
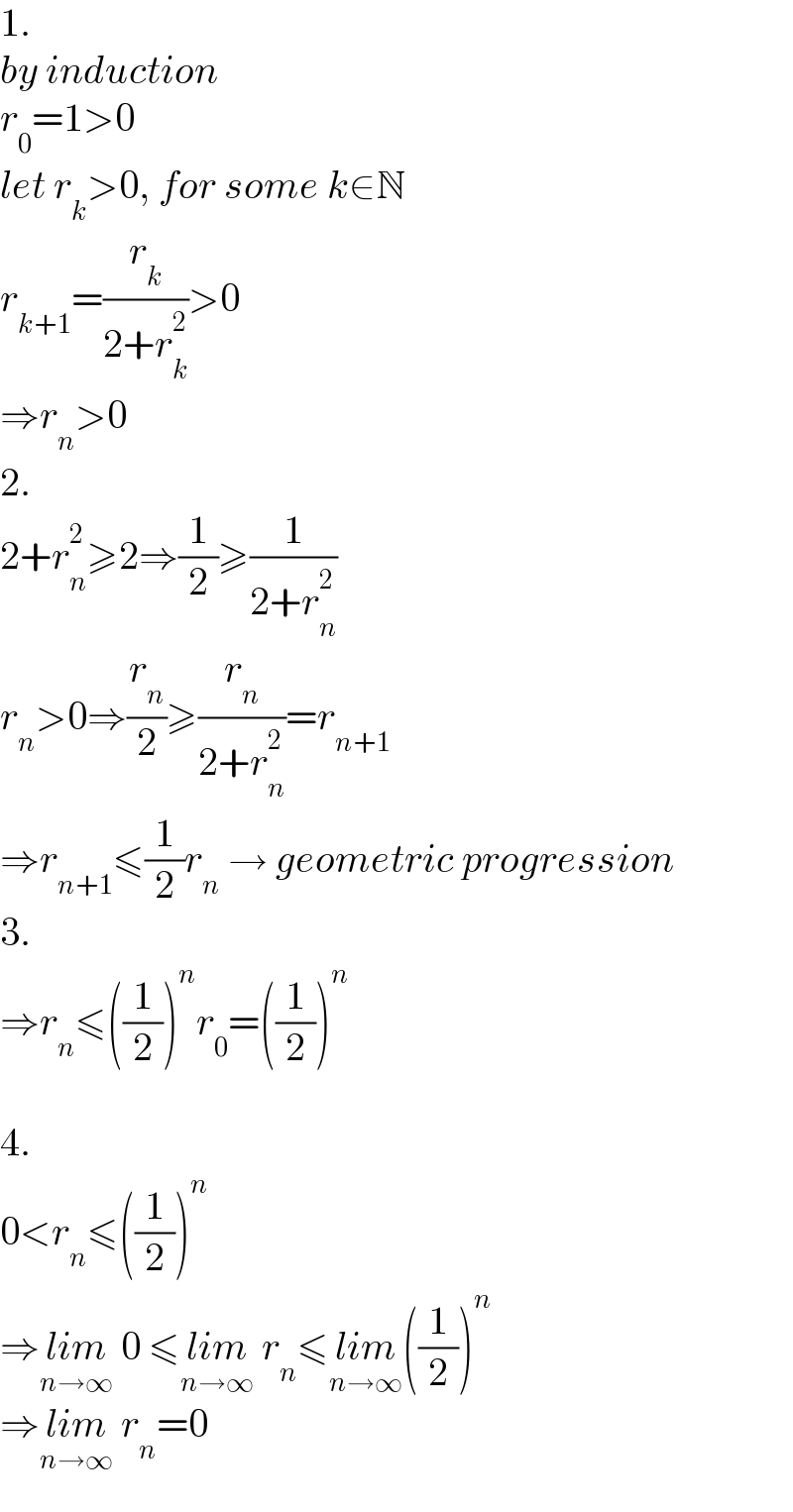
$$\mathrm{1}. \\ $$$${by}\:{induction} \\ $$$${r}_{\mathrm{0}} =\mathrm{1}>\mathrm{0} \\ $$$${let}\:{r}_{{k}} >\mathrm{0},\:{for}\:{some}\:{k}\in\mathbb{N} \\ $$$${r}_{{k}+\mathrm{1}} =\frac{{r}_{{k}} }{\mathrm{2}+{r}_{{k}} ^{\mathrm{2}} }>\mathrm{0} \\ $$$$\Rightarrow{r}_{{n}} >\mathrm{0} \\ $$$$\mathrm{2}. \\ $$$$\mathrm{2}+{r}_{{n}} ^{\mathrm{2}} \geqslant\mathrm{2}\Rightarrow\frac{\mathrm{1}}{\mathrm{2}}\geqslant\frac{\mathrm{1}}{\mathrm{2}+{r}_{{n}} ^{\mathrm{2}} } \\ $$$${r}_{{n}} >\mathrm{0}\Rightarrow\frac{{r}_{{n}} }{\mathrm{2}}\geqslant\frac{{r}_{{n}} }{\mathrm{2}+{r}_{{n}} ^{\mathrm{2}} }={r}_{{n}+\mathrm{1}} \\ $$$$\Rightarrow{r}_{{n}+\mathrm{1}} \leqslant\frac{\mathrm{1}}{\mathrm{2}}{r}_{{n}} \:\rightarrow\:{geometric}\:{progression} \\ $$$$\mathrm{3}. \\ $$$$\Rightarrow{r}_{{n}} \leqslant\left(\frac{\mathrm{1}}{\mathrm{2}}\right)^{{n}} {r}_{\mathrm{0}} =\left(\frac{\mathrm{1}}{\mathrm{2}}\right)^{{n}} \\ $$$$ \\ $$$$\mathrm{4}. \\ $$$$\mathrm{0}<{r}_{{n}} \leqslant\left(\frac{\mathrm{1}}{\mathrm{2}}\right)^{{n}} \\ $$$$\Rightarrow\underset{{n}\rightarrow\infty} {{lim}}\:\mathrm{0}\:\leqslant\underset{{n}\rightarrow\infty} {{lim}}\:{r}_{{n}} \leqslant\underset{{n}\rightarrow\infty} {{lim}}\left(\frac{\mathrm{1}}{\mathrm{2}}\right)^{{n}} \\ $$$$\Rightarrow\underset{{n}\rightarrow\infty} {{lim}}\:{r}_{{n}} =\mathrm{0} \\ $$
