
Question Number 178632 by Best1 last updated on 19/Oct/22

$${show}\:{that}\:{range}\:{of}\:{the}\:{ff}\:{projection} \\ $$$$\:{obtained}\:{by}\:{algebric}\:{expression}\: \\ $$$${R}=\left({ucos}\theta\right)\left({usin}\theta\right)+\sqrt{\left({usin}\theta\right)^{\mathrm{2}} +\mathrm{2}{gh}} \\ $$
Commented by Best1 last updated on 19/Oct/22

$${please}\:{help}\:{me} \\ $$
Commented by mr W last updated on 19/Oct/22

$${what}\:{you}\:{gave}\:{is}\:{wrong}.\:{Ar}\:{Brandon} \\ $$$${sir}\:{has}\:{given}\:{you}\:{the}\:{correct}\:{answer} \\ $$$${and}\:{corresponding}\:{working}. \\ $$
Commented by Best1 last updated on 19/Oct/22

$${i}\:{corrected}\:{it}\:{sir}\: \\ $$
Commented by mr W last updated on 19/Oct/22

$${the}\:{horizontal}\:{range}\:{is} \\ $$$${R}=\frac{{u}\:\mathrm{cos}\:\theta}{{g}}\left({u}\:\mathrm{sin}\:\theta+\sqrt{{u}^{\mathrm{2}} \:\mathrm{sin}^{\mathrm{2}} \:\theta+\mathrm{2}{gh}}\right) \\ $$$${with}\:{h}\geqslant\mathrm{0}. \\ $$
Commented by Best1 last updated on 19/Oct/22

$${please}\:{show}\:{me}\:{the}\:{step} \\ $$
Commented by Best1 last updated on 19/Oct/22

$$ \\ $$
Commented by mr W last updated on 19/Oct/22
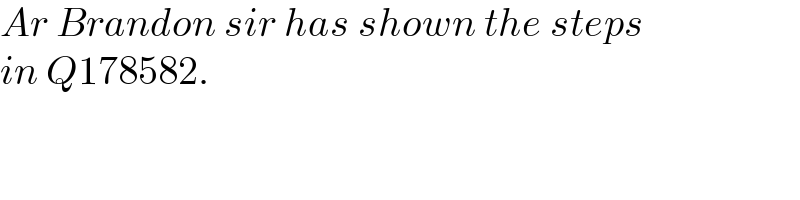
$${Ar}\:{Brandon}\:{sir}\:{has}\:{shown}\:{the}\:{steps} \\ $$$${in}\:{Q}\mathrm{178582}. \\ $$
