
Question Number 192270 by universe last updated on 13/May/23
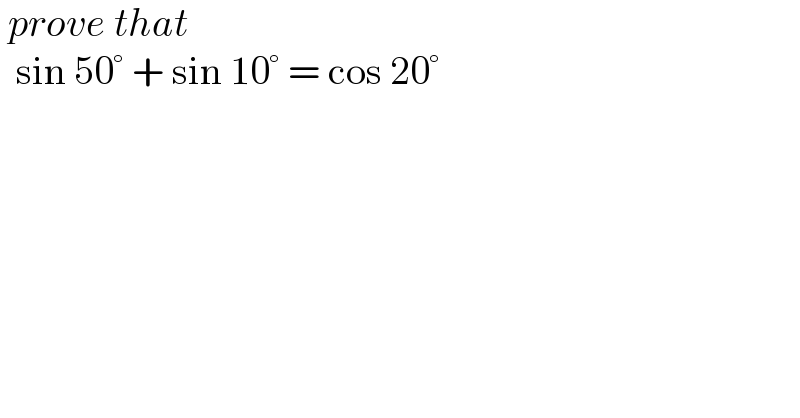
$$\:{prove}\:{that} \\ $$$$\:\:\mathrm{sin}\:\mathrm{50}°\:+\:\mathrm{sin}\:\mathrm{10}°\:=\:\mathrm{cos}\:\mathrm{20}° \\ $$
Answered by Frix last updated on 13/May/23
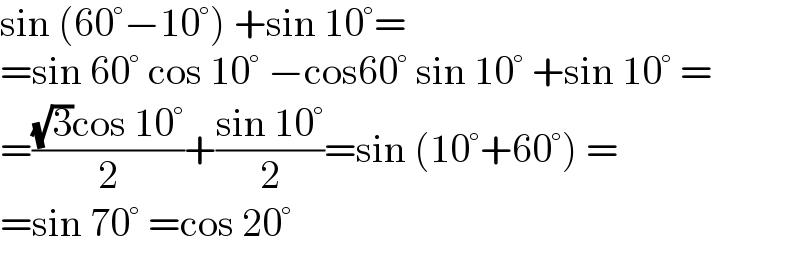
$$\mathrm{sin}\:\left(\mathrm{60}°−\mathrm{10}°\right)\:+\mathrm{sin}\:\mathrm{10}°= \\ $$$$=\mathrm{sin}\:\mathrm{60}°\:\mathrm{cos}\:\mathrm{10}°\:−\mathrm{cos60}°\:\mathrm{sin}\:\mathrm{10}°\:+\mathrm{sin}\:\mathrm{10}°\:= \\ $$$$=\frac{\sqrt{\mathrm{3}}\mathrm{cos}\:\mathrm{10}°}{\mathrm{2}}+\frac{\mathrm{sin}\:\mathrm{10}°}{\mathrm{2}}=\mathrm{sin}\:\left(\mathrm{10}°+\mathrm{60}°\right)\:= \\ $$$$=\mathrm{sin}\:\mathrm{70}°\:=\mathrm{cos}\:\mathrm{20}° \\ $$
Answered by mehdee42 last updated on 13/May/23
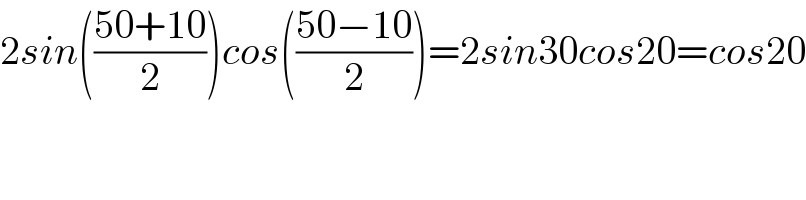
$$\mathrm{2}{sin}\left(\frac{\mathrm{50}+\mathrm{10}}{\mathrm{2}}\right){cos}\left(\frac{\mathrm{50}−\mathrm{10}}{\mathrm{2}}\right)=\mathrm{2}{sin}\mathrm{30}{cos}\mathrm{20}={cos}\mathrm{20} \\ $$
