Question and Answers Forum

All Questions      Topic List

Differentiation Questions

Previous in All Question      Next in All Question      

Previous in Differentiation      Next in Differentiation      

Question Number 139457 by mnjuly1970 last updated on 27/Apr/21

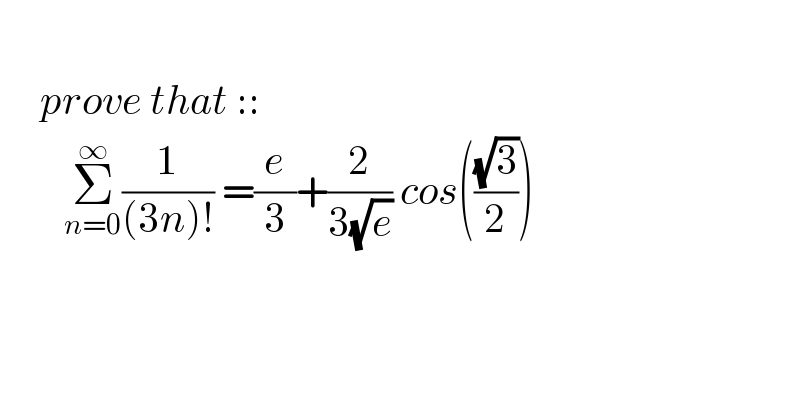
_ prove that :: Σ_(n=0) ^∞ (1/((3n)!)) =(e/3)+(2/(3(√e))) cos(((√3)/2))

$$\:_{} \:\:\: \\ $$$$\:\:\:\:\:{prove}\:{that}\::: \\ $$$$\:\:\:\:\:\:\:\:\underset{{n}=\mathrm{0}} {\overset{\infty} {\sum}}\frac{\mathrm{1}}{\left(\mathrm{3}{n}\right)!}\:=\frac{{e}}{\mathrm{3}}+\frac{\mathrm{2}}{\mathrm{3}\sqrt{{e}}}\:{cos}\left(\frac{\sqrt{\mathrm{3}}}{\mathrm{2}}\right) \\ $$$$ \\ $$

Answered by Dwaipayan Shikari last updated on 27/Apr/21

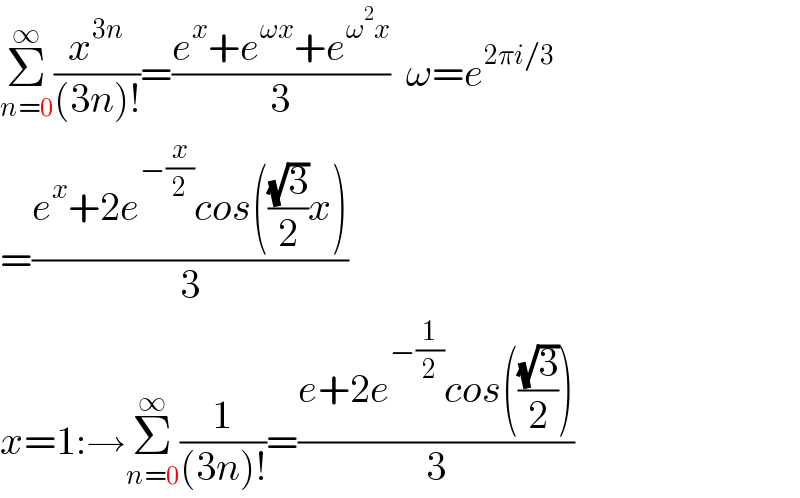
Σ_(n=0) ^∞ (x^(3n) /((3n)!))=((e^x +e^(ωx) +e^(ω^2 x) )/3) ω=e^(2πi/3) =((e^x +2e^(−(x/2)) cos(((√3)/2)x))/3) x=1:→Σ_(n=0) ^∞ (1/((3n)!))=((e+2e^(−(1/2)) cos(((√3)/2)))/3)

$$\underset{{n}=\mathrm{0}} {\overset{\infty} {\sum}}\frac{{x}^{\mathrm{3}{n}} }{\left(\mathrm{3}{n}\right)!}=\frac{{e}^{{x}} +{e}^{\omega{x}} +{e}^{\omega^{\mathrm{2}} {x}} }{\mathrm{3}}\:\:\omega={e}^{\mathrm{2}\pi{i}/\mathrm{3}} \\ $$$$=\frac{{e}^{{x}} +\mathrm{2}{e}^{−\frac{{x}}{\mathrm{2}}} {cos}\left(\frac{\sqrt{\mathrm{3}}}{\mathrm{2}}{x}\right)}{\mathrm{3}}\: \\ $$$${x}=\mathrm{1}:\rightarrow\underset{{n}=\mathrm{0}} {\overset{\infty} {\sum}}\frac{\mathrm{1}}{\left(\mathrm{3}{n}\right)!}=\frac{{e}+\mathrm{2}{e}^{−\frac{\mathrm{1}}{\mathrm{2}}} {cos}\left(\frac{\sqrt{\mathrm{3}}}{\mathrm{2}}\right)}{\mathrm{3}} \\ $$

Commented by Dwaipayan Shikari last updated on 27/Apr/21

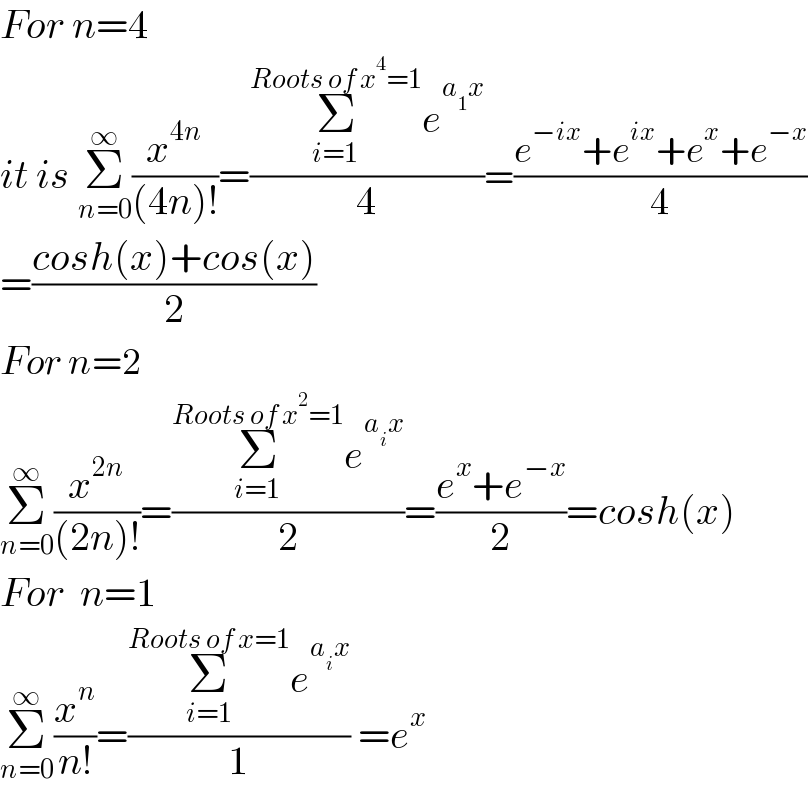
For n=4 it is Σ_(n=0) ^∞ (x^(4n) /((4n)!))=((Σ_(i=1) ^(Roots of x^4 =1) e^(a_1 x) )/4)=((e^(−ix) +e^(ix) +e^x +e^(−x) )/4) =((cosh(x)+cos(x))/2) For n=2 Σ_(n=0) ^∞ (x^(2n) /((2n)!))=((Σ_(i=1) ^(Roots of x^2 =1) e^(a_i x) )/2)=((e^x +e^(−x) )/2)=cosh(x) For n=1 Σ_(n=0) ^∞ (x^n /(n!))=((Σ_(i=1) ^(Roots of x=1) e^(a_i x) )/1) =e^x

$${For}\:{n}=\mathrm{4} \\ $$$${it}\:{is}\:\underset{{n}=\mathrm{0}} {\overset{\infty} {\sum}}\frac{{x}^{\mathrm{4}{n}} }{\left(\mathrm{4}{n}\right)!}=\frac{\underset{{i}=\mathrm{1}} {\overset{{Roots}\:{of}\:{x}^{\mathrm{4}} =\mathrm{1}} {\sum}}{e}^{{a}_{\mathrm{1}} {x}} }{\mathrm{4}}=\frac{{e}^{−{ix}} +{e}^{{ix}} +{e}^{{x}} +{e}^{−{x}} }{\mathrm{4}} \\ $$$$=\frac{{cosh}\left({x}\right)+{cos}\left({x}\right)}{\mathrm{2}} \\ $$$${For}\:{n}=\mathrm{2} \\ $$$$\underset{{n}=\mathrm{0}} {\overset{\infty} {\sum}}\frac{{x}^{\mathrm{2}{n}} }{\left(\mathrm{2}{n}\right)!}=\frac{\underset{{i}=\mathrm{1}} {\overset{{Roots}\:{of}\:{x}^{\mathrm{2}} =\mathrm{1}} {\sum}}{e}^{{a}_{{i}} {x}} }{\mathrm{2}}=\frac{{e}^{{x}} +{e}^{−{x}} }{\mathrm{2}}={cosh}\left({x}\right) \\ $$$${For}\:\:{n}=\mathrm{1} \\ $$$$\underset{{n}=\mathrm{0}} {\overset{\infty} {\sum}}\frac{{x}^{{n}} }{{n}!}=\frac{\underset{{i}=\mathrm{1}} {\overset{{Roots}\:{of}\:{x}=\mathrm{1}} {\sum}}{e}^{{a}_{{i}} {x}} }{\mathrm{1}}\:={e}^{{x}} \\ $$

Commented by mnjuly1970 last updated on 27/Apr/21

very nice...

$$\:{very}\:{nice}... \\ $$

Commented by Dwaipayan Shikari last updated on 27/Apr/21

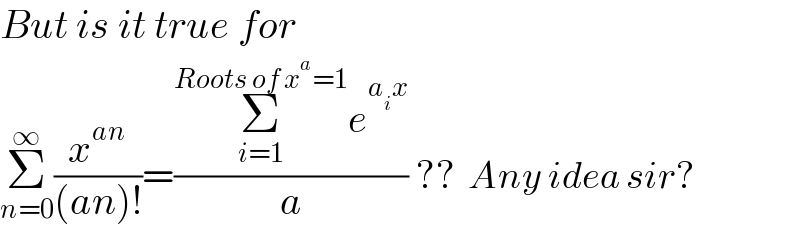
But is it true for Σ_(n=0) ^∞ (x^(an) /((an)!))=((Σ_(i=1) ^(Roots of x^a =1) e^(a_i x) )/a) ?? Any idea sir?

$${But}\:{is}\:{it}\:{true}\:{for} \\ $$$$\underset{{n}=\mathrm{0}} {\overset{\infty} {\sum}}\frac{{x}^{{an}} }{\left({an}\right)!}=\frac{\underset{{i}=\mathrm{1}} {\overset{{Roots}\:{of}\:{x}^{{a}} =\mathrm{1}} {\sum}}{e}^{{a}_{{i}} {x}} }{{a}}\:??\:\:{Any}\:{idea}\:{sir}? \\ $$

Commented by Dwaipayan Shikari last updated on 27/Apr/21

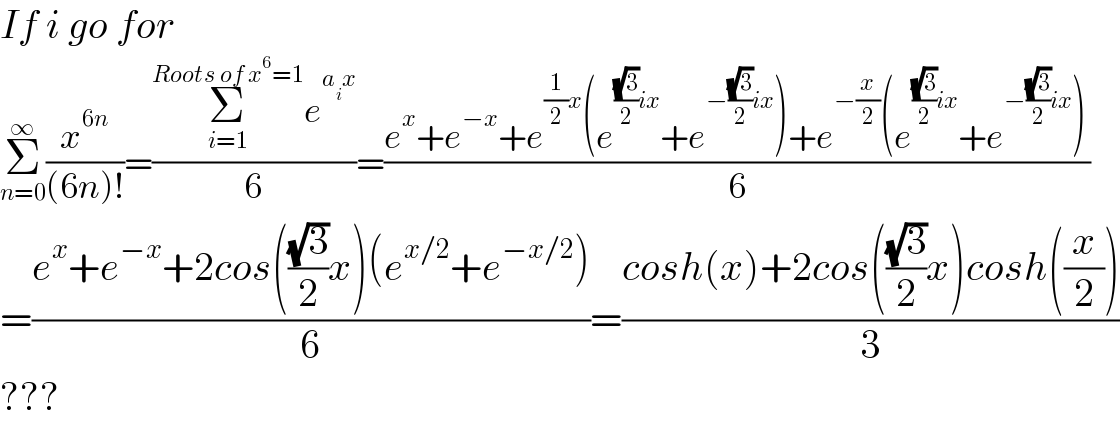
If i go for Σ_(n=0) ^∞ (x^(6n) /((6n)!))=((Σ_(i=1) ^(Roots of x^6 =1) e^(a_i x) )/6)=((e^x +e^(−x) +e^((1/2)x) (e^(((√3)/2)ix) +e^(−((√3)/2)ix) )+e^(−(x/2)) (e^(((√3)/2)ix) +e^(−((√3)/2)ix) ))/6) =((e^x +e^(−x) +2cos(((√3)/2)x)(e^(x/2) +e^(−x/2) ))/6)=((cosh(x)+2cos(((√3)/2)x)cosh((x/2)))/3) ???

$${If}\:{i}\:{go}\:{for} \\ $$$$\underset{{n}=\mathrm{0}} {\overset{\infty} {\sum}}\frac{{x}^{\mathrm{6}{n}} }{\left(\mathrm{6}{n}\right)!}=\frac{\underset{{i}=\mathrm{1}} {\overset{{Roots}\:{of}\:{x}^{\mathrm{6}} =\mathrm{1}} {\sum}}{e}^{{a}_{{i}} {x}} }{\mathrm{6}}=\frac{{e}^{{x}} +{e}^{−{x}} +{e}^{\frac{\mathrm{1}}{\mathrm{2}}{x}} \left({e}^{\frac{\sqrt{\mathrm{3}}}{\mathrm{2}}{ix}} +{e}^{−\frac{\sqrt{\mathrm{3}}}{\mathrm{2}}{ix}} \right)+{e}^{−\frac{{x}}{\mathrm{2}}} \left({e}^{\frac{\sqrt{\mathrm{3}}}{\mathrm{2}}{ix}} +{e}^{−\frac{\sqrt{\mathrm{3}}}{\mathrm{2}}{ix}} \right)}{\mathrm{6}}\: \\ $$$$=\frac{{e}^{{x}} +{e}^{−{x}} +\mathrm{2}{cos}\left(\frac{\sqrt{\mathrm{3}}}{\mathrm{2}}{x}\right)\left({e}^{{x}/\mathrm{2}} +{e}^{−{x}/\mathrm{2}} \right)}{\mathrm{6}}=\frac{{cosh}\left({x}\right)+\mathrm{2}{cos}\left(\frac{\sqrt{\mathrm{3}}}{\mathrm{2}}{x}\right){cosh}\left(\frac{{x}}{\mathrm{2}}\right)}{\mathrm{3}} \\ $$$$??? \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com