Question and Answers Forum

All Questions      Topic List

Trigonometry Questions

Previous in All Question      Next in All Question      

Previous in Trigonometry      Next in Trigonometry      

Question Number 12572 by tawa last updated on 25/Apr/17

prove that if, sin(θ) = ((1 − x)/(1 + x)) then tan((x/4) − (θ/2)) = (√x)

$$\mathrm{prove}\:\mathrm{that}\:\mathrm{if}, \\ $$$$\mathrm{sin}\left(\theta\right)\:=\:\frac{\mathrm{1}\:−\:\mathrm{x}}{\mathrm{1}\:+\:\mathrm{x}}\:\:\:\:\:\mathrm{then}\:\:\mathrm{tan}\left(\frac{\mathrm{x}}{\mathrm{4}}\:−\:\frac{\theta}{\mathrm{2}}\right)\:=\:\sqrt{\mathrm{x}} \\ $$

Commented by mrW1 last updated on 26/Apr/17

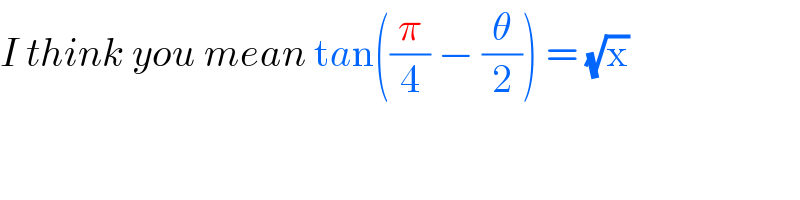
I think you mean tan((π/4) − (θ/2)) = (√x)

$${I}\:{think}\:{you}\:{mean}\:\mathrm{t}{a}\mathrm{n}\left(\frac{\pi}{\mathrm{4}}\:−\:\frac{\theta}{\mathrm{2}}\right)\:=\:\sqrt{\mathrm{x}} \\ $$

Answered by mrW1 last updated on 26/Apr/17

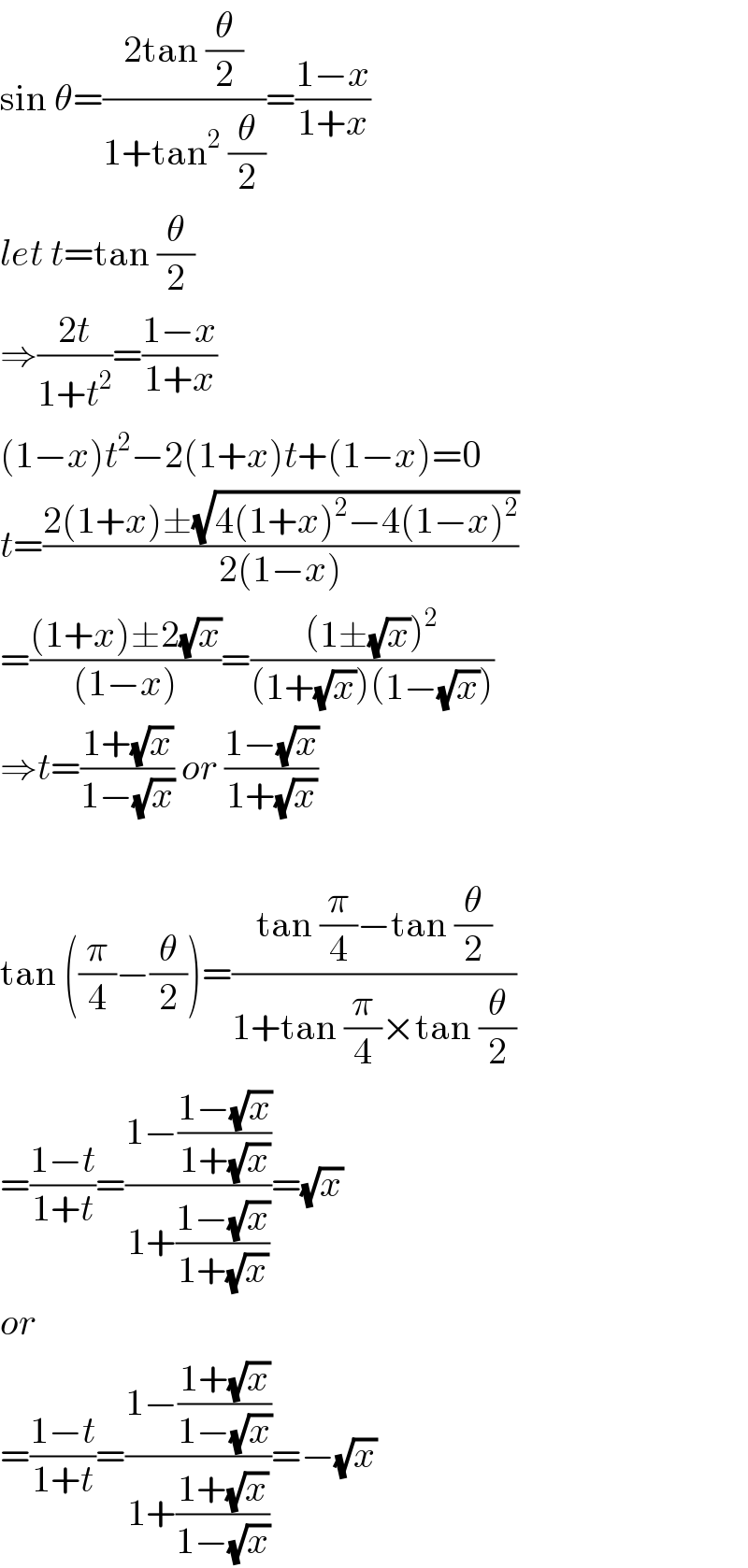
sin θ=((2tan (θ/2))/(1+tan^2 (θ/2)))=((1−x)/(1+x)) let t=tan (θ/2) ⇒((2t)/(1+t^2 ))=((1−x)/(1+x)) (1−x)t^2 −2(1+x)t+(1−x)=0 t=((2(1+x)±(√(4(1+x)^2 −4(1−x)^2 )))/(2(1−x))) =(((1+x)±2(√x))/((1−x)))=(((1±(√x))^2 )/((1+(√x))(1−(√x)))) ⇒t=((1+(√x))/(1−(√x))) or ((1−(√x))/(1+(√x))) tan ((π/4)−(θ/2))=((tan (π/4)−tan (θ/2))/(1+tan (π/4)×tan (θ/2))) =((1−t)/(1+t))=((1−((1−(√x))/(1+(√x))))/(1+((1−(√x))/(1+(√x)))))=(√x) or =((1−t)/(1+t))=((1−((1+(√x))/(1−(√x))))/(1+((1+(√x))/(1−(√x)))))=−(√x)

$$\mathrm{sin}\:\theta=\frac{\mathrm{2tan}\:\frac{\theta}{\mathrm{2}}}{\mathrm{1}+\mathrm{tan}^{\mathrm{2}} \:\frac{\theta}{\mathrm{2}}}=\frac{\mathrm{1}−{x}}{\mathrm{1}+{x}} \\ $$$${let}\:{t}=\mathrm{tan}\:\frac{\theta}{\mathrm{2}} \\ $$$$\Rightarrow\frac{\mathrm{2}{t}}{\mathrm{1}+{t}^{\mathrm{2}} }=\frac{\mathrm{1}−{x}}{\mathrm{1}+{x}} \\ $$$$\left(\mathrm{1}−{x}\right){t}^{\mathrm{2}} −\mathrm{2}\left(\mathrm{1}+{x}\right){t}+\left(\mathrm{1}−{x}\right)=\mathrm{0} \\ $$$${t}=\frac{\mathrm{2}\left(\mathrm{1}+{x}\right)\pm\sqrt{\mathrm{4}\left(\mathrm{1}+{x}\right)^{\mathrm{2}} −\mathrm{4}\left(\mathrm{1}−{x}\right)^{\mathrm{2}} }}{\mathrm{2}\left(\mathrm{1}−{x}\right)} \\ $$$$=\frac{\left(\mathrm{1}+{x}\right)\pm\mathrm{2}\sqrt{{x}}}{\left(\mathrm{1}−{x}\right)}=\frac{\left(\mathrm{1}\pm\sqrt{{x}}\right)^{\mathrm{2}} }{\left(\mathrm{1}+\sqrt{{x}}\right)\left(\mathrm{1}−\sqrt{{x}}\right)} \\ $$$$\Rightarrow{t}=\frac{\mathrm{1}+\sqrt{{x}}}{\mathrm{1}−\sqrt{{x}}}\:{or}\:\frac{\mathrm{1}−\sqrt{{x}}}{\mathrm{1}+\sqrt{{x}}} \\ $$$$ \\ $$$$\mathrm{tan}\:\left(\frac{\pi}{\mathrm{4}}−\frac{\theta}{\mathrm{2}}\right)=\frac{\mathrm{tan}\:\frac{\pi}{\mathrm{4}}−\mathrm{tan}\:\frac{\theta}{\mathrm{2}}}{\mathrm{1}+\mathrm{tan}\:\frac{\pi}{\mathrm{4}}×\mathrm{tan}\:\frac{\theta}{\mathrm{2}}} \\ $$$$=\frac{\mathrm{1}−{t}}{\mathrm{1}+{t}}=\frac{\mathrm{1}−\frac{\mathrm{1}−\sqrt{{x}}}{\mathrm{1}+\sqrt{{x}}}}{\mathrm{1}+\frac{\mathrm{1}−\sqrt{{x}}}{\mathrm{1}+\sqrt{{x}}}}=\sqrt{{x}} \\ $$$${or} \\ $$$$=\frac{\mathrm{1}−{t}}{\mathrm{1}+{t}}=\frac{\mathrm{1}−\frac{\mathrm{1}+\sqrt{{x}}}{\mathrm{1}−\sqrt{{x}}}}{\mathrm{1}+\frac{\mathrm{1}+\sqrt{{x}}}{\mathrm{1}−\sqrt{{x}}}}=−\sqrt{{x}} \\ $$

Commented by tawa last updated on 26/Apr/17

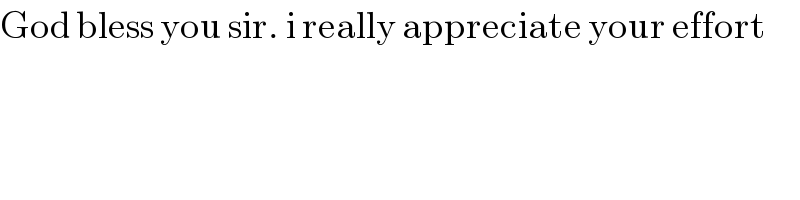
God bless you sir. i really appreciate your effort

$$\mathrm{God}\:\mathrm{bless}\:\mathrm{you}\:\mathrm{sir}.\:\mathrm{i}\:\mathrm{really}\:\mathrm{appreciate}\:\mathrm{your}\:\mathrm{effort} \\ $$

Answered by ajfour last updated on 26/Apr/17

tan ((π/4)−(θ/2))=((1−tan (θ/2))/(1+tan (θ/2))) sin θ=((2tan (θ/2))/(1+tan^2 (θ/2)))=((1−x)/(1+x)) =(p/q) (say) ((q−p)/(q+p)) =[((1−tan (θ/2))/(1+tan (θ/2)))]^2 =(((1+x)−(1−x))/((1+x)+(1−x))) ⇒ tan^2 ((π/4)−(θ/2))=((2x)/2) =x ⇒tan ((π/4)−(θ/2))=±(√x) .

$$\mathrm{tan}\:\left(\frac{\pi}{\mathrm{4}}−\frac{\theta}{\mathrm{2}}\right)=\frac{\mathrm{1}−\mathrm{tan}\:\left(\theta/\mathrm{2}\right)}{\mathrm{1}+\mathrm{tan}\:\left(\theta/\mathrm{2}\right)} \\ $$$$\:\:\mathrm{sin}\:\theta=\frac{\mathrm{2tan}\:\left(\theta/\mathrm{2}\right)}{\mathrm{1}+\mathrm{tan}\:^{\mathrm{2}} \left(\theta/\mathrm{2}\right)}=\frac{\mathrm{1}−{x}}{\mathrm{1}+{x}}\:=\frac{{p}}{{q}}\:\left({say}\right) \\ $$$$\frac{{q}−{p}}{{q}+{p}}\:=\left[\frac{\mathrm{1}−\mathrm{tan}\:\left(\theta/\mathrm{2}\right)}{\mathrm{1}+\mathrm{tan}\:\left(\theta/\mathrm{2}\right)}\right]^{\mathrm{2}} \:=\frac{\left(\mathrm{1}+{x}\right)−\left(\mathrm{1}−{x}\right)}{\left(\mathrm{1}+{x}\right)+\left(\mathrm{1}−{x}\right)} \\ $$$$\Rightarrow\:\mathrm{tan}^{\mathrm{2}} \:\left(\frac{\pi}{\mathrm{4}}−\frac{\theta}{\mathrm{2}}\right)=\frac{\mathrm{2}{x}}{\mathrm{2}}\:={x}\: \\ $$$$\Rightarrow\mathrm{tan}\:\left(\frac{\pi}{\mathrm{4}}−\frac{\theta}{\mathrm{2}}\right)=\pm\sqrt{\boldsymbol{{x}}}\:. \\ $$

Commented by mrW1 last updated on 26/Apr/17

Can you please check: In my way I get tan ((π/4)−(θ/2))=±(√x) but in your way you get only (√(x.)) For example: x=3 sin θ=((1−3)/(1+3))=−(2/4)=−(1/2) ⇒θ=((7π)/6) or −(π/6) (π/4)−(θ/2)=(π/4)−((7π)/(12))=−(π/3) or =(π/4)+(π/(12))=(π/3) tan ((π/4)−(θ/2))=tan (−(π/3))=−(√3) or tan ((π/4)−(θ/2))=tan ((π/3))=(√3) Is something wrong here?

$${Can}\:{you}\:{please}\:{check}: \\ $$$${In}\:{my}\:{way}\:{I}\:{get}\:\mathrm{tan}\:\left(\frac{\pi}{\mathrm{4}}−\frac{\theta}{\mathrm{2}}\right)=\pm\sqrt{{x}} \\ $$$${but}\:{in}\:{your}\:{way}\:{you}\:{get}\:{only}\:\sqrt{{x}.} \\ $$$$ \\ $$$${For}\:{example}: \\ $$$${x}=\mathrm{3} \\ $$$$\mathrm{sin}\:\theta=\frac{\mathrm{1}−\mathrm{3}}{\mathrm{1}+\mathrm{3}}=−\frac{\mathrm{2}}{\mathrm{4}}=−\frac{\mathrm{1}}{\mathrm{2}} \\ $$$$\Rightarrow\theta=\frac{\mathrm{7}\pi}{\mathrm{6}}\:{or}\:−\frac{\pi}{\mathrm{6}} \\ $$$$\frac{\pi}{\mathrm{4}}−\frac{\theta}{\mathrm{2}}=\frac{\pi}{\mathrm{4}}−\frac{\mathrm{7}\pi}{\mathrm{12}}=−\frac{\pi}{\mathrm{3}}\:{or}\:=\frac{\pi}{\mathrm{4}}+\frac{\pi}{\mathrm{12}}=\frac{\pi}{\mathrm{3}} \\ $$$$\mathrm{tan}\:\left(\frac{\pi}{\mathrm{4}}−\frac{\theta}{\mathrm{2}}\right)=\mathrm{tan}\:\left(−\frac{\pi}{\mathrm{3}}\right)=−\sqrt{\mathrm{3}} \\ $$$${or} \\ $$$$\mathrm{tan}\:\left(\frac{\pi}{\mathrm{4}}−\frac{\theta}{\mathrm{2}}\right)=\mathrm{tan}\:\left(\frac{\pi}{\mathrm{3}}\right)=\sqrt{\mathrm{3}} \\ $$$$ \\ $$$${Is}\:{something}\:{wrong}\:{here}? \\ $$

Commented by ajfour last updated on 26/Apr/17

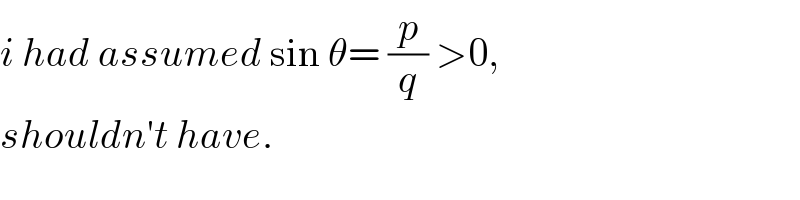
i had assumed sin θ= (p/q) >0, shouldn′t have.

$${i}\:{had}\:{assumed}\:\mathrm{sin}\:\theta=\:\frac{{p}}{{q}}\:>\mathrm{0}, \\ $$$${shouldn}'{t}\:{have}. \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com