Question and Answers Forum

All Questions      Topic List

Number Theory Questions

Previous in All Question      Next in All Question      

Previous in Number Theory      Next in Number Theory      

Question Number 217129 by ArshadS last updated on 02/Mar/25

prove that if an integer n is not divisible by 2 or 3 then n^2 ≡1(mod 24)

$${prove}\:{that}\:{if}\:{an}\:{integer}\:{n}\:{is}\:{not}\:{divisible}\:{by}\:\mathrm{2}\:{or}\:\mathrm{3} \\ $$$$\:{then}\:{n}^{\mathrm{2}} \equiv\mathrm{1}\left({mod}\:\mathrm{24}\right) \\ $$

Commented by A5T last updated on 02/Mar/25

This is not necessarily true. n could also be ≡ 5,7,11,13,17,19,23 (mod 24)

$$\mathrm{This}\:\mathrm{is}\:\mathrm{not}\:\mathrm{necessarily}\:\mathrm{true}.\: \\ $$$$\mathrm{n}\:\mathrm{could}\:\mathrm{also}\:\mathrm{be}\:\equiv\:\mathrm{5},\mathrm{7},\mathrm{11},\mathrm{13},\mathrm{17},\mathrm{19},\mathrm{23}\:\left(\mathrm{mod}\:\mathrm{24}\right) \\ $$

Commented by ArshadS last updated on 02/Mar/25

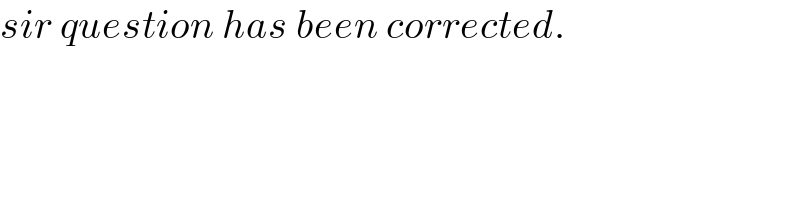
sir question has been corrected.

$${sir}\:{question}\:{has}\:{been}\:{corrected}. \\ $$

Answered by A5T last updated on 02/Mar/25

2∤n and 3∤n⇒n≡1,5,7,11,13,17,19 or 23 (mod 24) ⇒n^2 ≡ 1,25, 49,121,169,289,361 or 529(mod 24) which are all equivalent to 1 (mod 24)

$$\mathrm{2}\nmid\mathrm{n}\:\mathrm{and}\:\mathrm{3}\nmid\mathrm{n}\Rightarrow\mathrm{n}\equiv\mathrm{1},\mathrm{5},\mathrm{7},\mathrm{11},\mathrm{13},\mathrm{17},\mathrm{19}\:\mathrm{or}\:\mathrm{23}\:\left(\mathrm{mod}\:\mathrm{24}\right) \\ $$$$\Rightarrow\mathrm{n}^{\mathrm{2}} \equiv\:\mathrm{1},\mathrm{25},\:\mathrm{49},\mathrm{121},\mathrm{169},\mathrm{289},\mathrm{361}\:\mathrm{or}\:\mathrm{529}\left(\mathrm{mod}\:\mathrm{24}\right) \\ $$$$\mathrm{which}\:\mathrm{are}\:\mathrm{all}\:\mathrm{equivalent}\:\mathrm{to}\:\mathrm{1}\:\left(\mathrm{mod}\:\mathrm{24}\right)\: \\ $$

Commented by ArshadS last updated on 03/Mar/25

Nice approach!

$$\mathcal{N}{ice}\:{approach}! \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com