Question and Answers Forum

All Questions      Topic List

Differentiation Questions

Previous in All Question      Next in All Question      

Previous in Differentiation      Next in Differentiation      

Question Number 3417 by RasheedSindhi last updated on 13/Dec/15

prove that among all closed figures having same perimeter circle has maximum area.

$${prove}\:{that}\:{among}\:{all}\:{closed}\:{figures} \\ $$$${having}\:{same}\:{perimeter}\:{circle} \\ $$$${has}\:{maximum}\:{area}. \\ $$

Commented by Filup last updated on 13/Dec/15

I dont understand the question

$$\mathrm{I}\:\mathrm{dont}\:\mathrm{understand}\:\mathrm{the}\:\mathrm{question} \\ $$

Commented by RasheedSindhi last updated on 13/Dec/15

Consider closed figures triangle, quadilateral,pentagon...or other closed curves or mixture of curves and straight line segments ,all having same perimeter.Among all the areas these figures have the largest is the area of circle.

$${Consider}\:{closed}\:{figures}\:{triangle}, \\ $$$${quadilateral},{pentagon}...{or}\:{other} \\ $$$${closed}\:{curves}\:{or}\:{mixture}\:{of}\:{curves} \\ $$$${and}\:{straight}\:{line}\:{segments}\:,{all} \\ $$$${having}\:{same}\:{perimeter}.{Among} \\ $$$${all}\:{the}\:{areas}\:{these}\:{figures}\:{have} \\ $$$${the}\:{largest}\:{is}\:{the}\:{area}\:{of}\:{circle}. \\ $$

Commented by 123456 last updated on 13/Dec/15

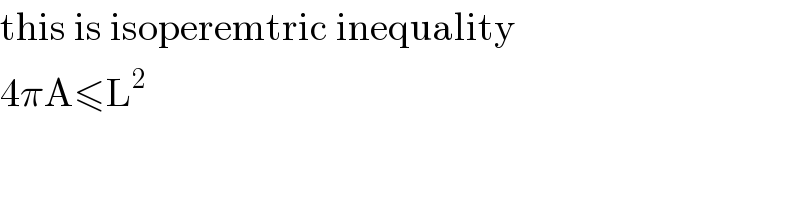
this is isoperemtric inequality 4πA≤L^2

$$\mathrm{this}\:\mathrm{is}\:\mathrm{isoperemtric}\:\mathrm{inequality} \\ $$$$\mathrm{4}\pi\mathrm{A}\leqslant\mathrm{L}^{\mathrm{2}} \\ $$

Commented by Filup last updated on 13/Dec/15

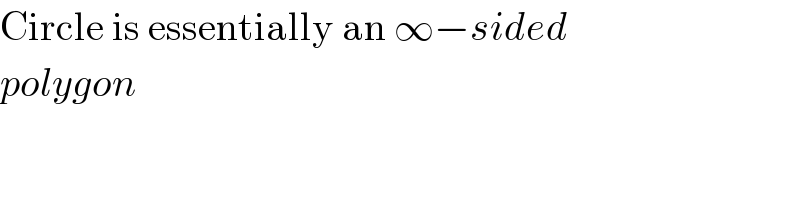
Circle is essentially an ∞−sided polygon

$$\mathrm{Circle}\:\mathrm{is}\:\mathrm{essentially}\:\mathrm{an}\:\infty−{sided} \\ $$$${polygon} \\ $$

Commented by Filup last updated on 13/Dec/15

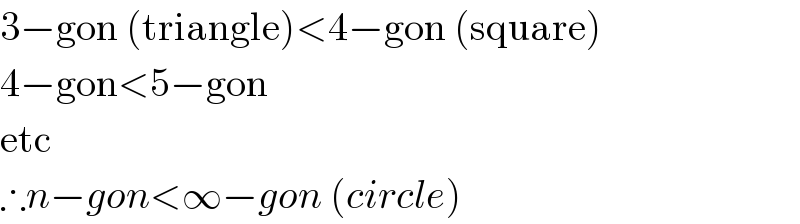
3−gon (triangle)<4−gon (square) 4−gon<5−gon etc ∴n−gon<∞−gon (circle)

$$\mathrm{3}−\mathrm{gon}\:\left(\mathrm{triangle}\right)<\mathrm{4}−\mathrm{gon}\:\left(\mathrm{square}\right) \\ $$$$\mathrm{4}−\mathrm{gon}<\mathrm{5}−\mathrm{gon} \\ $$$$\mathrm{etc} \\ $$$$\therefore{n}−{gon}<\infty−{gon}\:\left({circle}\right) \\ $$

Commented by Rasheed Soomro last updated on 13/Dec/15

What is meant by isoperemtric inequality ?

$$\mathcal{W}{hat}\:{is}\:{meant}\:{by}\:\mathrm{isoperemtric}\:\mathrm{inequality}\:? \\ $$

Commented by RasheedSindhi last updated on 13/Dec/15

Th∝nkS

$$\mathcal{T}{h}\propto{nk}\mathcal{S} \\ $$

Commented by prakash jain last updated on 13/Dec/15

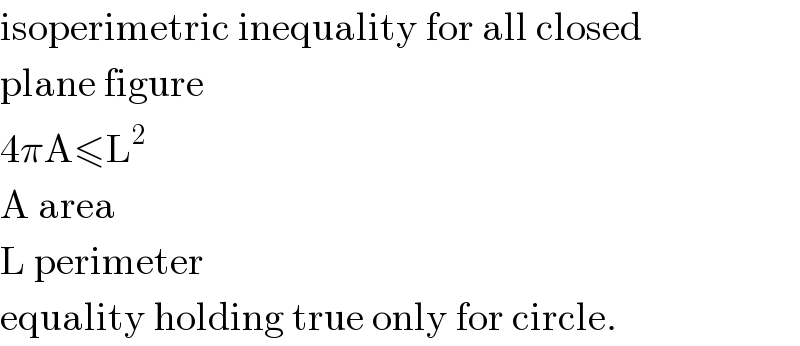
isoperimetric inequality for all closed plane figure 4πA≤L^2 A area L perimeter equality holding true only for circle.

$$\mathrm{isoperimetric}\:\mathrm{inequality}\:\mathrm{for}\:\mathrm{all}\:\mathrm{closed} \\ $$$$\mathrm{plane}\:\mathrm{figure} \\ $$$$\mathrm{4}\pi\mathrm{A}\leqslant\mathrm{L}^{\mathrm{2}} \\ $$$$\mathrm{A}\:\mathrm{area} \\ $$$$\mathrm{L}\:\mathrm{perimeter} \\ $$$$\mathrm{equality}\:\mathrm{holding}\:\mathrm{true}\:\mathrm{only}\:\mathrm{for}\:\mathrm{circle}. \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com