
Previous in Matrices and Determinants Next in Matrices and Determinants
Question Number 98003 by M±th+et+s last updated on 11/Jun/20

$${prove}\:{that}\: \\ $$$${E}={mc}^{\mathrm{2}} \:\: \\ $$
Answered by Rio Michael last updated on 11/Jun/20
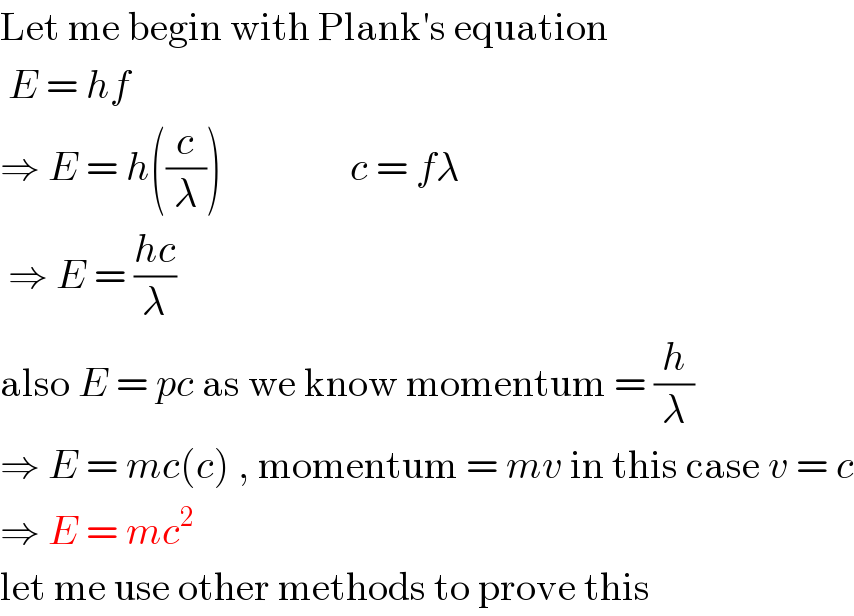
$$\mathrm{Let}\:\mathrm{me}\:\mathrm{begin}\:\mathrm{with}\:\mathrm{Plank}'\mathrm{s}\:\mathrm{equation} \\ $$$$\:{E}\:=\:{hf}\: \\ $$$$\Rightarrow\:{E}\:=\:{h}\left(\frac{{c}}{\lambda}\right)\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:{c}\:=\:{f}\lambda \\ $$$$\:\Rightarrow\:{E}\:=\:\frac{{hc}}{\lambda} \\ $$$$\mathrm{also}\:{E}\:=\:{pc}\:\mathrm{as}\:\mathrm{we}\:\mathrm{know}\:\mathrm{momentum}\:=\:\frac{{h}}{\lambda} \\ $$$$\Rightarrow\:{E}\:=\:{mc}\left({c}\right)\:,\:\mathrm{momentum}\:=\:{mv}\:\mathrm{in}\:\mathrm{this}\:\mathrm{case}\:{v}\:=\:{c} \\ $$$$\Rightarrow\:{E}\:=\:{mc}^{\mathrm{2}} \\ $$$$\mathrm{let}\:\mathrm{me}\:\mathrm{use}\:\mathrm{other}\:\mathrm{methods}\:\mathrm{to}\:\mathrm{prove}\:\mathrm{this} \\ $$
Answered by Rio Michael last updated on 11/Jun/20
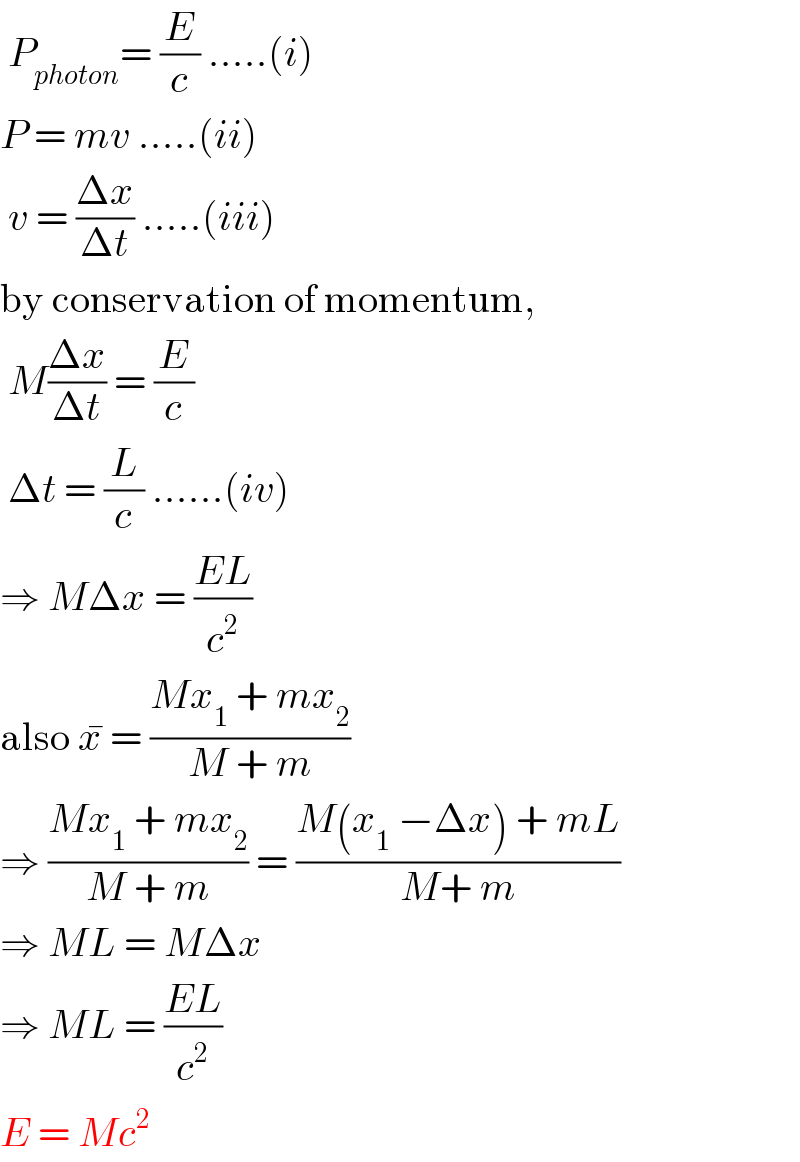
$$\:{P}_{{photon}} =\:\frac{{E}}{{c}}\:.....\left({i}\right) \\ $$$${P}\:=\:{mv}\:.....\left({ii}\right) \\ $$$$\:{v}\:=\:\frac{\Delta{x}}{\Delta{t}}\:.....\left({iii}\right) \\ $$$$\mathrm{by}\:\mathrm{conservation}\:\mathrm{of}\:\mathrm{momentum}, \\ $$$$\:{M}\frac{\Delta{x}}{\Delta{t}}\:=\:\frac{{E}}{{c}} \\ $$$$\:\Delta{t}\:=\:\frac{{L}}{{c}}\:......\left({iv}\right) \\ $$$$\Rightarrow\:{M}\Delta{x}\:=\:\frac{{EL}}{{c}^{\mathrm{2}} } \\ $$$$\mathrm{also}\:\bar {{x}}\:=\:\frac{{Mx}_{\mathrm{1}} \:+\:{mx}_{\mathrm{2}} }{{M}\:+\:{m}} \\ $$$$\Rightarrow\:\frac{{Mx}_{\mathrm{1}} \:+\:{mx}_{\mathrm{2}} }{{M}\:+\:{m}}\:=\:\frac{{M}\left({x}_{\mathrm{1}} \:−\Delta{x}\right)\:+\:{mL}}{{M}+\:{m}} \\ $$$$\Rightarrow\:{ML}\:=\:{M}\Delta{x} \\ $$$$\Rightarrow\:{ML}\:=\:\frac{{EL}}{{c}^{\mathrm{2}} } \\ $$$${E}\:=\:{Mc}^{\mathrm{2}} \\ $$
Answered by Rio Michael last updated on 11/Jun/20
![KE = ∫_0 ^s F ds where F = ((d(mv))/dt) ⇒ KE = ∫_0 ^s ((d(mv))/dt) ds = ∫_0 ^(mv) v d(mv) = ∫_0 ^v v d[((m_0 v)/(√(1 −v^2 /c^2 )))] integrating by parts KE = ((m_0 v^2 )/(√(1−v^2 /c^2 ))) −m_0 ∫_0 ^v ((v dv)/(√(1−v^2 /c^2 ))) = ((m_0 v^2 )/(√(1−v^2 /c^2 ))) + ∣m_0 v^2 = ((m_0 c^2 )/(√(1−v^2 /c^2 ))) + m_0 c^2 = mc^2 − m_0 c^2 ⇒ mc^2 = m_0 c^2 +KE ⇒ E = E_0 + KE where E_0 = m_0 c^2 also see that E = mc^2 = ((m_0 c^2 )/(√(1−v^2 /c^2 ))) .](Q98011.png)
$$\:{KE}\:=\:\int_{\mathrm{0}} ^{{s}} {F}\:{ds}\: \\ $$$$\mathrm{where}\:{F}\:=\:\frac{{d}\left({mv}\right)}{{dt}} \\ $$$$\Rightarrow\:{KE}\:=\:\int_{\mathrm{0}} ^{{s}} \frac{{d}\left({mv}\right)}{{dt}}\:{ds}\:=\:\int_{\mathrm{0}} ^{{mv}} {v}\:{d}\left({mv}\right)\:=\:\int_{\mathrm{0}} ^{{v}} {v}\:{d}\left[\frac{{m}_{\mathrm{0}} {v}}{\sqrt{\mathrm{1}\:−{v}^{\mathrm{2}} /{c}^{\mathrm{2}} }}\right] \\ $$$$\mathrm{integrating}\:\mathrm{by}\:\mathrm{parts} \\ $$$${KE}\:=\:\frac{{m}_{\mathrm{0}} {v}^{\mathrm{2}} }{\sqrt{\mathrm{1}−{v}^{\mathrm{2}} /{c}^{\mathrm{2}} }}\:−{m}_{\mathrm{0}} \:\int_{\mathrm{0}} ^{{v}} \frac{{v}\:{dv}}{\sqrt{\mathrm{1}−{v}^{\mathrm{2}} /{c}^{\mathrm{2}} }} \\ $$$$\:\:\:\:\:\:\:\:=\:\frac{{m}_{\mathrm{0}} {v}^{\mathrm{2}} }{\sqrt{\mathrm{1}−{v}^{\mathrm{2}} /{c}^{\mathrm{2}} }}\:+\:\mid{m}_{\mathrm{0}} {v}^{\mathrm{2}} \\ $$$$\:\:\:\:\:\:\:\:=\:\frac{{m}_{\mathrm{0}} {c}^{\mathrm{2}} }{\sqrt{\mathrm{1}−{v}^{\mathrm{2}} /{c}^{\mathrm{2}} }}\:+\:{m}_{\mathrm{0}} {c}^{\mathrm{2}} \\ $$$$\:\:\:\:\:\:\:=\:{mc}^{\mathrm{2}} \:−\:{m}_{\mathrm{0}} {c}^{\mathrm{2}} \\ $$$$\Rightarrow\:{mc}^{\mathrm{2}} \:=\:{m}_{\mathrm{0}} {c}^{\mathrm{2}} \:+{KE} \\ $$$$\Rightarrow\:{E}\:=\:{E}_{\mathrm{0}} \:+\:{KE} \\ $$$$\mathrm{where}\:{E}_{\mathrm{0}} \:=\:{m}_{\mathrm{0}} {c}^{\mathrm{2}} \: \\ $$$$\mathrm{also}\:\mathrm{see}\:\mathrm{that}\:{E}\:=\:{mc}^{\mathrm{2}} \:=\:\frac{{m}_{\mathrm{0}} {c}^{\mathrm{2}} }{\sqrt{\mathrm{1}−{v}^{\mathrm{2}} /{c}^{\mathrm{2}} }}\:.\: \\ $$
Commented by M±th+et+s last updated on 11/Jun/20
$${well}\:{done}\:{thank}\:{you} \\ $$
Commented by Rio Michael last updated on 11/Jun/20

$$\mathrm{welcome}\:\mathrm{sir}. \\ $$
Answered by smridha last updated on 11/Jun/20
![let me show how I usually like to do this...this is not the method of the god of physics[EINSTEIN]. with due respect to him let′s start. ★let′s do some thought expt:★ consider two inertial frame of reference s and s′.now an atom is placed into the s′which is moving very very fast at a speed u with respect to s.now consider two observers A_s and A_(s′) .now suppose the atom emits two photon of frequency 𝛎,one is going towards both the observers and another is going farther away from them. now let′s see how the energy and momentum changed with respect to two observers. for the observer A_(s^′ ) the change of momentum is (𝚫p)_s^′ =((h𝛎)/c)−((h𝛎)/c)=0 because it′s remain standing with rest with respect to A_s^′ (acc to 1st postulate of Relativity) now change of of energy (𝚫E)_s^′ =2h𝛎. now let′s see what is going on with A_s .we fast consider the atom emits two photon from the moving fram of reference s^′ (respect to s).. and the s^′ frame moving very very fast so now Relativistic doppler effect comes with a magical spell on observer A_s . the change frequency of photon which comes towards A_s 𝛎_1 =𝛎(√((1+(u/c))/(1−(u/c)))) (blueshift) and which is moving away from A_(s ) ,ν_2 =𝛎(√((1−(u/c))/(1+(u/c)))) (red shift) so the change energy in frame s with respect to s^′ is (𝚫E)_s =h𝛎_1 +h𝛎_2 =((2h𝛎)/(√(1−(u^2 /c^2 ))))=𝛄(𝚫E)_s^′ where 𝛄=[1−(u^2 /c^2 )]^(−(1/2)) now change in momentum (𝚫p)_s =((h𝛎_1 )/c)−((h𝛎_2 )/c)=((𝛄(𝚫E)_s^′ )/c^2 )u=(((𝚫E)_s )/c^2 )u....(a) now consider the mass of the atom is (𝚫m) very small.and for ovserver A_s the mass is moving so it has momentum so now from eq^n ( a)we get (𝚫m)_s u=(((𝚫E)_s )/c^2 )u[c speed of light] now our worlds famous eq^n come which really changed the world is... (𝚫E)_s =(𝚫m)_s c^2 but acctually most logical eq^n is E=(√(p^2 c^2 +m^2 c^4 )) in original paper of Einstein the equantion is like that m=(L/v^2 ) ′′ does the inertia of body depend upon it′s energy content′′ the titel of this paper.](Q98028.png)
$$\boldsymbol{{let}}\:\boldsymbol{{me}}\:\boldsymbol{{show}}\:\boldsymbol{{how}}\:\boldsymbol{{I}}\:\boldsymbol{{usually}}\:\boldsymbol{{like}}\:\boldsymbol{{to}} \\ $$$$\boldsymbol{{do}}\:\boldsymbol{{this}}...\boldsymbol{{this}}\:\boldsymbol{{is}}\:\boldsymbol{{not}}\:\boldsymbol{{the}}\:\boldsymbol{{method}}\: \\ $$$$\boldsymbol{{of}}\:\boldsymbol{{the}}\:\boldsymbol{{god}}\:\boldsymbol{{of}}\:\boldsymbol{{physics}}\left[\mathbb{EINSTEIN}\right]. \\ $$$$\boldsymbol{{with}}\:\boldsymbol{{due}}\:\boldsymbol{{respect}}\:\boldsymbol{{to}}\:\boldsymbol{{him}}\:\boldsymbol{{let}}'\boldsymbol{{s}}\:\boldsymbol{{start}}. \\ $$$$\:\:\bigstar\boldsymbol{{let}}'{s}\:\boldsymbol{{do}}\:\boldsymbol{{some}}\:\boldsymbol{{thought}}\:\boldsymbol{{expt}}:\bigstar \\ $$$$\boldsymbol{{consider}}\:\boldsymbol{{two}}\:\boldsymbol{{inertial}}\:\boldsymbol{{frame}}\:\boldsymbol{{of}}\:\boldsymbol{{reference}} \\ $$$$\boldsymbol{{s}}\:\boldsymbol{{and}}\:\boldsymbol{{s}}'.\boldsymbol{{now}}\:\boldsymbol{{an}}\:\boldsymbol{{atom}}\:\boldsymbol{{is}}\:\boldsymbol{{placed}}\: \\ $$$$\boldsymbol{{into}}\:\boldsymbol{{the}}\:\boldsymbol{{s}}'\boldsymbol{{which}}\:\boldsymbol{{is}}\:\boldsymbol{{moving}}\:\boldsymbol{{very}}\: \\ $$$$\boldsymbol{{very}}\:\boldsymbol{{fast}}\:\boldsymbol{{at}}\:\boldsymbol{{a}}\:\boldsymbol{{speed}}\:\boldsymbol{{u}}\:\boldsymbol{{with}}\:\boldsymbol{{respect}} \\ $$$$\boldsymbol{{to}}\:\boldsymbol{{s}}.\boldsymbol{{now}}\:\boldsymbol{{consider}}\:\boldsymbol{{two}}\:\boldsymbol{{observers}} \\ $$$$\boldsymbol{{A}}_{\boldsymbol{{s}}} \boldsymbol{{and}}\:\boldsymbol{{A}}_{\boldsymbol{{s}}'} .\boldsymbol{{now}}\:\boldsymbol{{suppose}}\:\boldsymbol{{the}}\:\boldsymbol{{atom}} \\ $$$$\boldsymbol{{emits}}\:\boldsymbol{{two}}\:\boldsymbol{{photon}}\:\boldsymbol{{of}}\:\boldsymbol{{frequency}} \\ $$$$\boldsymbol{\nu},\boldsymbol{{one}}\:\boldsymbol{{is}}\:\boldsymbol{{going}}\:\boldsymbol{{towards}}\:\boldsymbol{{both}}\:\boldsymbol{{the}} \\ $$$$\boldsymbol{{observers}}\:\boldsymbol{{and}}\:\boldsymbol{{another}}\:\boldsymbol{{is}}\:\boldsymbol{{going}} \\ $$$$\boldsymbol{{farther}}\:\boldsymbol{{away}}\:\boldsymbol{{from}}\:\boldsymbol{{them}}. \\ $$$$\boldsymbol{{now}}\:\boldsymbol{{let}}'\boldsymbol{{s}}\:\boldsymbol{{see}}\:\boldsymbol{{how}}\:\boldsymbol{{the}}\:\boldsymbol{{energy}}\:\boldsymbol{{and}} \\ $$$$\boldsymbol{{momentum}}\:\boldsymbol{{changed}}\:\boldsymbol{{with}}\:\boldsymbol{{respect}} \\ $$$$\boldsymbol{{to}}\:\boldsymbol{{two}}\:\boldsymbol{{observers}}. \\ $$$$\boldsymbol{{for}}\:\boldsymbol{{the}}\:\boldsymbol{{observer}}\:\boldsymbol{{A}}_{{s}\:^{'} } \boldsymbol{{the}}\:\boldsymbol{{change}}\:\boldsymbol{{of}} \\ $$$$\boldsymbol{{momentum}}\:\boldsymbol{{is}}\:\left(\boldsymbol{\Delta}{p}\right)_{\boldsymbol{{s}}^{'} } =\frac{\boldsymbol{{h}\nu}}{\boldsymbol{{c}}}−\frac{\boldsymbol{{h}\nu}}{\boldsymbol{{c}}}=\mathrm{0} \\ $$$$\boldsymbol{{because}}\:\boldsymbol{{it}}'{s}\:\boldsymbol{{remain}}\:\boldsymbol{{standing}}\: \\ $$$$\boldsymbol{{with}}\:\boldsymbol{{rest}}\:\boldsymbol{{with}}\:\boldsymbol{{respect}}\:\boldsymbol{{to}}\:\boldsymbol{{A}}_{\boldsymbol{{s}}^{'} } \left(\boldsymbol{{acc}}\right. \\ $$$$\left.\boldsymbol{{to}}\:\mathrm{1}\boldsymbol{{st}}\:\boldsymbol{{postulate}}\:\boldsymbol{{of}}\:\boldsymbol{{R}}{e}\boldsymbol{{lativity}}\right) \\ $$$$\boldsymbol{{now}}\:\boldsymbol{{change}}\:\boldsymbol{{of}}\:\boldsymbol{{of}}\:\boldsymbol{{energy}} \\ $$$$\left(\boldsymbol{\Delta{E}}\right)_{{s}^{'} } =\mathrm{2}\boldsymbol{{h}\nu}. \\ $$$$\boldsymbol{{now}}\:\boldsymbol{{let}}'{s}\:\boldsymbol{{see}}\:\boldsymbol{{what}}\:\boldsymbol{{is}}\:\boldsymbol{{going}}\:\boldsymbol{{on}}\:\boldsymbol{{with}} \\ $$$$\boldsymbol{{A}}_{{s}} .\boldsymbol{{we}}\:\boldsymbol{{fast}}\:\boldsymbol{{consider}}\:\boldsymbol{{the}}\:\boldsymbol{{atom}}\:\boldsymbol{{emits}} \\ $$$$\boldsymbol{{two}}\:\boldsymbol{{photon}}\:\boldsymbol{{from}}\:\boldsymbol{{the}}\:\boldsymbol{{moving}}\:\boldsymbol{{fram}} \\ $$$$\boldsymbol{{of}}\:\boldsymbol{{reference}}\:\boldsymbol{{s}}^{'} \left(\boldsymbol{{respect}}\:\boldsymbol{{to}}\:\boldsymbol{{s}}\right).. \\ $$$$\boldsymbol{{and}}\:\boldsymbol{{the}}\:\boldsymbol{{s}}^{'} {frame}\:\boldsymbol{{moving}}\:\boldsymbol{{very}}\:\boldsymbol{{very}} \\ $$$$\boldsymbol{{fast}}\:\boldsymbol{{so}}\:\boldsymbol{{now}}\:\boldsymbol{{R}}{e}\boldsymbol{{lativistic}}\:\boldsymbol{{doppler}} \\ $$$$\boldsymbol{{effect}}\:\boldsymbol{{comes}}\:\boldsymbol{{with}}\:\boldsymbol{{a}}\:\boldsymbol{{magical}} \\ $$$$\boldsymbol{{spell}}\:\boldsymbol{{on}}\:\boldsymbol{{observer}}\:\boldsymbol{{A}}_{{s}} . \\ $$$$\boldsymbol{{the}}\:\boldsymbol{{change}}\:\boldsymbol{{frequency}}\:\boldsymbol{{of}}\:\boldsymbol{{photon}} \\ $$$$\boldsymbol{{which}}\:\boldsymbol{{comes}}\:\boldsymbol{{towards}}\:\boldsymbol{{A}}_{{s}} \\ $$$$\boldsymbol{\nu}_{\mathrm{1}} =\boldsymbol{\nu}\sqrt{\frac{\mathrm{1}+\frac{\boldsymbol{{u}}}{\boldsymbol{{c}}}}{\mathrm{1}−\frac{\boldsymbol{{u}}}{\boldsymbol{{c}}}}}\:\left(\boldsymbol{{blueshift}}\right) \\ $$$${and}\:{whi}\boldsymbol{{ch}}\:\boldsymbol{{is}}\:\boldsymbol{{moving}}\:\boldsymbol{{away}}\:\boldsymbol{{from}}\: \\ $$$$\boldsymbol{{A}}_{{s}\:} ,\nu_{\mathrm{2}} =\boldsymbol{\nu}\sqrt{\frac{\mathrm{1}−\frac{\boldsymbol{{u}}}{\boldsymbol{{c}}}}{\mathrm{1}+\frac{\boldsymbol{{u}}}{\boldsymbol{{c}}}}}\:\left(\boldsymbol{{red}}\:\boldsymbol{{shift}}\right) \\ $$$$\boldsymbol{{so}}\:\boldsymbol{{the}}\:\boldsymbol{{change}}\:\boldsymbol{{energy}}\:\boldsymbol{{in}} \\ $$$$\boldsymbol{{frame}}\:\boldsymbol{{s}}\:\boldsymbol{{with}}\:\boldsymbol{{respect}}\:\boldsymbol{{to}}\:\boldsymbol{{s}}^{'} \:{is} \\ $$$$\:\:\:\:\left(\boldsymbol{\Delta{E}}\right)_{\boldsymbol{{s}}} =\boldsymbol{{h}\nu}_{\mathrm{1}} +\boldsymbol{{h}\nu}_{\mathrm{2}} =\frac{\mathrm{2}\boldsymbol{{h}\nu}}{\sqrt{\mathrm{1}−\frac{\boldsymbol{{u}}^{\mathrm{2}} }{\boldsymbol{{c}}^{\mathrm{2}} }}}=\boldsymbol{\gamma}\left(\boldsymbol{\Delta{E}}\right)_{\boldsymbol{{s}}^{'} } \\ $$$$\boldsymbol{{where}}\:\boldsymbol{\gamma}=\left[\mathrm{1}−\frac{\boldsymbol{{u}}^{\mathrm{2}} }{\boldsymbol{{c}}^{\mathrm{2}} }\right]^{−\frac{\mathrm{1}}{\mathrm{2}}} \\ $$$$\boldsymbol{{now}}\:\boldsymbol{{change}}\:\boldsymbol{{in}}\:\boldsymbol{{momentum}} \\ $$$$\left(\boldsymbol{\Delta{p}}\right)_{\boldsymbol{{s}}} =\frac{\boldsymbol{{h}\nu}_{\mathrm{1}} }{\boldsymbol{{c}}}−\frac{\boldsymbol{{h}\nu}_{\mathrm{2}} }{{c}}=\frac{\boldsymbol{\gamma}\left(\boldsymbol{\Delta{E}}\right)_{{s}^{'} } }{\boldsymbol{{c}}^{\mathrm{2}} }\boldsymbol{{u}}=\frac{\left(\boldsymbol{\Delta{E}}\right)_{{s}} }{\boldsymbol{{c}}^{\mathrm{2}} }\boldsymbol{{u}}....\left(\boldsymbol{{a}}\right) \\ $$$$\boldsymbol{{now}}\:\boldsymbol{{consider}}\:\boldsymbol{{the}}\:\boldsymbol{{mass}}\:\boldsymbol{{of}}\:\boldsymbol{{the}}\: \\ $$$$\boldsymbol{{atom}}\:\boldsymbol{{is}}\:\left(\boldsymbol{\Delta{m}}\right)\:\boldsymbol{{very}}\:\boldsymbol{{small}}.\boldsymbol{{and}} \\ $$$$\boldsymbol{{for}}\:\boldsymbol{{ovserver}}\:\boldsymbol{{A}}_{\boldsymbol{{s}}} \:\boldsymbol{{the}}\:\boldsymbol{{mass}}\:\boldsymbol{{is}}\:\boldsymbol{{moving}} \\ $$$$\boldsymbol{{so}}\:\boldsymbol{{it}}\:\boldsymbol{{has}}\:\boldsymbol{{momentum}}\:\boldsymbol{{so}}\:\boldsymbol{{now}}\:\boldsymbol{{from}} \\ $$$$\boldsymbol{{eq}}^{\boldsymbol{{n}}} \left(\:\boldsymbol{{a}}\right)\boldsymbol{{we}}\:\boldsymbol{{get}} \\ $$$$\left(\boldsymbol{\Delta{m}}\right)_{\boldsymbol{{s}}} \boldsymbol{{u}}=\frac{\left(\boldsymbol{\Delta{E}}\right)_{{s}} }{\boldsymbol{{c}}^{\mathrm{2}} }\boldsymbol{{u}}\left[\boldsymbol{{c}}\:\boldsymbol{{speed}}\:\boldsymbol{{of}}\:\boldsymbol{{light}}\right] \\ $$$$\boldsymbol{{now}}\:\boldsymbol{{our}}\:\boldsymbol{{worlds}}\:\boldsymbol{{famous}}\:\boldsymbol{{eq}}^{\boldsymbol{{n}}} \boldsymbol{{come}} \\ $$$$\boldsymbol{{which}}\:\boldsymbol{{really}}\:\boldsymbol{{changed}}\:\boldsymbol{{the}}\:\boldsymbol{{world}} \\ $$$$\boldsymbol{{is}}... \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\left(\boldsymbol{\Delta{E}}\right)_{{s}} =\left(\boldsymbol{\Delta{m}}\right)_{\boldsymbol{{s}}} \boldsymbol{{c}}^{\mathrm{2}} \\ $$$$\boldsymbol{{but}}\:\boldsymbol{{acctually}}\:\boldsymbol{{most}}\:\boldsymbol{{logical}} \\ $$$$\boldsymbol{{eq}}^{\boldsymbol{{n}}} \:\boldsymbol{{is}}\:\boldsymbol{{E}}=\sqrt{\boldsymbol{{p}}^{\mathrm{2}} \boldsymbol{{c}}^{\mathrm{2}} +\boldsymbol{{m}}^{\mathrm{2}} \boldsymbol{{c}}^{\mathrm{4}} } \\ $$$$\boldsymbol{{in}} \\ $$$$\boldsymbol{{original}}\:\boldsymbol{{paper}}\:\boldsymbol{{of}}\:\:\boldsymbol{{E}}{i}\boldsymbol{{nstein}} \\ $$$$\boldsymbol{{the}}\:\boldsymbol{{equantion}}\:\boldsymbol{{is}}\:\boldsymbol{{like}}\:\boldsymbol{{that}} \\ $$$$\:\:\:\:\:\:\:\:\:\boldsymbol{{m}}=\frac{\boldsymbol{{L}}}{\boldsymbol{{v}}^{\mathrm{2}} }\: \\ $$$$''\:\boldsymbol{{does}}\:\boldsymbol{{the}}\:\boldsymbol{{inertia}}\:\boldsymbol{{of}}\:\boldsymbol{{body}}\:\boldsymbol{{depend}} \\ $$$$\boldsymbol{{upon}}\:\boldsymbol{{it}}'\boldsymbol{{s}}\:\boldsymbol{{energy}}\:\boldsymbol{{content}}'' \\ $$$${t}\boldsymbol{{he}}\:\boldsymbol{{titel}}\:\boldsymbol{{of}}\:\boldsymbol{{this}}\:\boldsymbol{{paper}}. \\ $$$$ \\ $$
Commented by M±th+et+s last updated on 11/Jun/20
$${nice}\:{work}\:{thank}\:{you}\:{sir} \\ $$
Commented by smridha last updated on 11/Jun/20

$$\boldsymbol{{welcome}}. \\ $$
