Question and Answers Forum

All Questions      Topic List

Differentiation Questions

Previous in All Question      Next in All Question      

Previous in Differentiation      Next in Differentiation      

Question Number 138112 by 676597498 last updated on 10/Apr/21

prove that ∫_0 ^( (π/2)) (sin^(2k) (x)dx)=(((2k)!2^(−2k−1) )/((k!)^2 ))

$${prove}\:{that} \\ $$$$\int_{\mathrm{0}} ^{\:\frac{\pi}{\mathrm{2}}} \left({sin}^{\mathrm{2}{k}} \left({x}\right){dx}\right)=\frac{\left(\mathrm{2}{k}\right)!\mathrm{2}^{−\mathrm{2}{k}−\mathrm{1}} }{\left({k}!\right)^{\mathrm{2}} } \\ $$

Answered by Dwaipayan Shikari last updated on 10/Apr/21

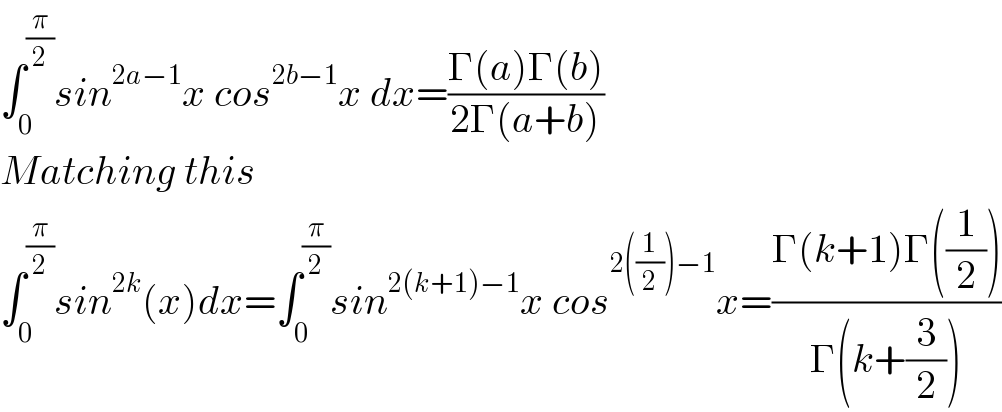
∫_0 ^(π/2) sin^(2a−1) x cos^(2b−1) x dx=((Γ(a)Γ(b))/(2Γ(a+b))) Matching this ∫_0 ^(π/2) sin^(2k) (x)dx=∫_0 ^(π/2) sin^(2(k+1)−1) x cos^(2((1/2))−1) x=((Γ(k+1)Γ((1/2)))/(Γ(k+(3/2))))

$$\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} {sin}^{\mathrm{2}{a}−\mathrm{1}} {x}\:{cos}^{\mathrm{2}{b}−\mathrm{1}} {x}\:{dx}=\frac{\Gamma\left({a}\right)\Gamma\left({b}\right)}{\mathrm{2}\Gamma\left({a}+{b}\right)} \\ $$$${Matching}\:{this} \\ $$$$\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} {sin}^{\mathrm{2}{k}} \left({x}\right){dx}=\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} {sin}^{\mathrm{2}\left({k}+\mathrm{1}\right)−\mathrm{1}} {x}\:{cos}^{\mathrm{2}\left(\frac{\mathrm{1}}{\mathrm{2}}\right)−\mathrm{1}} {x}=\frac{\Gamma\left({k}+\mathrm{1}\right)\Gamma\left(\frac{\mathrm{1}}{\mathrm{2}}\right)}{\Gamma\left({k}+\frac{\mathrm{3}}{\mathrm{2}}\right)} \\ $$

Answered by mathmax by abdo last updated on 10/Apr/21

let I_n =∫_0 ^(π/2) sin^n xdx ⇒I_n =∫_0 ^(π/2) sin^(n−2) x (1−cos^2 x)dx =∫_0 ^(π/2) sin^(n−2) xdx−∫_0 ^(π/2) cos^2 x sin^(n−2) xdx we[have ∫_0 ^(π/2) sin^(n−2) xdx=I_(n−2) and by recurrence ∫_0 ^(π/2) cosx (cosx sin^(n−2) x)dx =[((sin^(n−1) x)/(n−1))cosx]_0 ^(π/2) +∫_0 ^(π/2) sinx ((sin^(n−1) x)/(n−1))dx =(1/(n−1))I_n ⇒I_n =I_(n−2) −(1/(n−1))I_n ⇒(1+(1/(n−1)))I_n =I_(n−2) ⇒ (n/(n−1))I_n =I_(n−2) ⇒I_n =((n−1)/n) I_(n−2) ⇒ I_(2n) =((2n−1)/(2n))I_(2n−2) (n≥1) ⇒Π_(k=1) ^(n ) I_(2k) =Π_(k=1) ^n ((2k−1)/(2k)) I_(2k−2) ⇒ I_2 .I_4 ......I_(2n) =((Π_(k=1) ^n (2k−1))/(2^n n!)) I_0 .I_2 .....I_(2n−2) ⇒ I_(2n) =((1.3.5....(2n−1))/(2^n .n!)) I_0 =(π/2) ((1.2.3.4.5.....(2n−1)(2n))/(2^n n! .2^(n .) n!)) =(π/2)×(((2n)!)/(2^(2n) (n!)^2 )) ⇒∫_0 ^(π/2) sin^(2n) xdx =((π(2n)!)/(2^(2n+1) (n!)^2 ))

$$\mathrm{let}\:\:\mathrm{I}_{\mathrm{n}} =\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \:\mathrm{sin}^{\mathrm{n}} \mathrm{xdx}\:\Rightarrow\mathrm{I}_{\mathrm{n}} =\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \:\mathrm{sin}^{\mathrm{n}−\mathrm{2}} \mathrm{x}\:\left(\mathrm{1}−\mathrm{cos}^{\mathrm{2}} \mathrm{x}\right)\mathrm{dx} \\ $$$$=\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \:\mathrm{sin}^{\mathrm{n}−\mathrm{2}} \mathrm{xdx}−\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \:\mathrm{cos}^{\mathrm{2}} \mathrm{x}\:\mathrm{sin}^{\mathrm{n}−\mathrm{2}} \mathrm{xdx}\:\:\mathrm{we}\left[\mathrm{have}\right. \\ $$$$\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \:\mathrm{sin}^{\mathrm{n}−\mathrm{2}} \mathrm{xdx}=\mathrm{I}_{\mathrm{n}−\mathrm{2}} \:\:\mathrm{and}\:\mathrm{by}\:\mathrm{recurrence} \\ $$$$\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \mathrm{cosx}\:\left(\mathrm{cosx}\:\mathrm{sin}^{\mathrm{n}−\mathrm{2}} \mathrm{x}\right)\mathrm{dx}\:=\left[\frac{\mathrm{sin}^{\mathrm{n}−\mathrm{1}} \mathrm{x}}{\mathrm{n}−\mathrm{1}}\mathrm{cosx}\right]_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} +\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \mathrm{sinx}\:\frac{\mathrm{sin}^{\mathrm{n}−\mathrm{1}} \mathrm{x}}{\mathrm{n}−\mathrm{1}}\mathrm{dx} \\ $$$$=\frac{\mathrm{1}}{\mathrm{n}−\mathrm{1}}\mathrm{I}_{\mathrm{n}} \:\Rightarrow\mathrm{I}_{\mathrm{n}} =\mathrm{I}_{\mathrm{n}−\mathrm{2}} −\frac{\mathrm{1}}{\mathrm{n}−\mathrm{1}}\mathrm{I}_{\mathrm{n}} \:\Rightarrow\left(\mathrm{1}+\frac{\mathrm{1}}{\mathrm{n}−\mathrm{1}}\right)\mathrm{I}_{\mathrm{n}} =\mathrm{I}_{\mathrm{n}−\mathrm{2}} \:\Rightarrow \\ $$$$\frac{\mathrm{n}}{\mathrm{n}−\mathrm{1}}\mathrm{I}_{\mathrm{n}} =\mathrm{I}_{\mathrm{n}−\mathrm{2}} \:\Rightarrow\mathrm{I}_{\mathrm{n}} =\frac{\mathrm{n}−\mathrm{1}}{\mathrm{n}}\:\mathrm{I}_{\mathrm{n}−\mathrm{2}} \:\Rightarrow\:\mathrm{I}_{\mathrm{2n}} =\frac{\mathrm{2n}−\mathrm{1}}{\mathrm{2n}}\mathrm{I}_{\mathrm{2n}−\mathrm{2}} \:\:\:\:\left(\mathrm{n}\geqslant\mathrm{1}\right) \\ $$$$\Rightarrow\prod_{\mathrm{k}=\mathrm{1}} ^{\mathrm{n}\:} \:\mathrm{I}_{\mathrm{2k}} =\prod_{\mathrm{k}=\mathrm{1}} ^{\mathrm{n}} \:\frac{\mathrm{2k}−\mathrm{1}}{\mathrm{2k}}\:\mathrm{I}_{\mathrm{2k}−\mathrm{2}} \:\Rightarrow \\ $$$$\mathrm{I}_{\mathrm{2}} .\mathrm{I}_{\mathrm{4}} ......\mathrm{I}_{\mathrm{2n}} =\frac{\prod_{\mathrm{k}=\mathrm{1}} ^{\mathrm{n}} \:\left(\mathrm{2k}−\mathrm{1}\right)}{\mathrm{2}^{\mathrm{n}} \:\mathrm{n}!}\:\mathrm{I}_{\mathrm{0}} .\mathrm{I}_{\mathrm{2}} .....\mathrm{I}_{\mathrm{2n}−\mathrm{2}} \:\Rightarrow \\ $$$$\mathrm{I}_{\mathrm{2n}} =\frac{\mathrm{1}.\mathrm{3}.\mathrm{5}....\left(\mathrm{2n}−\mathrm{1}\right)}{\mathrm{2}^{\mathrm{n}} .\mathrm{n}!}\:\mathrm{I}_{\mathrm{0}} =\frac{\pi}{\mathrm{2}}\:\frac{\mathrm{1}.\mathrm{2}.\mathrm{3}.\mathrm{4}.\mathrm{5}.....\left(\mathrm{2n}−\mathrm{1}\right)\left(\mathrm{2n}\right)}{\mathrm{2}^{\mathrm{n}} \mathrm{n}!\:.\mathrm{2}^{\mathrm{n}\:.} \mathrm{n}!} \\ $$$$=\frac{\pi}{\mathrm{2}}×\frac{\left(\mathrm{2n}\right)!}{\mathrm{2}^{\mathrm{2n}} \left(\mathrm{n}!\right)^{\mathrm{2}} }\:\Rightarrow\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \:\mathrm{sin}^{\mathrm{2n}} \mathrm{xdx}\:=\frac{\pi\left(\mathrm{2n}\right)!}{\mathrm{2}^{\mathrm{2n}+\mathrm{1}} \left(\mathrm{n}!\right)^{\mathrm{2}} } \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com