Question and Answers Forum

All Questions      Topic List

None Questions

Previous in All Question      Next in All Question      

Previous in None      Next in None      

Question Number 114037 by mohammad17 last updated on 16/Sep/20

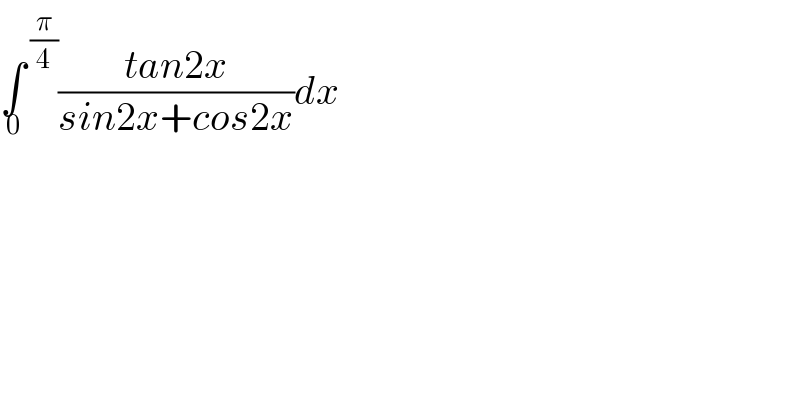
∫^( (π/4)) _0 ((tan2x)/(sin2x+cos2x))dx

$$\underset{\mathrm{0}} {\int}^{\:\frac{\pi}{\mathrm{4}}} \frac{{tan}\mathrm{2}{x}}{{sin}\mathrm{2}{x}+{cos}\mathrm{2}{x}}{dx} \\ $$

Answered by Dwaipayan Shikari last updated on 16/Sep/20

∫_0 ^(π/4) ((sin2x)/(cos2x(sin2x+cos2x)))dx ∫_0 ^(π/4) (1/(cos2x))−(1/(sin2x+cos2x))dx =∫_0 ^(π/4) sec2x−(1/( (√2)))∫_0 ^(π/4) (1/( cos((π/4)−2x)))dx =(1/2)[log(sec2x+tan2x)]_0 ^(π/4) −(1/( (√2)))∫_0 ^(π/4) sec((π/4)−2x)dx →∞ Divergent

$$\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{4}}} \frac{{sin}\mathrm{2}{x}}{{cos}\mathrm{2}{x}\left({sin}\mathrm{2}{x}+{cos}\mathrm{2}{x}\right)}{dx} \\ $$$$\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{4}}} \frac{\mathrm{1}}{{cos}\mathrm{2}{x}}−\frac{\mathrm{1}}{{sin}\mathrm{2}{x}+{cos}\mathrm{2}{x}}{dx} \\ $$$$=\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{4}}} {sec}\mathrm{2}{x}−\frac{\mathrm{1}}{\:\sqrt{\mathrm{2}}}\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{4}}} \frac{\mathrm{1}}{\:{cos}\left(\frac{\pi}{\mathrm{4}}−\mathrm{2}{x}\right)}{dx} \\ $$$$=\frac{\mathrm{1}}{\mathrm{2}}\left[{log}\left({sec}\mathrm{2}{x}+{tan}\mathrm{2}{x}\right)\right]_{\mathrm{0}} ^{\frac{\pi}{\mathrm{4}}} −\frac{\mathrm{1}}{\:\sqrt{\mathrm{2}}}\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{4}}} {sec}\left(\frac{\pi}{\mathrm{4}}−\mathrm{2}{x}\right){dx} \\ $$$$\rightarrow\infty \\ $$$${Divergent} \\ $$

Answered by mathmax by abdo last updated on 16/Sep/20

I =∫_0 ^(π/4) ((tan(2x))/(sin(2x)+cos(2x))) dx ⇒ I =_(2x=t) (1/2) ∫_0 ^(π/2) ((tan(t))/(sint +cos(t)))dt =_(tan((t/2))=u) (1/2) ∫_0 ^1 (((2u)/(1−u^2 ))/(((2u)/(1+u^2 ))+((1−u^2 )/(1+u^2 )))) du =(1/2) ∫_0 ^1 ((2u(1+u^2 ))/((2u+1−u^2 )(1−u^2 ))) du = ∫_0 ^1 ((u(u^2 +1))/((u^2 −2u−1)(u^2 −1))) du but at v(1) ((u(u^2 +1))/((u^2 −2u−1)(u^2 −1))) ∼(2/(−2(u^2 −1))) =(1/(1−u^2 )) and ∫_0 ^1 (du/(1−u^2 )) diverges ⇒ the intehral I is dkvervent ...!

$$\mathrm{I}\:=\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{4}}} \:\frac{\mathrm{tan}\left(\mathrm{2x}\right)}{\mathrm{sin}\left(\mathrm{2x}\right)+\mathrm{cos}\left(\mathrm{2x}\right)}\:\mathrm{dx}\:\Rightarrow\:\mathrm{I}\:=_{\mathrm{2x}=\mathrm{t}} \frac{\mathrm{1}}{\mathrm{2}}\:\:\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \:\frac{\mathrm{tan}\left(\mathrm{t}\right)}{\mathrm{sint}\:+\mathrm{cos}\left(\mathrm{t}\right)}\mathrm{dt} \\ $$$$=_{\mathrm{tan}\left(\frac{\mathrm{t}}{\mathrm{2}}\right)=\mathrm{u}} \:\:\:\:\frac{\mathrm{1}}{\mathrm{2}}\:\:\int_{\mathrm{0}} ^{\mathrm{1}} \:\frac{\frac{\mathrm{2u}}{\mathrm{1}−\mathrm{u}^{\mathrm{2}} }}{\frac{\mathrm{2u}}{\mathrm{1}+\mathrm{u}^{\mathrm{2}} }+\frac{\mathrm{1}−\mathrm{u}^{\mathrm{2}} }{\mathrm{1}+\mathrm{u}^{\mathrm{2}} }}\:\mathrm{du}\:=\frac{\mathrm{1}}{\mathrm{2}}\:\int_{\mathrm{0}} ^{\mathrm{1}} \:\frac{\mathrm{2u}\left(\mathrm{1}+\mathrm{u}^{\mathrm{2}} \right)}{\left(\mathrm{2u}+\mathrm{1}−\mathrm{u}^{\mathrm{2}} \right)\left(\mathrm{1}−\mathrm{u}^{\mathrm{2}} \right)}\:\mathrm{du} \\ $$$$=\:\int_{\mathrm{0}} ^{\mathrm{1}} \:\:\:\:\frac{\mathrm{u}\left(\mathrm{u}^{\mathrm{2}} \:+\mathrm{1}\right)}{\left(\mathrm{u}^{\mathrm{2}} −\mathrm{2u}−\mathrm{1}\right)\left(\mathrm{u}^{\mathrm{2}} −\mathrm{1}\right)}\:\mathrm{du}\:\:\mathrm{but}\:\mathrm{at}\:\mathrm{v}\left(\mathrm{1}\right) \\ $$$$\frac{\mathrm{u}\left(\mathrm{u}^{\mathrm{2}} \:+\mathrm{1}\right)}{\left(\mathrm{u}^{\mathrm{2}} −\mathrm{2u}−\mathrm{1}\right)\left(\mathrm{u}^{\mathrm{2}} −\mathrm{1}\right)}\:\sim\frac{\mathrm{2}}{−\mathrm{2}\left(\mathrm{u}^{\mathrm{2}} −\mathrm{1}\right)}\:=\frac{\mathrm{1}}{\mathrm{1}−\mathrm{u}^{\mathrm{2}} }\:\mathrm{and}\:\int_{\mathrm{0}} ^{\mathrm{1}} \:\frac{\mathrm{du}}{\mathrm{1}−\mathrm{u}^{\mathrm{2}} }\:\mathrm{diverges} \\ $$$$\Rightarrow\:\mathrm{the}\:\mathrm{intehral}\:\mathrm{I}\:\mathrm{is}\:\mathrm{dkvervent}\:...! \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com