
Question Number 123987 by mnjuly1970 last updated on 29/Nov/20
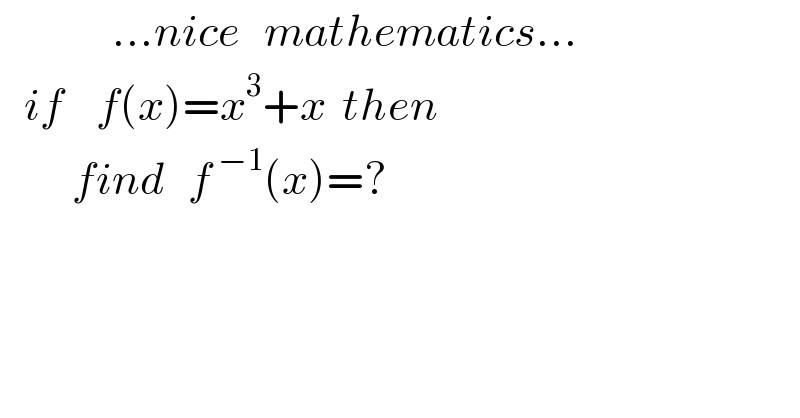
$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:...{nice}\:\:\:{mathematics}... \\ $$$$\:\:\:{if}\:\:\:\:{f}\left({x}\right)={x}^{\mathrm{3}} +{x}\:\:{then} \\ $$$$\:\:\:\:\:\:\:\:\:{find}\:\:\:{f}^{\:−\mathrm{1}} \left({x}\right)=? \\ $$$$\:\:\:\: \\ $$
Answered by MJS_new last updated on 29/Nov/20
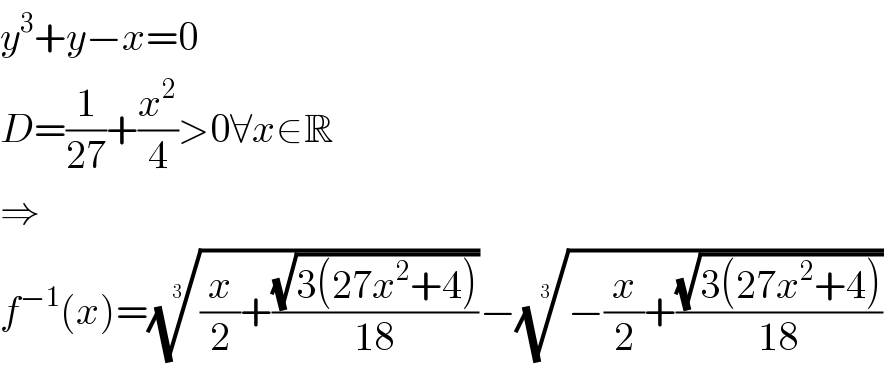
$${y}^{\mathrm{3}} +{y}−{x}=\mathrm{0} \\ $$$${D}=\frac{\mathrm{1}}{\mathrm{27}}+\frac{{x}^{\mathrm{2}} }{\mathrm{4}}>\mathrm{0}\forall{x}\in\mathbb{R} \\ $$$$\Rightarrow \\ $$$${f}^{−\mathrm{1}} \left({x}\right)=\sqrt[{\mathrm{3}}]{\frac{{x}}{\mathrm{2}}+\frac{\sqrt{\mathrm{3}\left(\mathrm{27}{x}^{\mathrm{2}} +\mathrm{4}\right)}}{\mathrm{18}}}−\sqrt[{\mathrm{3}}]{−\frac{{x}}{\mathrm{2}}+\frac{\sqrt{\mathrm{3}\left(\mathrm{27}{x}^{\mathrm{2}} +\mathrm{4}\right)}}{\mathrm{18}}} \\ $$
Commented by john_santu last updated on 30/Nov/20

$${Cardano} \\ $$
Commented by MJS_new last updated on 30/Nov/20

$$\mathrm{yes} \\ $$
