
Question Number 136555 by mnjuly1970 last updated on 23/Mar/21
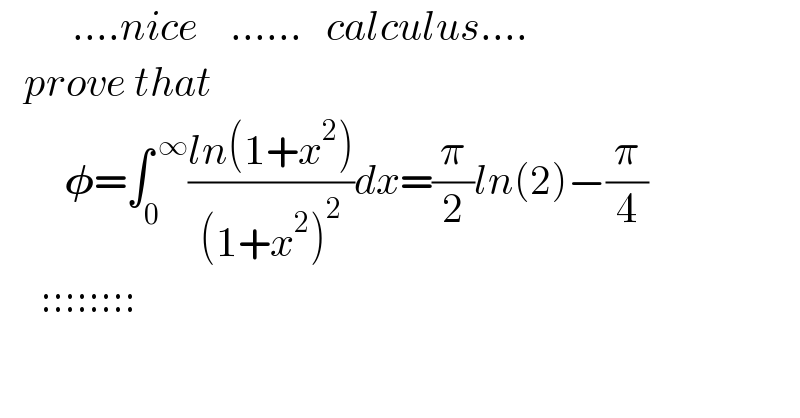
$$\:\:\:\:\:\:\:\:\:....{nice}\:\:\:\:......\:\:\:{calculus}.... \\ $$$$\:\:\:{prove}\:{that} \\ $$$$\:\:\:\:\:\:\:\:\boldsymbol{\phi}=\int_{\mathrm{0}} ^{\:\infty} \frac{{ln}\left(\mathrm{1}+{x}^{\mathrm{2}} \right)}{\left(\mathrm{1}+{x}^{\mathrm{2}} \right)^{\mathrm{2}} }{dx}=\frac{\pi}{\mathrm{2}}{ln}\left(\mathrm{2}\right)−\frac{\pi}{\mathrm{4}} \\ $$$$\:\:\:\:\::::::::: \\ $$
Answered by mnjuly1970 last updated on 23/Mar/21

$$\:\:\:{f}\left({a}\right)=\int_{\mathrm{0}} ^{\:\infty} \frac{{dx}}{\left(\mathrm{1}+{x}^{\mathrm{2}} \right)^{{a}} }\:\:\:\:...\left({a}>\frac{\mathrm{1}}{\mathrm{2}}\right) \\ $$$$\:\:\:\:\:{f}\left({a}\right)\overset{{x}^{\mathrm{2}} ={y}} {=}\frac{\mathrm{1}}{\mathrm{2}}\int_{\mathrm{0}} ^{\:\infty} \frac{{y}^{\frac{−\mathrm{1}}{\mathrm{2}}} }{\left(\mathrm{1}+{y}\right)^{{a}} }{dy}=\beta\left(\frac{\mathrm{1}}{\mathrm{2}},{a}−\frac{\mathrm{1}}{\mathrm{2}}\right) \\ $$$$\:\:\:\:\:{f}\left({a}\right)=\frac{\mathrm{1}}{\mathrm{2}}\left(\frac{\sqrt{\pi}\:\Gamma\left({a}−\frac{\mathrm{1}}{\mathrm{2}}\right)}{\Gamma\left({a}\right)}\right) \\ $$$$\:\:\:\:\:{goal}::\:\:\boldsymbol{\phi}=−{f}\:'\left(\mathrm{2}\right) \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:{f}\:'\left({a}\right)=\frac{\sqrt{\pi}}{\mathrm{2}}\:\left(\frac{\Gamma'\left({a}−\frac{\mathrm{1}}{\mathrm{2}}\right).\Gamma\left({a}\right)−\Gamma'\left({a}\right).\Gamma\left({a}−\frac{\mathrm{1}}{\mathrm{2}}\right)}{\Gamma^{\mathrm{2}} \left({a}\right)}\right) \\ $$$$\:\:\:\:\:\:\:\:\:\:{f}\:'\left(\mathrm{2}\right)=\frac{\sqrt{\pi}}{\mathrm{2}}\left(\frac{\psi\left(\frac{\mathrm{3}}{\mathrm{2}}\right)\Gamma\left(\frac{\mathrm{3}}{\mathrm{2}}\right)−\psi\left(\mathrm{2}\right)\Gamma\left(\frac{\mathrm{3}}{\mathrm{2}}\:\right)}{\mathrm{1}^{\mathrm{2}} }\right) \\ $$$$\:\:\:\:=\frac{\sqrt{\pi}}{\mathrm{2}}\left(\frac{\sqrt{\pi}}{\mathrm{2}}\left(\mathrm{2}−\gamma−\mathrm{2}{ln}\left(\mathrm{2}\right)−\left(\mathrm{1}−\gamma\right)\right)\right) \\ $$$$\:\:\:\:\:\:\:\therefore\:\:\:−\boldsymbol{\phi}=\frac{\pi}{\mathrm{4}}\left(\mathrm{2}−\gamma−\mathrm{2}{ln}\left(\mathrm{2}\right)−\mathrm{1}+\gamma\right) \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:=\frac{\pi}{\mathrm{4}}\left(\mathrm{1}−\mathrm{2}{ln}\left(\mathrm{2}\right)\right)=\frac{\pi}{\mathrm{4}}−\frac{\pi}{\mathrm{2}}{ln}\left(\mathrm{2}\right)..\checkmark \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\therefore\:\:\boldsymbol{\phi}=\frac{\pi}{\mathrm{2}}{ln}\left(\mathrm{2}\right)−\frac{\pi}{\mathrm{4}}\:.... \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\: \\ $$$$\:\:\:{m}.{n} \\ $$
Answered by mindispower last updated on 23/Mar/21
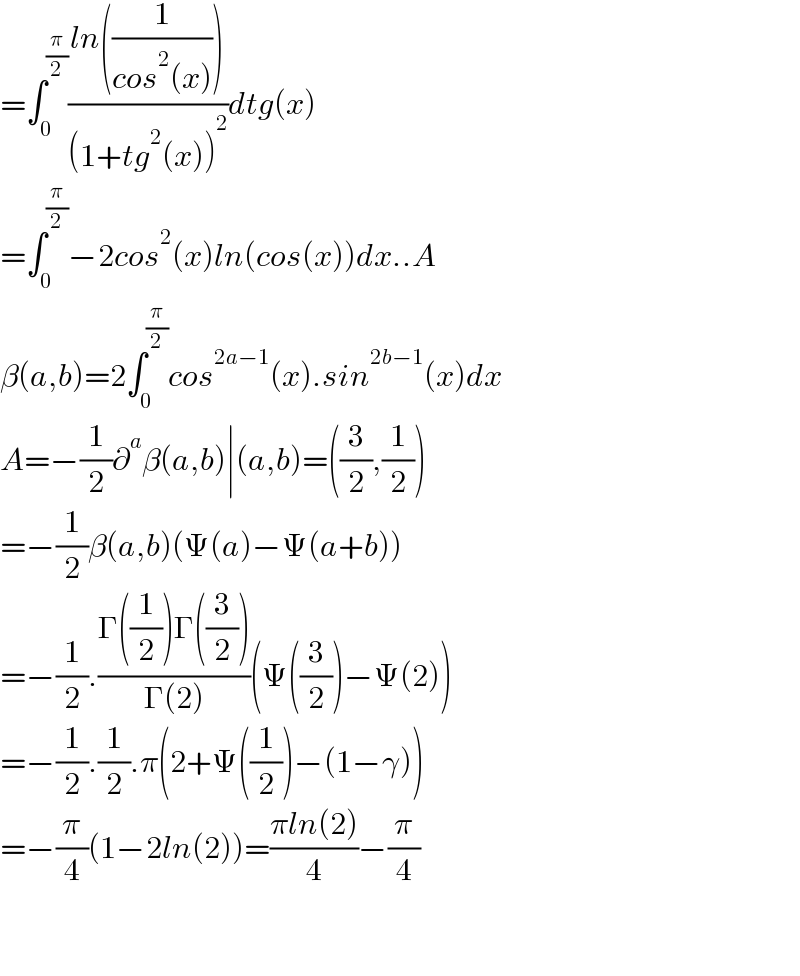
$$=\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \frac{{ln}\left(\frac{\mathrm{1}}{{cos}^{\mathrm{2}} \left({x}\right)}\right)}{\left(\mathrm{1}+{tg}^{\mathrm{2}} \left({x}\right)\right)^{\mathrm{2}} }{dtg}\left({x}\right) \\ $$$$=\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} −\mathrm{2}{cos}^{\mathrm{2}} \left({x}\right){ln}\left({cos}\left({x}\right)\right){dx}..{A} \\ $$$$\beta\left({a},{b}\right)=\mathrm{2}\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} {cos}^{\mathrm{2}{a}−\mathrm{1}} \left({x}\right).{sin}^{\mathrm{2}{b}−\mathrm{1}} \left({x}\right){dx} \\ $$$${A}=−\frac{\mathrm{1}}{\mathrm{2}}\partial^{{a}} \beta\left({a},{b}\right)\mid\left({a},{b}\right)=\left(\frac{\mathrm{3}}{\mathrm{2}},\frac{\mathrm{1}}{\mathrm{2}}\right) \\ $$$$=−\frac{\mathrm{1}}{\mathrm{2}}\beta\left({a},{b}\right)\left(\Psi\left({a}\right)−\Psi\left({a}+{b}\right)\right) \\ $$$$=−\frac{\mathrm{1}}{\mathrm{2}}.\frac{\Gamma\left(\frac{\mathrm{1}}{\mathrm{2}}\right)\Gamma\left(\frac{\mathrm{3}}{\mathrm{2}}\right)}{\Gamma\left(\mathrm{2}\right)}\left(\Psi\left(\frac{\mathrm{3}}{\mathrm{2}}\right)−\Psi\left(\mathrm{2}\right)\right) \\ $$$$=−\frac{\mathrm{1}}{\mathrm{2}}.\frac{\mathrm{1}}{\mathrm{2}}.\pi\left(\mathrm{2}+\Psi\left(\frac{\mathrm{1}}{\mathrm{2}}\right)−\left(\mathrm{1}−\gamma\right)\right) \\ $$$$=−\frac{\pi}{\mathrm{4}}\left(\mathrm{1}−\mathrm{2}{ln}\left(\mathrm{2}\right)\right)=\frac{\pi{ln}\left(\mathrm{2}\right)}{\mathrm{4}}−\frac{\pi}{\mathrm{4}} \\ $$$$ \\ $$$$ \\ $$
Commented by mnjuly1970 last updated on 23/Mar/21

$${thanks}\:{alot}.... \\ $$
Answered by Dwaipayan Shikari last updated on 23/Mar/21
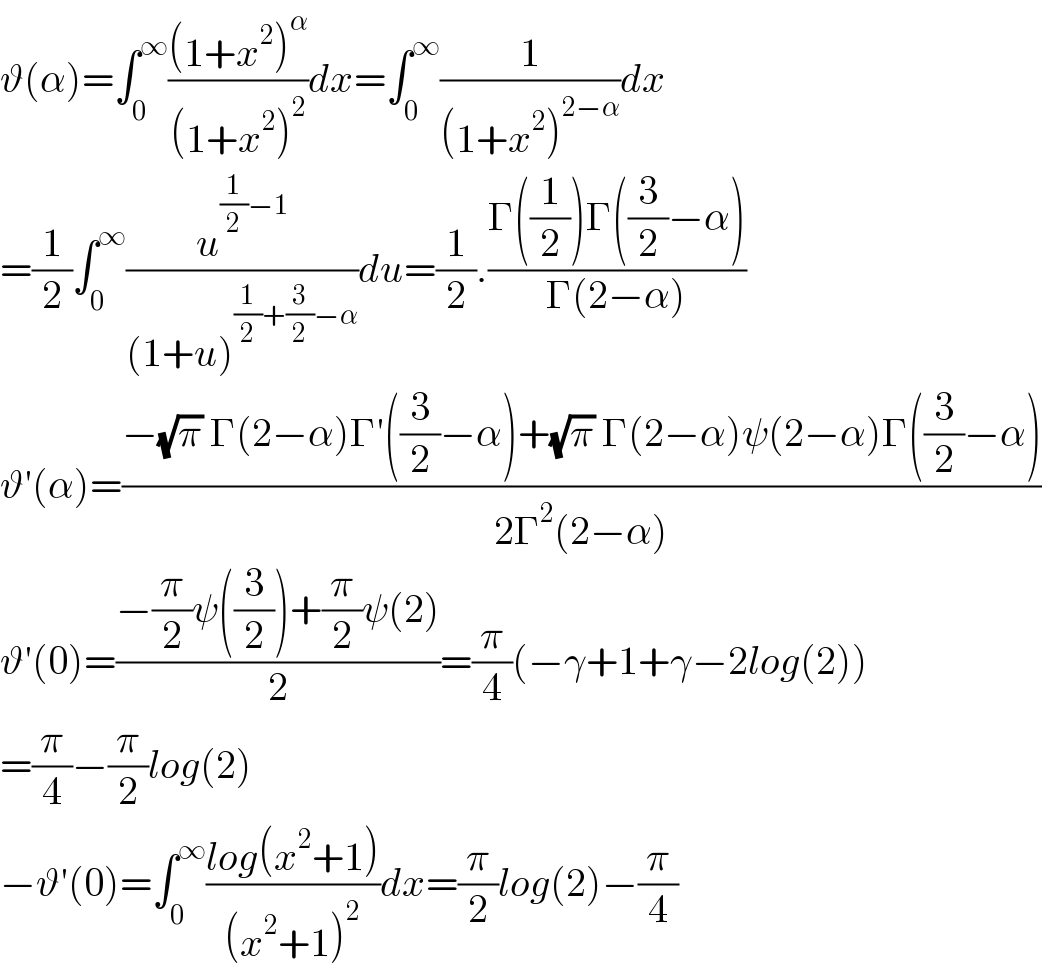
$$\vartheta\left(\alpha\right)=\int_{\mathrm{0}} ^{\infty} \frac{\left(\mathrm{1}+{x}^{\mathrm{2}} \right)^{\alpha} }{\left(\mathrm{1}+{x}^{\mathrm{2}} \right)^{\mathrm{2}} }{dx}=\int_{\mathrm{0}} ^{\infty} \frac{\mathrm{1}}{\left(\mathrm{1}+{x}^{\mathrm{2}} \right)^{\mathrm{2}−\alpha} }{dx} \\ $$$$=\frac{\mathrm{1}}{\mathrm{2}}\int_{\mathrm{0}} ^{\infty} \frac{{u}^{\frac{\mathrm{1}}{\mathrm{2}}−\mathrm{1}} }{\left(\mathrm{1}+{u}\right)^{\frac{\mathrm{1}}{\mathrm{2}}+\frac{\mathrm{3}}{\mathrm{2}}−\alpha} }{du}=\frac{\mathrm{1}}{\mathrm{2}}.\frac{\Gamma\left(\frac{\mathrm{1}}{\mathrm{2}}\right)\Gamma\left(\frac{\mathrm{3}}{\mathrm{2}}−\alpha\right)}{\Gamma\left(\mathrm{2}−\alpha\right)} \\ $$$$\vartheta'\left(\alpha\right)=\frac{−\sqrt{\pi}\:\Gamma\left(\mathrm{2}−\alpha\right)\Gamma'\left(\frac{\mathrm{3}}{\mathrm{2}}−\alpha\right)+\sqrt{\pi}\:\Gamma\left(\mathrm{2}−\alpha\right)\psi\left(\mathrm{2}−\alpha\right)\Gamma\left(\frac{\mathrm{3}}{\mathrm{2}}−\alpha\right)}{\mathrm{2}\Gamma^{\mathrm{2}} \left(\mathrm{2}−\alpha\right)} \\ $$$$\vartheta'\left(\mathrm{0}\right)=\frac{−\frac{\pi}{\mathrm{2}}\psi\left(\frac{\mathrm{3}}{\mathrm{2}}\right)+\frac{\pi}{\mathrm{2}}\psi\left(\mathrm{2}\right)}{\mathrm{2}}=\frac{\pi}{\mathrm{4}}\left(−\gamma+\mathrm{1}+\gamma−\mathrm{2}{log}\left(\mathrm{2}\right)\right) \\ $$$$=\frac{\pi}{\mathrm{4}}−\frac{\pi}{\mathrm{2}}{log}\left(\mathrm{2}\right) \\ $$$$−\vartheta'\left(\mathrm{0}\right)=\int_{\mathrm{0}} ^{\infty} \frac{{log}\left({x}^{\mathrm{2}} +\mathrm{1}\right)}{\left({x}^{\mathrm{2}} +\mathrm{1}\right)^{\mathrm{2}} }{dx}=\frac{\pi}{\mathrm{2}}{log}\left(\mathrm{2}\right)−\frac{\pi}{\mathrm{4}} \\ $$
Commented by mnjuly1970 last updated on 23/Mar/21

$${thanking}... \\ $$
