Question and Answers Forum

All Questions      Topic List

Integration Questions

Previous in All Question      Next in All Question      

Previous in Integration      Next in Integration      

Question Number 122636 by mnjuly1970 last updated on 18/Nov/20

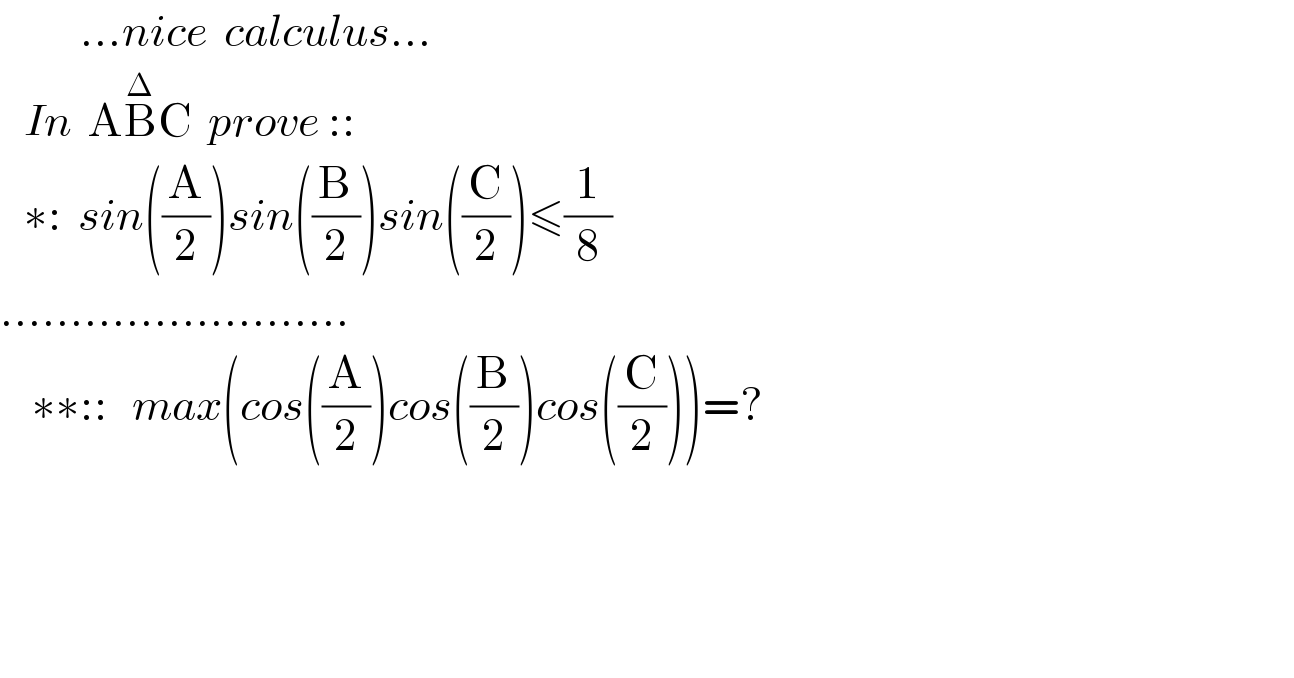
...nice calculus... In AB^Δ C prove :: ∗: sin((A/2))sin((B/2))sin((C/2))≤(1/8) ......................... ∗∗:: max(cos((A/2))cos((B/2))cos((C/2)))=?

$$\:\:\:\:\:\:\:\:\:\:...{nice}\:\:{calculus}... \\ $$$$\:\:\:{In}\:\:\mathrm{A}\overset{\Delta} {\mathrm{B}C}\:\:{prove}\:::\: \\ $$$$\:\:\:\ast:\:\:{sin}\left(\frac{\mathrm{A}}{\mathrm{2}}\right){sin}\left(\frac{\mathrm{B}}{\mathrm{2}}\right){sin}\left(\frac{\mathrm{C}}{\mathrm{2}}\right)\leqslant\frac{\mathrm{1}}{\mathrm{8}} \\ $$$$......................... \\ $$$$\:\:\:\:\ast\ast::\:\:\:{max}\left({cos}\left(\frac{\mathrm{A}}{\mathrm{2}}\right){cos}\left(\frac{\mathrm{B}}{\mathrm{2}}\right){cos}\left(\frac{\mathrm{C}}{\mathrm{2}}\right)\right)=? \\ $$$$\:\:\:\:\: \\ $$$$\: \\ $$$$\:\:\:\:\:\:\: \\ $$

Answered by TANMAY PANACEA last updated on 18/Nov/20

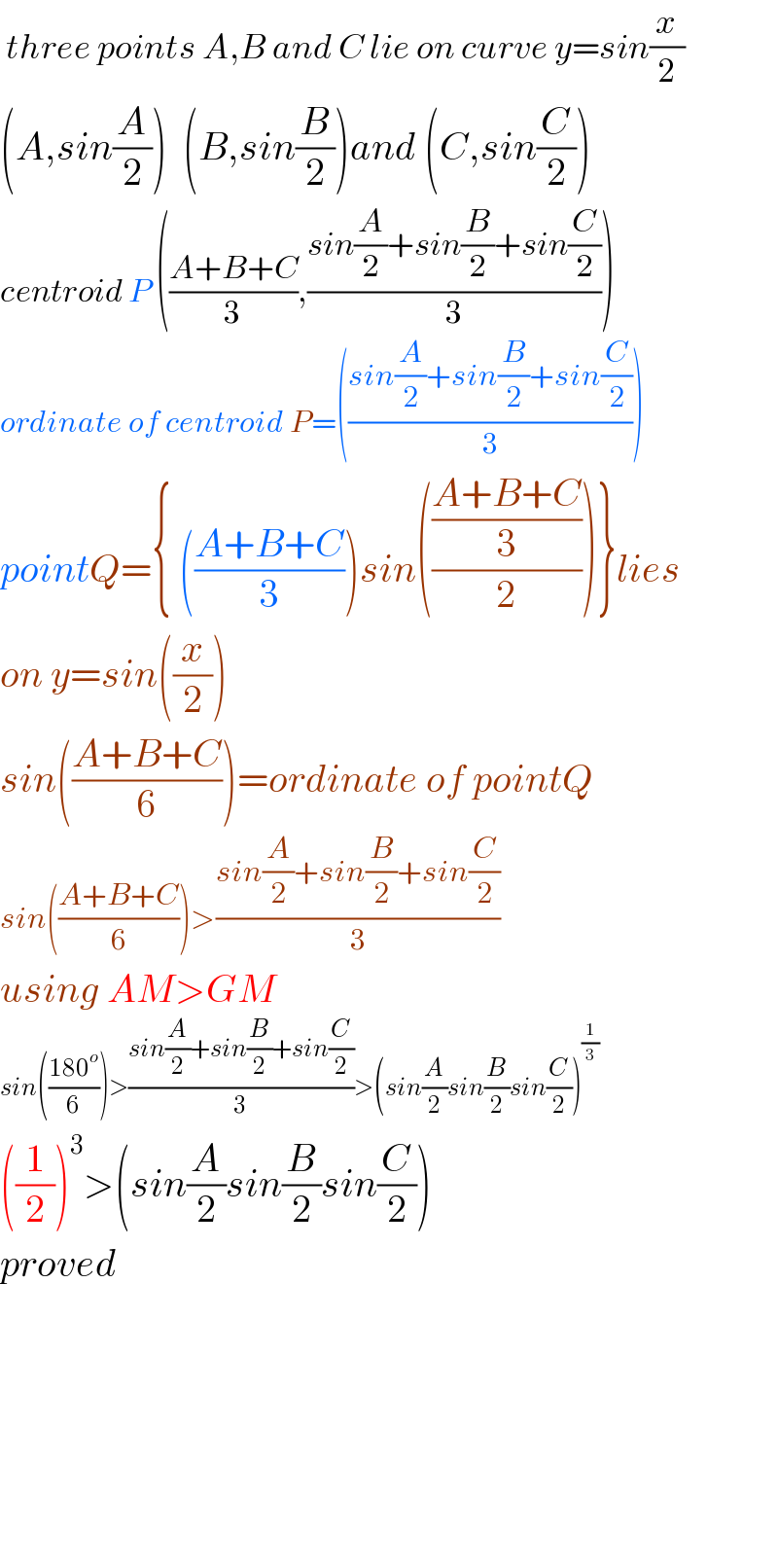
three points A,B and C lie on curve y=sin(x/2) (A,sin(A/2)) (B,sin(B/2))and (C,sin(C/2)) centroid P (((A+B+C)/3),((sin(A/2)+sin(B/2)+sin(C/2))/3)) ordinate of centroid P=(((sin(A/2)+sin(B/2)+sin(C/2))/3)) pointQ={ (((A+B+C)/3))sin((((A+B+C)/3)/2))}lies on y=sin((x/2)) sin(((A+B+C)/6))=ordinate of pointQ sin(((A+B+C)/6))>((sin(A/2)+sin(B/2)+sin(C/2))/3) using AM>GM sin(((180^o )/6))>((sin(A/2)+sin(B/2)+sin(C/2))/3)>(sin(A/2)sin(B/2)sin(C/2))^(1/3) ((1/2))^3 >(sin(A/2)sin(B/2)sin(C/2)) proved

$$\:{three}\:{points}\:{A},{B}\:{and}\:{C}\:{lie}\:{on}\:{curve}\:{y}={sin}\frac{{x}}{\mathrm{2}} \\ $$$$\left({A},{sin}\frac{{A}}{\mathrm{2}}\right)\:\:\left({B},{sin}\frac{{B}}{\mathrm{2}}\right){and}\:\left({C},{sin}\frac{{C}}{\mathrm{2}}\right) \\ $$$${centroid}\:{P}\:\left(\frac{{A}+{B}+{C}}{\mathrm{3}},\frac{{sin}\frac{{A}}{\mathrm{2}}+{sin}\frac{{B}}{\mathrm{2}}+{sin}\frac{{C}}{\mathrm{2}}}{\mathrm{3}}\right) \\ $$$${ordinate}\:{of}\:{centroid}\:{P}=\left(\frac{{sin}\frac{{A}}{\mathrm{2}}+{sin}\frac{{B}}{\mathrm{2}}+{sin}\frac{{C}}{\mathrm{2}}}{\mathrm{3}}\right) \\ $$$${pointQ}=\left\{\:\left(\frac{{A}+{B}+{C}}{\mathrm{3}}\right){sin}\left(\frac{\frac{{A}+{B}+{C}}{\mathrm{3}}}{\mathrm{2}}\right)\right\}{lies} \\ $$$${on}\:{y}={sin}\left(\frac{{x}}{\mathrm{2}}\right) \\ $$$${sin}\left(\frac{{A}+{B}+{C}}{\mathrm{6}}\right)={ordinate}\:{of}\:{pointQ} \\ $$$${sin}\left(\frac{{A}+{B}+{C}}{\mathrm{6}}\right)>\frac{{sin}\frac{{A}}{\mathrm{2}}+{sin}\frac{{B}}{\mathrm{2}}+{sin}\frac{{C}}{\mathrm{2}}}{\mathrm{3}} \\ $$$${using}\:{AM}>{GM} \\ $$$${sin}\left(\frac{\mathrm{180}^{{o}} }{\mathrm{6}}\right)>\frac{{sin}\frac{{A}}{\mathrm{2}}+{sin}\frac{{B}}{\mathrm{2}}+{sin}\frac{{C}}{\mathrm{2}}}{\mathrm{3}}>\left({sin}\frac{{A}}{\mathrm{2}}{sin}\frac{{B}}{\mathrm{2}}{sin}\frac{{C}}{\mathrm{2}}\right)^{\frac{\mathrm{1}}{\mathrm{3}}} \\ $$$$\left(\frac{\mathrm{1}}{\mathrm{2}}\right)^{\mathrm{3}} >\left({sin}\frac{{A}}{\mathrm{2}}{sin}\frac{{B}}{\mathrm{2}}{sin}\frac{{C}}{\mathrm{2}}\right) \\ $$$${proved} \\ $$$$ \\ $$$$ \\ $$$$ \\ $$$$ \\ $$$$ \\ $$

Commented by TANMAY PANACEA last updated on 18/Nov/20

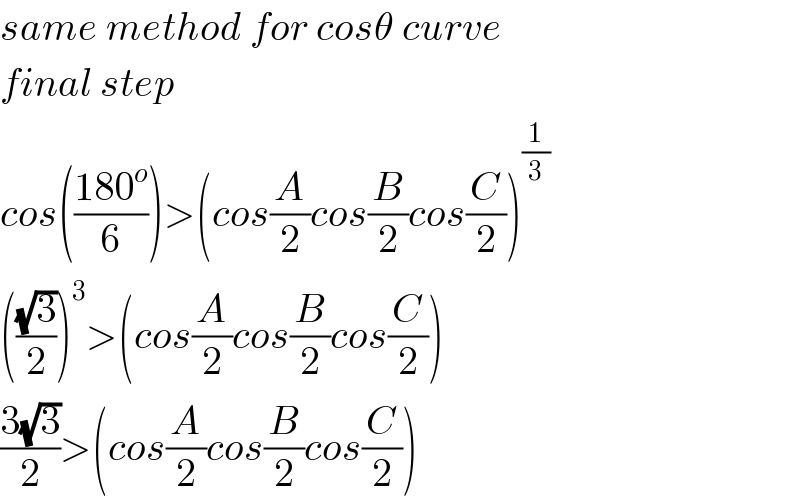
same method for cosθ curve final step cos(((180^o )/6))>(cos(A/2)cos(B/2)cos(C/2))^(1/3) (((√3)/2))^3 >(cos(A/2)cos(B/2)cos(C/2)) ((3(√3))/2)>(cos(A/2)cos(B/2)cos(C/2))

$${same}\:{method}\:{for}\:{cos}\theta\:{curve} \\ $$$${final}\:{step} \\ $$$${cos}\left(\frac{\mathrm{180}^{{o}} }{\mathrm{6}}\right)>\left({cos}\frac{{A}}{\mathrm{2}}{cos}\frac{{B}}{\mathrm{2}}{cos}\frac{{C}}{\mathrm{2}}\right)^{\frac{\mathrm{1}}{\mathrm{3}}} \\ $$$$\left(\frac{\sqrt{\mathrm{3}}}{\mathrm{2}}\right)^{\mathrm{3}} >\left({cos}\frac{{A}}{\mathrm{2}}{cos}\frac{{B}}{\mathrm{2}}{cos}\frac{{C}}{\mathrm{2}}\right) \\ $$$$\frac{\mathrm{3}\sqrt{\mathrm{3}}}{\mathrm{2}}>\left({cos}\frac{{A}}{\mathrm{2}}{cos}\frac{{B}}{\mathrm{2}}{cos}\frac{{C}}{\mathrm{2}}\right) \\ $$

Commented by mnjuly1970 last updated on 18/Nov/20

thank you sir tanmay... excellent...

$${thank}\:{you}\:{sir}\:{tanmay}... \\ $$$$\:\:{excellent}... \\ $$

Commented by TANMAY PANACEA last updated on 18/Nov/20

most welcome

$${most}\:{welcome} \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com