Question and Answers Forum

All Questions      Topic List

Limits Questions

Previous in All Question      Next in All Question      

Previous in Limits      Next in Limits      

Question Number 205774 by mnjuly1970 last updated on 30/Mar/24

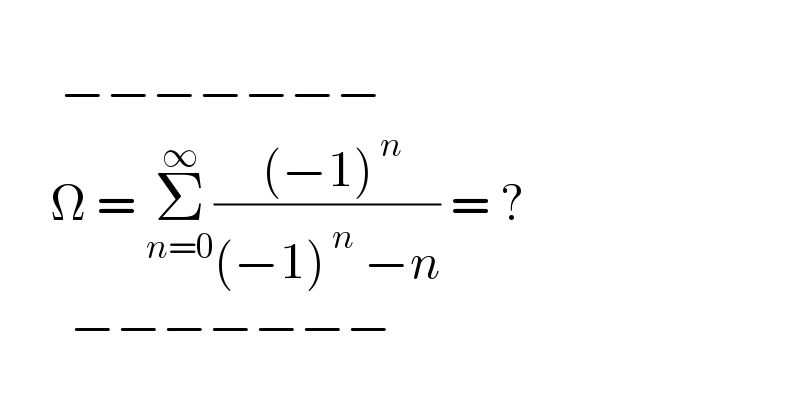
−−−−−−− Ω = Σ_(n=0) ^∞ (( (−1)^( n) )/((−1)^( n) −n)) = ? −−−−−−−

$$ \\ $$$$\:\:\:\:\:\:−−−−−−− \\ $$$$\:\:\:\:\:\Omega\:=\:\underset{{n}=\mathrm{0}} {\overset{\infty} {\sum}}\frac{\:\left(−\mathrm{1}\right)^{\:{n}} }{\left(−\mathrm{1}\right)^{\:{n}} \:−{n}}\:=\:?\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\: \\ $$$$\:\:\:\:\:\:\:−−−−−−− \\ $$

Answered by Berbere last updated on 30/Mar/24

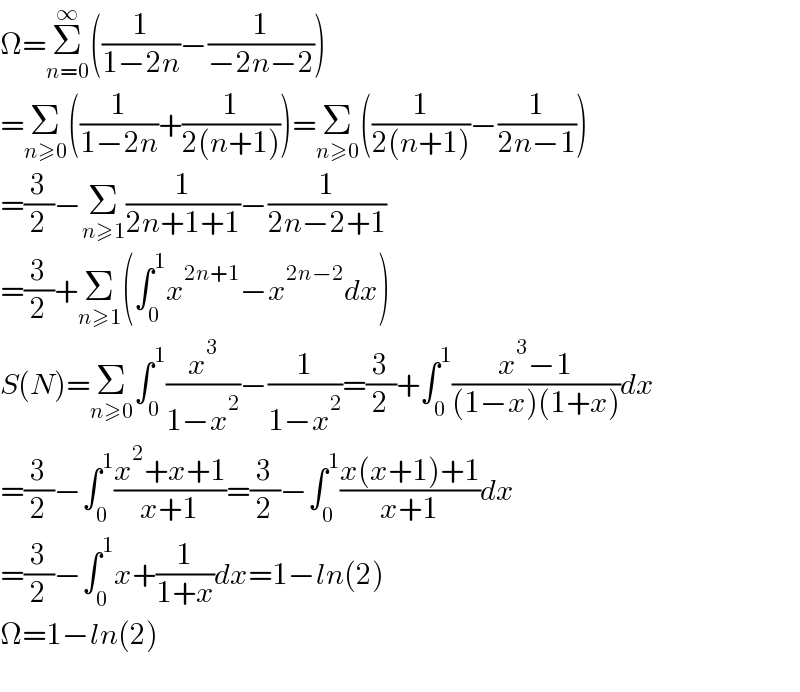
Ω=Σ_(n=0) ^∞ ((1/(1−2n))−(1/(−2n−2))) =Σ_(n≥0) ((1/(1−2n))+(1/(2(n+1))))=Σ_(n≥0) ((1/(2(n+1)))−(1/(2n−1))) =(3/2)−Σ_(n≥1) (1/(2n+1+1))−(1/(2n−2+1)) =(3/2)+Σ_(n≥1) (∫_0 ^1 x^(2n+1) −x^(2n−2) dx) S(N)=Σ_(n≥0) ∫_0 ^1 (x^3 /(1−x^2 ))−(1/(1−x^2 ))=(3/2)+∫_0 ^1 ((x^3 −1)/((1−x)(1+x)))dx =(3/2)−∫_0 ^1 ((x^2 +x+1)/(x+1))=(3/2)−∫_0 ^1 ((x(x+1)+1)/(x+1))dx =(3/2)−∫_0 ^1 x+(1/(1+x))dx=1−ln(2) Ω=1−ln(2)

$$\Omega=\underset{{n}=\mathrm{0}} {\overset{\infty} {\sum}}\left(\frac{\mathrm{1}}{\mathrm{1}−\mathrm{2}{n}}−\frac{\mathrm{1}}{−\mathrm{2}{n}−\mathrm{2}}\right) \\ $$$$=\underset{{n}\geqslant\mathrm{0}} {\sum}\left(\frac{\mathrm{1}}{\mathrm{1}−\mathrm{2}{n}}+\frac{\mathrm{1}}{\mathrm{2}\left({n}+\mathrm{1}\right)}\right)=\underset{{n}\geqslant\mathrm{0}} {\sum}\left(\frac{\mathrm{1}}{\mathrm{2}\left({n}+\mathrm{1}\right)}−\frac{\mathrm{1}}{\mathrm{2}{n}−\mathrm{1}}\right) \\ $$$$=\frac{\mathrm{3}}{\mathrm{2}}−\underset{{n}\geqslant\mathrm{1}} {\sum}\frac{\mathrm{1}}{\mathrm{2}{n}+\mathrm{1}+\mathrm{1}}−\frac{\mathrm{1}}{\mathrm{2}{n}−\mathrm{2}+\mathrm{1}} \\ $$$$=\frac{\mathrm{3}}{\mathrm{2}}+\underset{{n}\geqslant\mathrm{1}} {\sum}\left(\int_{\mathrm{0}} ^{\mathrm{1}} {x}^{\mathrm{2}{n}+\mathrm{1}} −{x}^{\mathrm{2}{n}−\mathrm{2}} {dx}\right) \\ $$$${S}\left({N}\right)=\underset{{n}\geqslant\mathrm{0}} {\sum}\int_{\mathrm{0}} ^{\mathrm{1}} \frac{{x}^{\mathrm{3}} }{\mathrm{1}−{x}^{\mathrm{2}} }−\frac{\mathrm{1}}{\mathrm{1}−{x}^{\mathrm{2}} }=\frac{\mathrm{3}}{\mathrm{2}}+\int_{\mathrm{0}} ^{\mathrm{1}} \frac{{x}^{\mathrm{3}} −\mathrm{1}}{\left(\mathrm{1}−{x}\right)\left(\mathrm{1}+{x}\right)}{dx} \\ $$$$=\frac{\mathrm{3}}{\mathrm{2}}−\int_{\mathrm{0}} ^{\mathrm{1}} \frac{{x}^{\mathrm{2}} +{x}+\mathrm{1}}{{x}+\mathrm{1}}=\frac{\mathrm{3}}{\mathrm{2}}−\int_{\mathrm{0}} ^{\mathrm{1}} \frac{{x}\left({x}+\mathrm{1}\right)+\mathrm{1}}{{x}+\mathrm{1}}{dx} \\ $$$$=\frac{\mathrm{3}}{\mathrm{2}}−\int_{\mathrm{0}} ^{\mathrm{1}} {x}+\frac{\mathrm{1}}{\mathrm{1}+{x}}{dx}=\mathrm{1}−{ln}\left(\mathrm{2}\right) \\ $$$$\Omega=\mathrm{1}−{ln}\left(\mathrm{2}\right) \\ $$$$ \\ $$

Commented by mnjuly1970 last updated on 30/Mar/24

so good solution

$${so}\:{good}\:{solution} \\ $$

Answered by MM42 last updated on 30/Mar/24

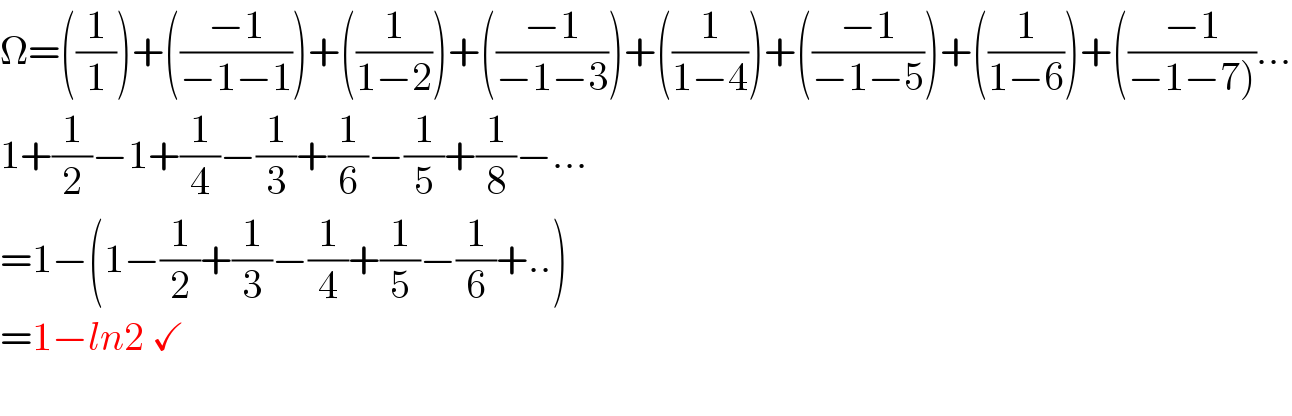
Ω=((1/1))+(((−1)/(−1−1)))+((1/(1−2)))+(((−1)/(−1−3)))+((1/(1−4)))+(((−1)/(−1−5)))+((1/(1−6)))+(((−1)/(−1−7)))... 1+(1/2)−1+(1/4)−(1/3)+(1/6)−(1/5)+(1/8)−... =1−(1−(1/2)+(1/3)−(1/4)+(1/5)−(1/6)+..) =1−ln2 ✓

$$\Omega=\left(\frac{\mathrm{1}}{\mathrm{1}}\right)+\left(\frac{−\mathrm{1}}{−\mathrm{1}−\mathrm{1}}\right)+\left(\frac{\mathrm{1}}{\mathrm{1}−\mathrm{2}}\right)+\left(\frac{−\mathrm{1}}{−\mathrm{1}−\mathrm{3}}\right)+\left(\frac{\mathrm{1}}{\mathrm{1}−\mathrm{4}}\right)+\left(\frac{−\mathrm{1}}{−\mathrm{1}−\mathrm{5}}\right)+\left(\frac{\mathrm{1}}{\mathrm{1}−\mathrm{6}}\right)+\left(\frac{−\mathrm{1}}{\left.−\mathrm{1}−\mathrm{7}\right)}...\right. \\ $$$$\mathrm{1}+\frac{\mathrm{1}}{\mathrm{2}}−\mathrm{1}+\frac{\mathrm{1}}{\mathrm{4}}−\frac{\mathrm{1}}{\mathrm{3}}+\frac{\mathrm{1}}{\mathrm{6}}−\frac{\mathrm{1}}{\mathrm{5}}+\frac{\mathrm{1}}{\mathrm{8}}−... \\ $$$$=\mathrm{1}−\left(\mathrm{1}−\frac{\mathrm{1}}{\mathrm{2}}+\frac{\mathrm{1}}{\mathrm{3}}−\frac{\mathrm{1}}{\mathrm{4}}+\frac{\mathrm{1}}{\mathrm{5}}−\frac{\mathrm{1}}{\mathrm{6}}+..\right) \\ $$$$=\mathrm{1}−{ln}\mathrm{2}\:\checkmark \\ $$$$ \\ $$

Commented by mnjuly1970 last updated on 30/Mar/24

thanks alot

$${thanks}\:{alot} \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com