
Question Number 192604 by sciencestudentW last updated on 22/May/23

$${many}\:{people}\:{say}\:{that}\:{the}\:\mathrm{0}^{\mathrm{0}\:} {is}\:{an}\:{uninfinity} \\ $$$${ones}\:{of}\:{them}\:{say}\:{that}\:\mathrm{0}^{\mathrm{0}} \:{is}\:{infinity}\:{and}\:{equal}\:{to}\:\mathrm{1}! \\ $$$${what}\:{do}\:{you}\:{think}\:{wich}\:{ones}\:{of}\:{them} \\ $$$${say}\:{right}? \\ $$
Commented by Frix last updated on 22/May/23
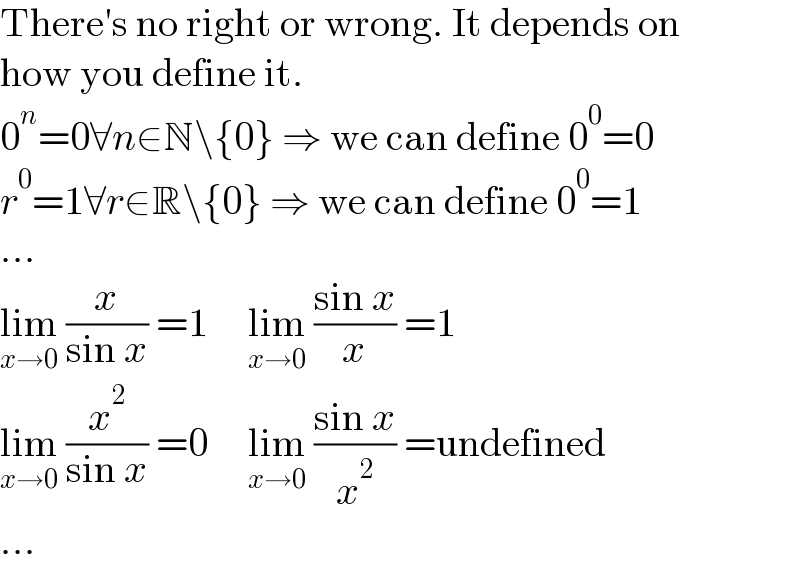
$$\mathrm{There}'\mathrm{s}\:\mathrm{no}\:\mathrm{right}\:\mathrm{or}\:\mathrm{wrong}.\:\mathrm{It}\:\mathrm{depends}\:\mathrm{on} \\ $$$$\mathrm{how}\:\mathrm{you}\:\mathrm{define}\:\mathrm{it}. \\ $$$$\mathrm{0}^{{n}} =\mathrm{0}\forall{n}\in\mathbb{N}\backslash\left\{\mathrm{0}\right\}\:\Rightarrow\:\mathrm{we}\:\mathrm{can}\:\mathrm{define}\:\mathrm{0}^{\mathrm{0}} =\mathrm{0} \\ $$$${r}^{\mathrm{0}} =\mathrm{1}\forall{r}\in\mathbb{R}\backslash\left\{\mathrm{0}\right\}\:\Rightarrow\:\mathrm{we}\:\mathrm{can}\:\mathrm{define}\:\mathrm{0}^{\mathrm{0}} =\mathrm{1} \\ $$$$... \\ $$$$\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\:\frac{{x}}{\mathrm{sin}\:{x}}\:=\mathrm{1}\:\:\:\:\:\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\:\frac{\mathrm{sin}\:{x}}{{x}}\:=\mathrm{1} \\ $$$$\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\:\frac{{x}^{\mathrm{2}} }{\mathrm{sin}\:{x}}\:=\mathrm{0}\:\:\:\:\:\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\:\frac{\mathrm{sin}\:{x}}{{x}^{\mathrm{2}} }\:=\mathrm{undefined} \\ $$$$... \\ $$
