Question and Answers Forum

All Questions      Topic List

Algebra Questions

Previous in All Question      Next in All Question      

Previous in Algebra      Next in Algebra      

Question Number 17775 by gourav~ last updated on 10/Jul/17

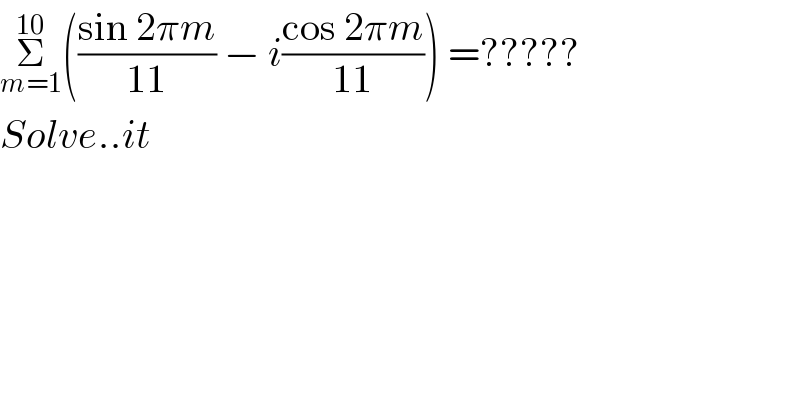
Σ_(m=1) ^(10) (((sin 2πm)/(11)) − i((cos 2πm)/(11))) =????? Solve..it

$$\underset{{m}=\mathrm{1}} {\overset{\mathrm{10}} {\sum}}\left(\frac{\mathrm{sin}\:\mathrm{2}\pi{m}}{\mathrm{11}}\:−\:{i}\frac{\mathrm{cos}\:\mathrm{2}\pi{m}}{\mathrm{11}}\right)\:=????? \\ $$$${Solve}..{it} \\ $$$$ \\ $$

Answered by alex041103 last updated on 10/Jul/17

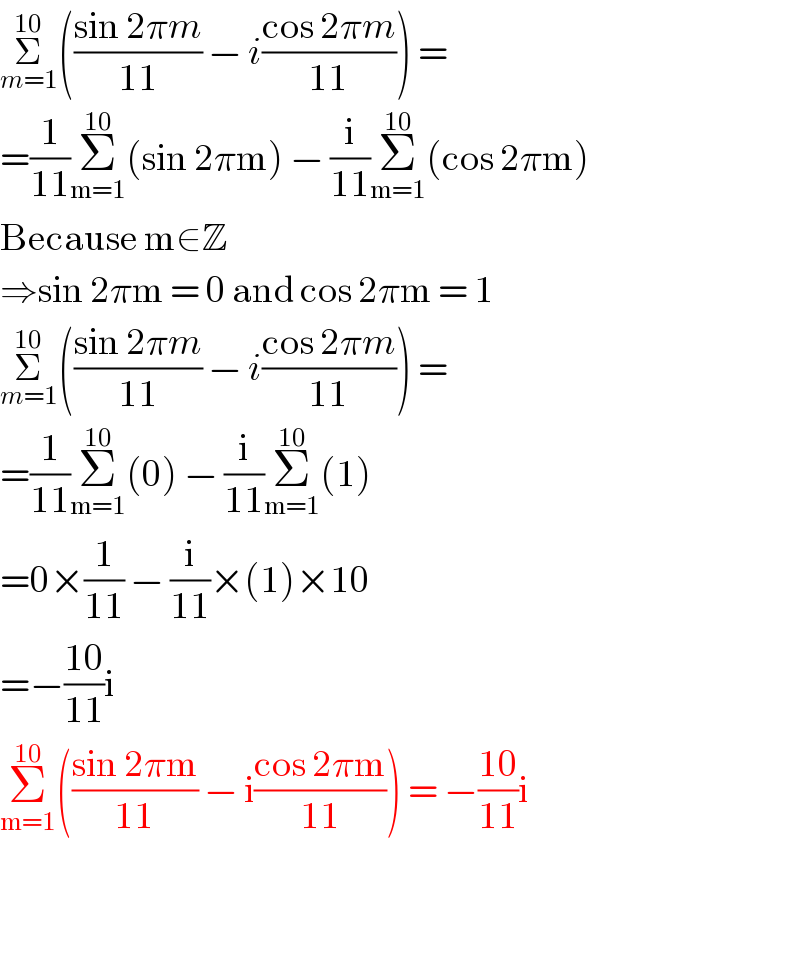
Σ_(m=1) ^(10) (((sin 2πm)/(11)) − i((cos 2πm)/(11))) = =(1/(11))Σ_(m=1) ^(10) (sin 2πm) − (i/(11))Σ_(m=1) ^(10) (cos 2πm) Because m∈Z ⇒sin 2πm = 0 and cos 2πm = 1 Σ_(m=1) ^(10) (((sin 2πm)/(11)) − i((cos 2πm)/(11))) = =(1/(11))Σ_(m=1) ^(10) (0) − (i/(11))Σ_(m=1) ^(10) (1) =0×(1/(11)) − (i/(11))×(1)×10 =−((10)/(11))i Σ_(m=1) ^(10) (((sin 2πm)/(11)) − i((cos 2πm)/(11))) = −((10)/(11))i

$$\underset{{m}=\mathrm{1}} {\overset{\mathrm{10}} {\sum}}\left(\frac{\mathrm{sin}\:\mathrm{2}\pi{m}}{\mathrm{11}}\:−\:{i}\frac{\mathrm{cos}\:\mathrm{2}\pi{m}}{\mathrm{11}}\right)\:= \\ $$$$=\frac{\mathrm{1}}{\mathrm{11}}\underset{\mathrm{m}=\mathrm{1}} {\overset{\mathrm{10}} {\sum}}\left(\mathrm{sin}\:\mathrm{2}\pi\mathrm{m}\right)\:−\:\frac{\mathrm{i}}{\mathrm{11}}\underset{\mathrm{m}=\mathrm{1}} {\overset{\mathrm{10}} {\sum}}\left(\mathrm{cos}\:\mathrm{2}\pi\mathrm{m}\right) \\ $$$$\mathrm{Because}\:\mathrm{m}\in\mathbb{Z}\: \\ $$$$\Rightarrow\mathrm{sin}\:\mathrm{2}\pi\mathrm{m}\:=\:\mathrm{0}\:\mathrm{and}\:\mathrm{cos}\:\mathrm{2}\pi\mathrm{m}\:=\:\mathrm{1} \\ $$$$\underset{{m}=\mathrm{1}} {\overset{\mathrm{10}} {\sum}}\left(\frac{\mathrm{sin}\:\mathrm{2}\pi{m}}{\mathrm{11}}\:−\:{i}\frac{\mathrm{cos}\:\mathrm{2}\pi{m}}{\mathrm{11}}\right)\:= \\ $$$$=\frac{\mathrm{1}}{\mathrm{11}}\underset{\mathrm{m}=\mathrm{1}} {\overset{\mathrm{10}} {\sum}}\left(\mathrm{0}\right)\:−\:\frac{\mathrm{i}}{\mathrm{11}}\underset{\mathrm{m}=\mathrm{1}} {\overset{\mathrm{10}} {\sum}}\left(\mathrm{1}\right) \\ $$$$=\mathrm{0}×\frac{\mathrm{1}}{\mathrm{11}}\:−\:\frac{\mathrm{i}}{\mathrm{11}}×\left(\mathrm{1}\right)×\mathrm{10} \\ $$$$=−\frac{\mathrm{10}}{\mathrm{11}}\mathrm{i} \\ $$$$\underset{\mathrm{m}=\mathrm{1}} {\overset{\mathrm{10}} {\sum}}\left(\frac{\mathrm{sin}\:\mathrm{2}\pi\mathrm{m}}{\mathrm{11}}\:−\:\mathrm{i}\frac{\mathrm{cos}\:\mathrm{2}\pi\mathrm{m}}{\mathrm{11}}\right)\:=\:−\frac{\mathrm{10}}{\mathrm{11}}\mathrm{i} \\ $$$$ \\ $$$$ \\ $$

Answered by ajfour last updated on 10/Jul/17

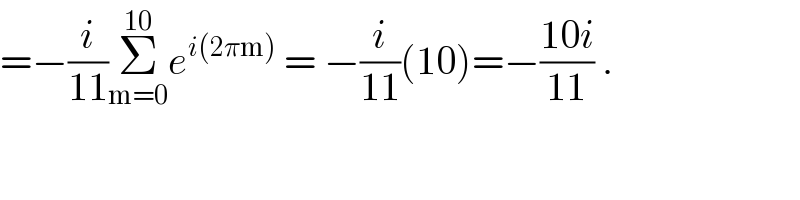
=−(i/(11))Σ_(m=0) ^(10) e^(i(2πm)) = −(i/(11))(10)=−((10i)/(11)) .

$$=−\frac{{i}}{\mathrm{11}}\underset{\mathrm{m}=\mathrm{0}} {\overset{\mathrm{10}} {\sum}}{e}^{{i}\left(\mathrm{2}\pi\mathrm{m}\right)} \:=\:−\frac{{i}}{\mathrm{11}}\left(\mathrm{10}\right)=−\frac{\mathrm{10}{i}}{\mathrm{11}}\:. \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com