
Question Number 122303 by Dwaipayan Shikari last updated on 15/Nov/20
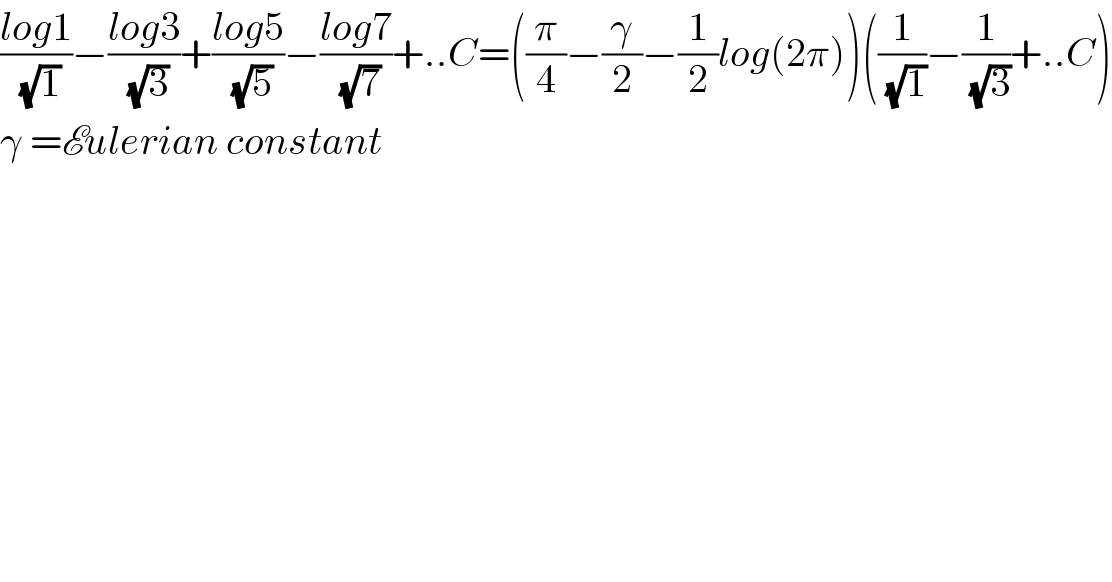
$$\frac{{log}\mathrm{1}}{\:\sqrt{\mathrm{1}}}−\frac{{log}\mathrm{3}}{\:\sqrt{\mathrm{3}}}+\frac{{log}\mathrm{5}}{\:\sqrt{\mathrm{5}}}−\frac{{log}\mathrm{7}}{\:\sqrt{\mathrm{7}}}+..{C}=\left(\frac{\pi}{\mathrm{4}}−\frac{\gamma}{\mathrm{2}}−\frac{\mathrm{1}}{\mathrm{2}}{log}\left(\mathrm{2}\pi\right)\right)\left(\frac{\mathrm{1}}{\:\sqrt{\mathrm{1}}}−\frac{\mathrm{1}}{\:\sqrt{\mathrm{3}}}+..{C}\right) \\ $$$$\gamma\:=\mathscr{E}{ulerian}\:{constant} \\ $$
Commented by rs4089 last updated on 15/Nov/20
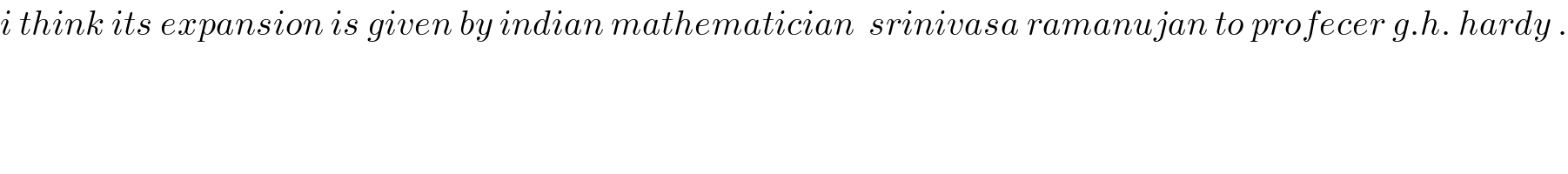
$${i}\:{think}\:{its}\:{expansion}\:{is}\:{given}\:{by}\:{indian}\:{mathematician}\:\:{srinivasa}\:{ramanujan}\:{to}\:{profecer}\:{g}.{h}.\:{hardy}\:. \\ $$
Commented by Dwaipayan Shikari last updated on 15/Nov/20
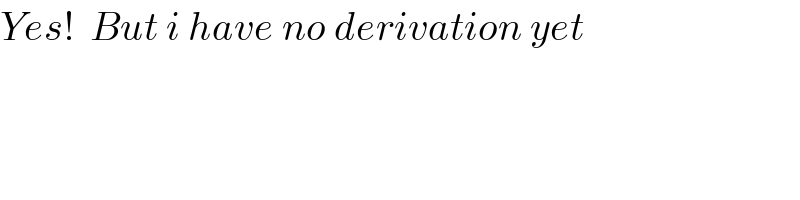
$${Yes}!\:\:{But}\:{i}\:{have}\:{no}\:{derivation}\:{yet} \\ $$
Commented by Dwaipayan Shikari last updated on 15/Nov/20
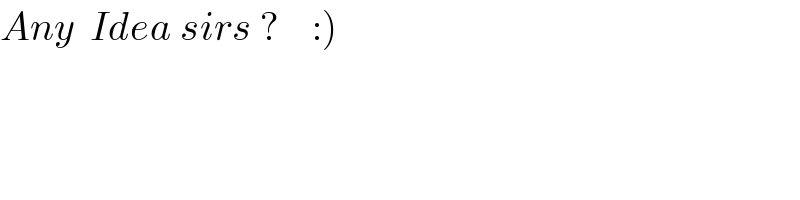
$$\left.{Any}\:\:{Idea}\:{sirs}\:?\:\:\:\::\right) \\ $$
Answered by mindispower last updated on 15/Nov/20

$$\zeta'\left({s}\right) \\ $$$$=\Sigma\frac{−{ln}\left({n}\right)}{{n}^{{s}} },{seeems}\:{s}=\frac{\mathrm{1}}{\mathrm{2}} \\ $$$${worcking}\:{on}\:{it}\: \\ $$
