
Question Number 121653 by Dwaipayan Shikari last updated on 10/Nov/20
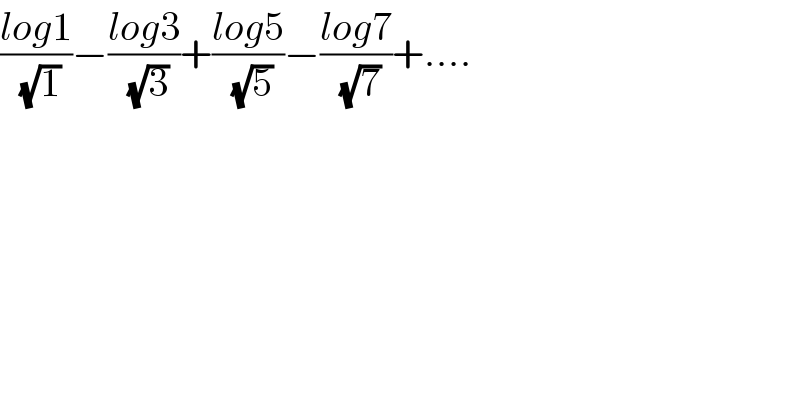
$$\frac{{log}\mathrm{1}}{\:\sqrt{\mathrm{1}}}−\frac{{log}\mathrm{3}}{\:\sqrt{\mathrm{3}}}+\frac{{log}\mathrm{5}}{\:\sqrt{\mathrm{5}}}−\frac{{log}\mathrm{7}}{\:\sqrt{\mathrm{7}}}+.... \\ $$
Commented by TANMAY PANACEA last updated on 10/Nov/20
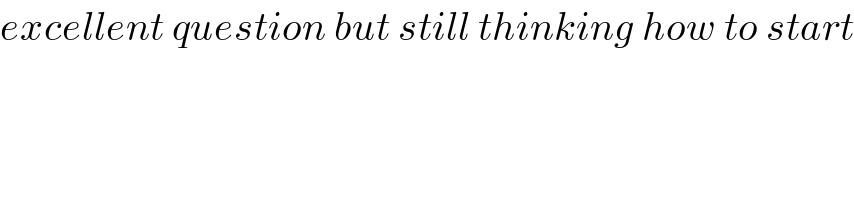
$${excellent}\:{question}\:{but}\:{still}\:{thinking}\:{how}\:{to}\:{start} \\ $$
Commented by Dwaipayan Shikari last updated on 10/Nov/20
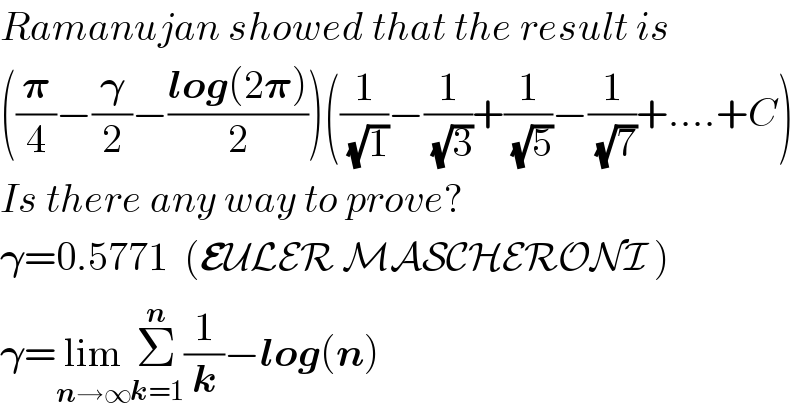
$${Ramanujan}\:{showed}\:{that}\:{the}\:{result}\:{is}\: \\ $$$$\left(\frac{\boldsymbol{\pi}}{\mathrm{4}}−\frac{\boldsymbol{\gamma}}{\mathrm{2}}−\frac{\boldsymbol{{log}}\left(\mathrm{2}\boldsymbol{\pi}\right)}{\mathrm{2}}\right)\left(\frac{\mathrm{1}}{\:\sqrt{\mathrm{1}}}−\frac{\mathrm{1}}{\:\sqrt{\mathrm{3}}}+\frac{\mathrm{1}}{\:\sqrt{\mathrm{5}}}−\frac{\mathrm{1}}{\:\sqrt{\mathrm{7}}}+....+{C}\right) \\ $$$${Is}\:{there}\:{any}\:{way}\:{to}\:{prove}? \\ $$$$\boldsymbol{\gamma}=\mathrm{0}.\mathrm{5771}\:\:\left(\boldsymbol{\mathcal{E}}\mathcal{ULER}\:\mathcal{MASCHERONI}\:\right) \\ $$$$\boldsymbol{\gamma}=\underset{\boldsymbol{{n}}\rightarrow\infty} {\mathrm{lim}}\underset{\boldsymbol{{k}}=\mathrm{1}} {\overset{\boldsymbol{{n}}} {\sum}}\frac{\mathrm{1}}{\boldsymbol{{k}}}−\boldsymbol{{log}}\left(\boldsymbol{{n}}\right) \\ $$
Commented by TANMAY PANACEA last updated on 10/Nov/20

$${excellent}...{did}\:{you}\:{heard}\:\:{name}\:{of}\:{Mr}.{Hardy} \\ $$
Commented by TANMAY PANACEA last updated on 10/Nov/20

Commented by Dwaipayan Shikari last updated on 10/Nov/20

$${Yes}\:{sir}! \\ $$
