
Question Number 130406 by john_santu last updated on 25/Jan/21
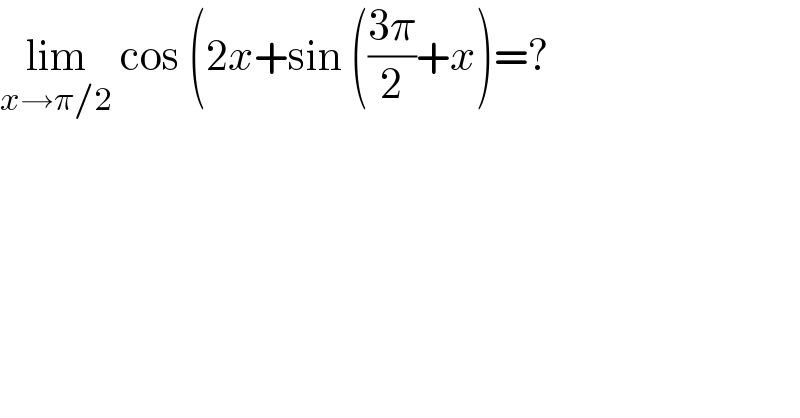
$$\underset{{x}\rightarrow\pi/\mathrm{2}} {\mathrm{lim}}\:\mathrm{cos}\:\left(\mathrm{2}{x}+\mathrm{sin}\:\left(\frac{\mathrm{3}\pi}{\mathrm{2}}+{x}\right)=?\right. \\ $$
Commented by Dwaipayan Shikari last updated on 25/Jan/21

$$−\mathrm{1} \\ $$
Answered by EDWIN88 last updated on 25/Jan/21
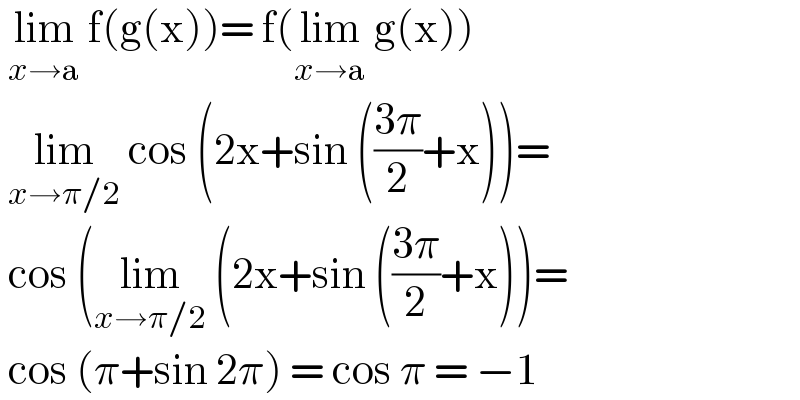
$$\:\underset{{x}\rightarrow\mathrm{a}} {\mathrm{lim}}\:\mathrm{f}\left(\mathrm{g}\left(\mathrm{x}\right)\right)=\:\mathrm{f}\left(\underset{{x}\rightarrow\mathrm{a}} {\mathrm{lim}}\:\mathrm{g}\left(\mathrm{x}\right)\right) \\ $$$$\:\underset{{x}\rightarrow\pi/\mathrm{2}} {\mathrm{lim}}\:\mathrm{cos}\:\left(\mathrm{2x}+\mathrm{sin}\:\left(\frac{\mathrm{3}\pi}{\mathrm{2}}+\mathrm{x}\right)\right)= \\ $$$$\:\mathrm{cos}\:\left(\underset{{x}\rightarrow\pi/\mathrm{2}} {\mathrm{lim}}\:\left(\mathrm{2x}+\mathrm{sin}\:\left(\frac{\mathrm{3}\pi}{\mathrm{2}}+\mathrm{x}\right)\right)=\right. \\ $$$$\:\mathrm{cos}\:\left(\pi+\mathrm{sin}\:\mathrm{2}\pi\right)\:=\:\mathrm{cos}\:\pi\:=\:−\mathrm{1} \\ $$
Answered by mathmax by abdo last updated on 25/Jan/21
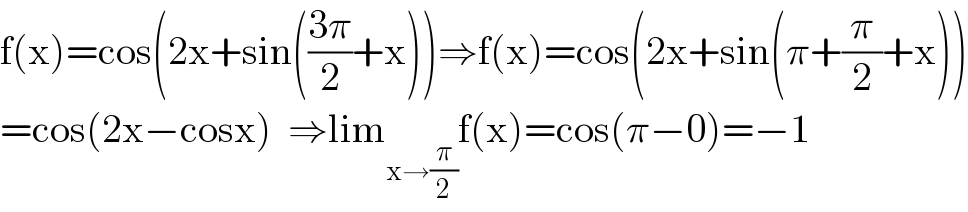
$$\mathrm{f}\left(\mathrm{x}\right)=\mathrm{cos}\left(\mathrm{2x}+\mathrm{sin}\left(\frac{\mathrm{3}\pi}{\mathrm{2}}+\mathrm{x}\right)\right)\Rightarrow\mathrm{f}\left(\mathrm{x}\right)=\mathrm{cos}\left(\mathrm{2x}+\mathrm{sin}\left(\pi+\frac{\pi}{\mathrm{2}}+\mathrm{x}\right)\right) \\ $$$$=\mathrm{cos}\left(\mathrm{2x}−\mathrm{cosx}\right)\:\:\Rightarrow\mathrm{lim}_{\mathrm{x}\rightarrow\frac{\pi}{\mathrm{2}}} \mathrm{f}\left(\mathrm{x}\right)=\mathrm{cos}\left(\pi−\mathrm{0}\right)=−\mathrm{1} \\ $$
