Question and Answers Forum

All Questions      Topic List

Limits Questions

Previous in All Question      Next in All Question      

Previous in Limits      Next in Limits      

Question Number 27723 by NECx last updated on 13/Jan/18

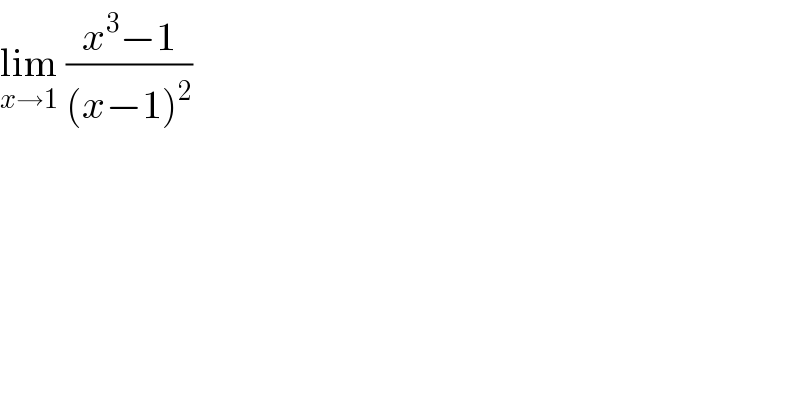
lim_(x→1) ((x^3 −1)/((x−1)^2 ))

$$\underset{{x}\rightarrow\mathrm{1}} {\mathrm{lim}}\:\frac{{x}^{\mathrm{3}} −\mathrm{1}}{\left({x}−\mathrm{1}\right)^{\mathrm{2}} } \\ $$

Answered by prakash jain last updated on 13/Jan/18

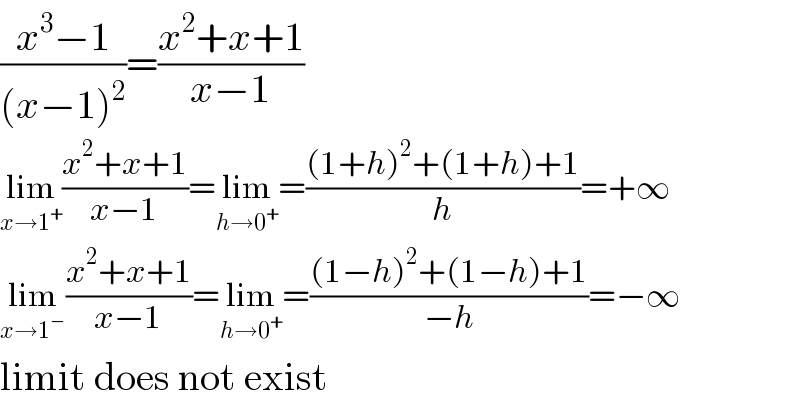
((x^3 −1)/((x−1)^2 ))=((x^2 +x+1)/(x−1)) lim_(x→1^+ ) ((x^2 +x+1)/(x−1))=lim_(h→0^+ ) =(((1+h)^2 +(1+h)+1)/h)=+∞ lim_(x→1^− ) ((x^2 +x+1)/(x−1))=lim_(h→0^+ ) =(((1−h)^2 +(1−h)+1)/(−h))=−∞ limit does not exist

$$\frac{{x}^{\mathrm{3}} −\mathrm{1}}{\left({x}−\mathrm{1}\right)^{\mathrm{2}} }=\frac{{x}^{\mathrm{2}} +{x}+\mathrm{1}}{{x}−\mathrm{1}} \\ $$$$\underset{{x}\rightarrow\mathrm{1}^{+} } {\mathrm{lim}}\frac{{x}^{\mathrm{2}} +{x}+\mathrm{1}}{{x}−\mathrm{1}}=\underset{{h}\rightarrow\mathrm{0}^{+} } {\mathrm{lim}}=\frac{\left(\mathrm{1}+{h}\right)^{\mathrm{2}} +\left(\mathrm{1}+{h}\right)+\mathrm{1}}{{h}}=+\infty \\ $$$$\underset{{x}\rightarrow\mathrm{1}^{−} } {\mathrm{lim}}\frac{{x}^{\mathrm{2}} +{x}+\mathrm{1}}{{x}−\mathrm{1}}=\underset{{h}\rightarrow\mathrm{0}^{+} } {\mathrm{lim}}=\frac{\left(\mathrm{1}−{h}\right)^{\mathrm{2}} +\left(\mathrm{1}−{h}\right)+\mathrm{1}}{−{h}}=−\infty \\ $$$$\mathrm{limit}\:\mathrm{does}\:\mathrm{not}\:\mathrm{exist} \\ $$

Commented by NECx last updated on 13/Jan/18

thanks

$${thanks} \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com