
Question Number 86675 by M±th+et£s last updated on 30/Mar/20
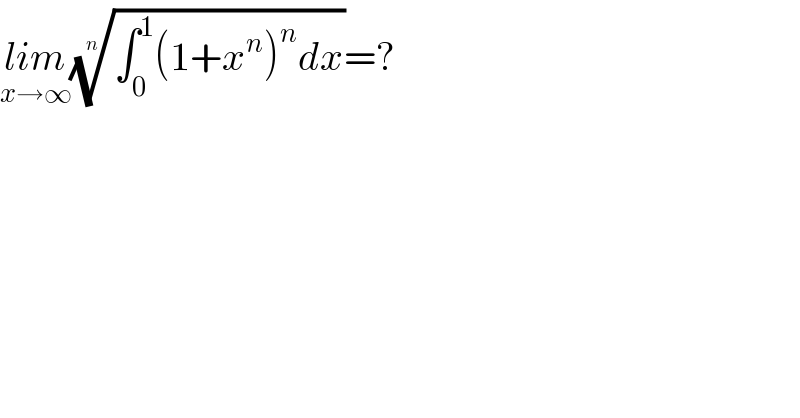
$$\underset{{x}\rightarrow\infty} {{lim}}\sqrt[{{n}}]{\int_{\mathrm{0}} ^{\mathrm{1}} \left(\mathrm{1}+{x}^{{n}} \right)^{{n}} {dx}}=? \\ $$
Commented by M±th+et£s last updated on 31/Mar/20

$$\underset{{n}\rightarrow\infty} {{lim}}\ast\ast\ast \\ $$
Commented by mr W last updated on 30/Mar/20

$$=\mathrm{1} \\ $$
Commented by redmiiuser last updated on 30/Mar/20

$${how}\:{sir}? \\ $$
Commented by mr W last updated on 30/Mar/20

$${through}\:{thinking}... \\ $$
Commented by mathmax by abdo last updated on 31/Mar/20
![let take a try A_n =(∫_0 ^1 (1+x^n )^n dx)^(1/n) ⇒ ln(A_n ) =(1/n)∫_0 ^1 (1+x^n )^n dx =(1/n) ∫_0 ^(1 ) ( Σ_(k=0) ^n x^(nk) )dx =(1/n)Σ_(k=0) ^n (1/(nk+1))[x^(nk+1) ]_0 ^1 dx =(1/n)Σ_(k=0) ^n (1/(nk+1)) x disappear something went wrong in the Q...!](Q86756.png)
$${let}\:{take}\:{a}\:{try}\:\:{A}_{{n}} =\left(\int_{\mathrm{0}} ^{\mathrm{1}} \left(\mathrm{1}+{x}^{{n}} \right)^{{n}} {dx}\right)^{\frac{\mathrm{1}}{{n}}} \:\Rightarrow \\ $$$${ln}\left({A}_{{n}} \right)\:=\frac{\mathrm{1}}{{n}}\int_{\mathrm{0}} ^{\mathrm{1}} \:\left(\mathrm{1}+{x}^{{n}} \right)^{{n}} \:{dx}\:=\frac{\mathrm{1}}{{n}}\:\int_{\mathrm{0}} ^{\mathrm{1}\:} \left(\:\sum_{{k}=\mathrm{0}} ^{{n}} \:{x}^{{nk}} \right){dx} \\ $$$$=\frac{\mathrm{1}}{{n}}\sum_{{k}=\mathrm{0}} ^{{n}} \:\:\frac{\mathrm{1}}{{nk}+\mathrm{1}}\left[{x}^{{nk}+\mathrm{1}} \right]_{\mathrm{0}} ^{\mathrm{1}} \:{dx}\:=\frac{\mathrm{1}}{{n}}\sum_{{k}=\mathrm{0}} ^{{n}} \:\frac{\mathrm{1}}{{nk}+\mathrm{1}}\:\:{x}\:{disappear} \\ $$$${something}\:{went}\:{wrong}\:{in}\:{the}\:{Q}...! \\ $$
Commented by mr W last updated on 31/Mar/20
![should it not be: ln(A_n ) =(1/n)ln [∫_0 ^1 (1+x^n )^n dx] instead of ln(A_n ) =(1/n)∫_0 ^1 (1+x^n )^n dx ?](Q86823.png)
$${should}\:{it}\:{not}\:{be}: \\ $$$${ln}\left({A}_{{n}} \right)\:=\frac{\mathrm{1}}{{n}}\mathrm{ln}\:\left[\int_{\mathrm{0}} ^{\mathrm{1}} \:\left(\mathrm{1}+{x}^{{n}} \right)^{{n}} \:{dx}\right]\:{instead}\:{of} \\ $$$${ln}\left({A}_{{n}} \right)\:=\frac{\mathrm{1}}{{n}}\int_{\mathrm{0}} ^{\mathrm{1}} \:\left(\mathrm{1}+{x}^{{n}} \right)^{{n}} \:{dx}\:? \\ $$
Commented by mathmax by abdo last updated on 01/Apr/20

$${yes}\:{sir}. \\ $$
Answered by redmiiuser last updated on 30/Mar/20
![(1+x^n )^n =Σ_(k=0) ^n .C_k ^n .(x^n )^k ∫_0 ^1 Σ_(k=0) ^n .C_k ^n .x^(nk) .dx =Σ_(k=0) ^n .C_k ^n .[(x^(nk+1) /(nk+1))]_0 ^1 =Σ_(k=0) ^n .C_(k ) ^n .[(1/(nk+1))] ∴ [Σ_(k=0) ^n .C_k ^n .[(1/(nk+1))]]^((1/n)) (/)answer](Q86676.png)
$$\left(\mathrm{1}+{x}^{{n}} \right)^{{n}} \\ $$$$=\underset{{k}=\mathrm{0}} {\overset{{n}} {\sum}}.\underset{{k}} {\overset{{n}} {{C}}}.\left({x}^{{n}} \right)^{{k}} \\ $$$$\underset{\mathrm{0}} {\overset{\mathrm{1}} {\int}}\underset{{k}=\mathrm{0}} {\overset{{n}} {\sum}}.\underset{{k}} {\overset{{n}} {{C}}}.{x}^{{nk}} .{dx} \\ $$$$=\underset{{k}=\mathrm{0}} {\overset{{n}} {\sum}}.\underset{{k}} {\overset{{n}} {{C}}}.\left[\frac{{x}^{{nk}+\mathrm{1}} }{{nk}+\mathrm{1}}\right]_{\mathrm{0}} ^{\mathrm{1}} \\ $$$$=\underset{{k}=\mathrm{0}} {\overset{{n}} {\sum}}.\underset{{k}\:\:} {\overset{{n}} {{C}}}.\left[\frac{\mathrm{1}}{{nk}+\mathrm{1}}\right] \\ $$$$\therefore\:\left[\underset{{k}=\mathrm{0}} {\overset{{n}} {\sum}}.\underset{{k}} {\overset{{n}} {{C}}}.\left[\frac{\mathrm{1}}{{nk}+\mathrm{1}}\right]\right]^{\left(\mathrm{1}/{n}\right)} \:\frac{}{}{answer} \\ $$
Commented by redmiiuser last updated on 30/Mar/20
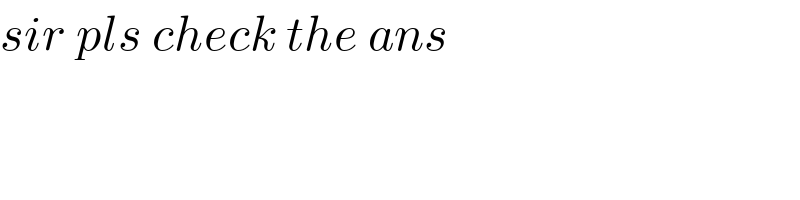
$${sir}\:{pls}\:{check}\:{the}\:{ans} \\ $$
Commented by M±th+et£s last updated on 30/Mar/20
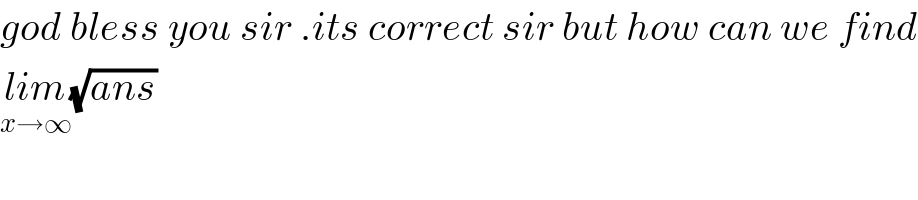
$${god}\:{bless}\:{you}\:{sir}\:.{its}\:{correct}\:{sir}\:{but}\:{how}\:{can}\:{we}\:{find} \\ $$$$\underset{{x}\rightarrow\infty} {{lim}}\sqrt{{ans}} \\ $$
Commented by redmiiuser last updated on 30/Mar/20

$${no}\:{term}\:{of}\:{x}\:{is}\:{present}\:{in} \\ $$$${my}\:{answer} \\ $$$${hence}\:{that}\:{li}\underset{{x}\rightarrow\infty} {{m}}\:{ans} \\ $$$${makes}\:{no}\:{sense}. \\ $$
Commented by M±th+et£s last updated on 30/Mar/20
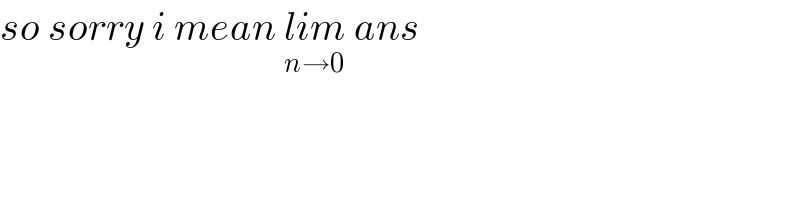
$${so}\:{sorry}\:{i}\:{mean}\:\underset{{n}\rightarrow\mathrm{0}} {{lim}}\:{ans} \\ $$
Commented by M±th+et£s last updated on 30/Mar/20

$$\underset{{n}\rightarrow\infty} {{lim}}\ast\ast\ast \\ $$
Commented by redmiiuser last updated on 30/Mar/20
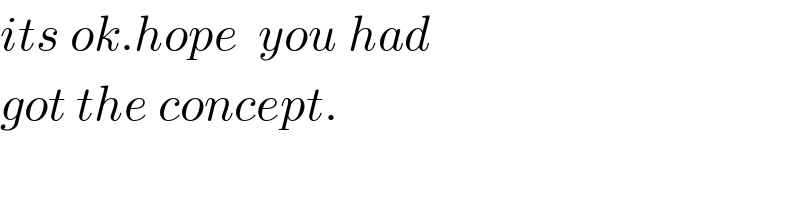
$${its}\:{ok}.{hope}\:\:{you}\:{had} \\ $$$${got}\:{the}\:{concept}. \\ $$
Commented by redmiiuser last updated on 30/Mar/20

$${ok}.{now}\:\:{i}\:{got}\:{it}. \\ $$
Commented by redmiiuser last updated on 30/Mar/20
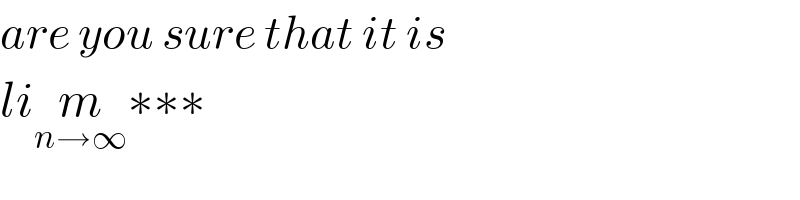
$${are}\:{you}\:{sure}\:{that}\:{it}\:{is} \\ $$$${li}\underset{{n}\rightarrow\infty} {{m}}\ast\ast\ast \\ $$
Commented by M±th+et£s last updated on 30/Mar/20

$${yes}\:{sir} \\ $$
Commented by redmiiuser last updated on 30/Mar/20
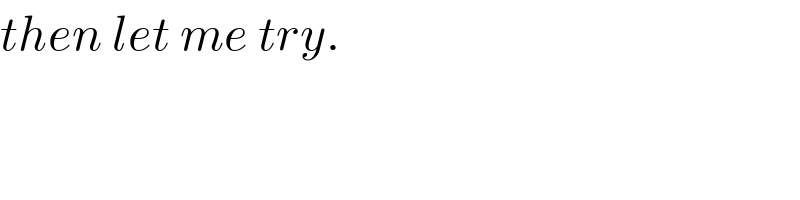
$${then}\:{let}\:{me}\:{try}. \\ $$
Commented by redmiiuser last updated on 30/Mar/20
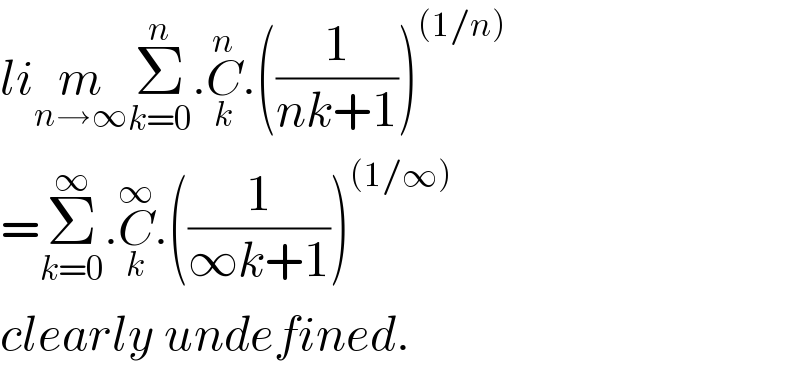
$${li}\underset{{n}\rightarrow\infty} {{m}}\underset{{k}=\mathrm{0}} {\overset{{n}} {\sum}}.\underset{{k}} {\overset{{n}} {{C}}}.\left(\frac{\mathrm{1}}{{nk}+\mathrm{1}}\right)^{\left(\mathrm{1}/{n}\right)} \\ $$$$=\underset{{k}=\mathrm{0}} {\overset{\infty} {\sum}}.\underset{{k}} {\overset{\infty} {{C}}}.\left(\frac{\mathrm{1}}{\infty{k}+\mathrm{1}}\right)^{\left(\mathrm{1}/\infty\right)} \\ $$$${clearly}\:{undefined}. \\ $$
Commented by redmiiuser last updated on 30/Mar/20
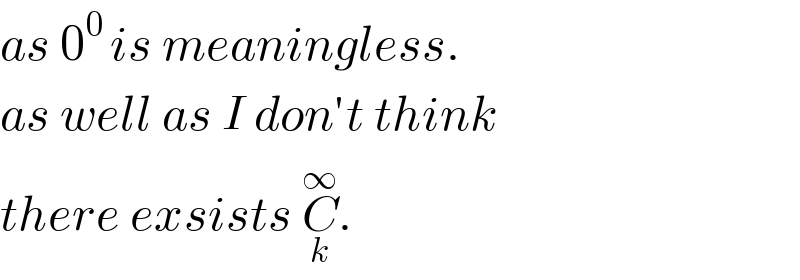
$${as}\:\mathrm{0}^{\mathrm{0}\:} {is}\:{meaningless}. \\ $$$${as}\:{well}\:{as}\:{I}\:{don}'{t}\:{think} \\ $$$${there}\:{exsists}\:\underset{{k}} {\overset{\infty} {{C}}}. \\ $$
Commented by redmiiuser last updated on 30/Mar/20
$${sir}\:{can}\:{you}\:{check}\:{it}? \\ $$
