
Previous in Relation and Functions Next in Relation and Functions
Question Number 78667 by berket last updated on 19/Jan/20
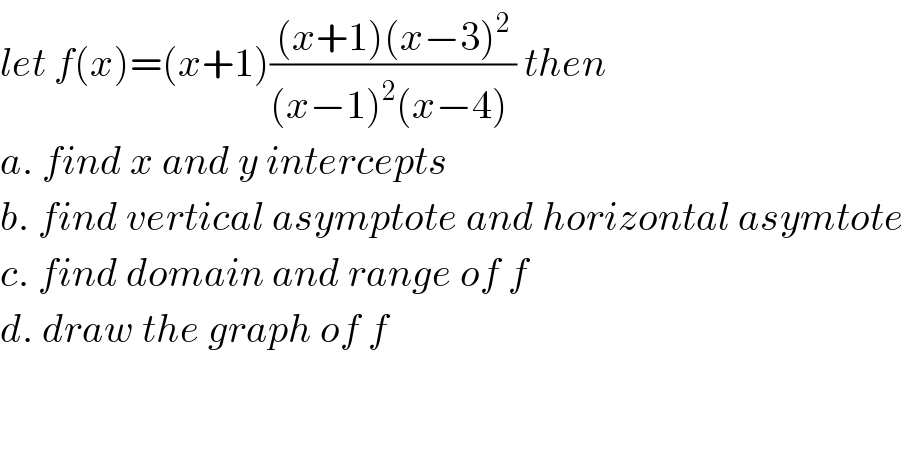
$${let}\:{f}\left({x}\right)=\left({x}+\mathrm{1}\right)\frac{\left({x}+\mathrm{1}\right)\left({x}−\mathrm{3}\right)^{\mathrm{2}} }{\left({x}−\mathrm{1}\right)^{\mathrm{2}} \left({x}−\mathrm{4}\right)\:}\:{then} \\ $$$${a}.\:{find}\:{x}\:{and}\:{y}\:{intercepts} \\ $$$${b}.\:{find}\:{vertical}\:{asymptote}\:{and}\:{horizontal}\:{asymtote} \\ $$$${c}.\:{find}\:{domain}\:{and}\:{range}\:{of}\:{f} \\ $$$${d}.\:{draw}\:{the}\:{graph}\:{of}\:{f} \\ $$
Answered by Rio Michael last updated on 19/Jan/20
![a. let y = (((x+1)^2 (x−3)^2 )/((x−1)^2 (x−4))) y−intercept , x = 0 y = (((0+1)^2 (0−3)^2 )/((0−1)^2 (0−4)^(2 ) )) = (9/(16)) point (0,(9/(16))) x−intercept, y = 0 0 = (((x+1)^2 (x−3)^2 )/((x−1)^2 (x−4)^2 )) [(x + 1)(x−3)]^2 = 0 x = −1 or x = 3 point (−1,0) and (3,0) b. Vertical asymptotes (x−1)^2 (x−4)=0 either (x−1)^2 =0 ⇒ x = 1 ∨ x = 4 Horizontal asymptote lim_(x→∞) f(x) = ∞ hence no horizontal asymptote. c. domain = vertical asympotes](Q78681.png)
$${a}.\:\:{let}\:{y}\:=\:\frac{\left({x}+\mathrm{1}\right)^{\mathrm{2}} \left({x}−\mathrm{3}\right)^{\mathrm{2}} }{\left({x}−\mathrm{1}\right)^{\mathrm{2}} \left({x}−\mathrm{4}\right)} \\ $$$${y}−{intercept}\:\:,\:{x}\:=\:\mathrm{0} \\ $$$$\:{y}\:=\:\frac{\left(\mathrm{0}+\mathrm{1}\right)^{\mathrm{2}} \left(\mathrm{0}−\mathrm{3}\right)^{\mathrm{2}} }{\left(\mathrm{0}−\mathrm{1}\right)^{\mathrm{2}} \left(\mathrm{0}−\mathrm{4}\right)^{\mathrm{2}\:} }\:=\:\frac{\mathrm{9}}{\mathrm{16}} \\ $$$${point}\:\:\left(\mathrm{0},\frac{\mathrm{9}}{\mathrm{16}}\right) \\ $$$${x}−{intercept},\:{y}\:=\:\mathrm{0} \\ $$$$\mathrm{0}\:=\:\frac{\left({x}+\mathrm{1}\right)^{\mathrm{2}} \left({x}−\mathrm{3}\right)^{\mathrm{2}} }{\left({x}−\mathrm{1}\right)^{\mathrm{2}} \left({x}−\mathrm{4}\right)^{\mathrm{2}} } \\ $$$$\:\left[\left({x}\:+\:\mathrm{1}\right)\left({x}−\mathrm{3}\right)\right]^{\mathrm{2}} =\:\mathrm{0} \\ $$$$\:\:\:{x}\:=\:−\mathrm{1}\:{or}\:{x}\:=\:\mathrm{3} \\ $$$${point}\:\left(−\mathrm{1},\mathrm{0}\right)\:{and}\:\:\left(\mathrm{3},\mathrm{0}\right) \\ $$$${b}.\:{Vertical}\:{asymptotes}\:\:\left({x}−\mathrm{1}\right)^{\mathrm{2}} \left({x}−\mathrm{4}\right)=\mathrm{0} \\ $$$${either}\:\left({x}−\mathrm{1}\right)^{\mathrm{2}} =\mathrm{0}\:\Rightarrow\:{x}\:=\:\mathrm{1}\:\vee\:{x}\:=\:\mathrm{4} \\ $$$${Horizontal}\:{asymptote}\: \\ $$$$\:\underset{{x}\rightarrow\infty} {\mathrm{lim}}\:{f}\left({x}\right)\:=\:\infty\:{hence}\:{no}\:{horizontal}\:{asymptote}. \\ $$$${c}.\:{domain}\:=\:{vertical}\:{asympotes} \\ $$$$ \\ $$$$ \\ $$
