
Question Number 54936 by maxmathsup by imad last updated on 14/Feb/19
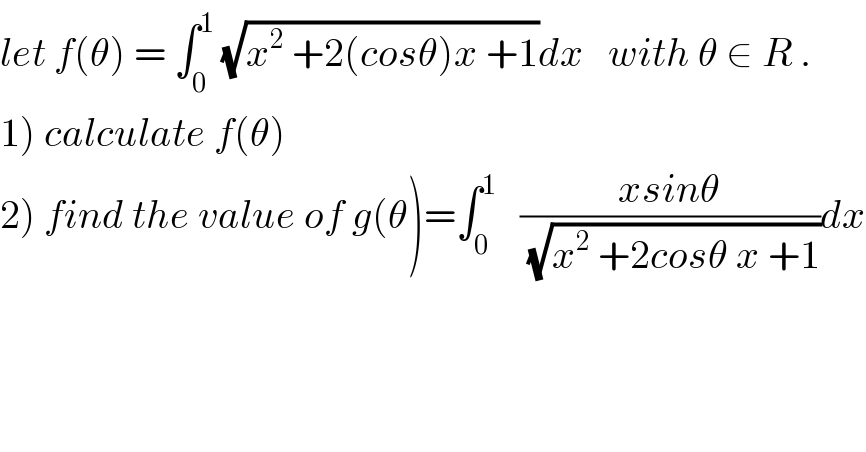
$${let}\:{f}\left(\theta\right)\:=\:\int_{\mathrm{0}} ^{\mathrm{1}} \:\sqrt{{x}^{\mathrm{2}} \:+\mathrm{2}\left({cos}\theta\right){x}\:+\mathrm{1}}{dx}\:\:\:{with}\:\theta\:\in\:{R}\:. \\ $$$$\left.\mathrm{1}\right)\:{calculate}\:{f}\left(\theta\right) \\ $$$$\left.\mathrm{2}\right)\:{find}\:{the}\:{value}\:{of}\:{g}\left(\theta\right)=\int_{\mathrm{0}} ^{\mathrm{1}} \:\:\:\frac{{xsin}\theta}{\sqrt{{x}^{\mathrm{2}} \:+\mathrm{2}{cos}\theta\:{x}\:+\mathrm{1}}}{dx} \\ $$
Commented by Abdo msup. last updated on 16/Feb/19
![1) we have f(θ)=∫_0 ^1 (√(x^(2 ) +2cosθ x +1))dx =∫_0 ^1 (√((x+cosθ)^2 +sin^2 θ))dθ chang.x+cosθ=sinθ t give f(θ)=∫_(coatanθ) ^(cotan((θ/2))) ∣sinθ∣sinθ (√(1+t^2 ))dt f(θ)=∣sinθ∣sinθ ∫_(cotan(θ)) ^(cotan((θ/2))) (√(1+t^2 ))dt let find ∫ (√(1+t^2 ))dt changement t=sh(u)give ∫ (√(1+t^2 ))dt =∫ ch(u)ch(u)du =∫ ch^2 (u)du =∫ ((1+ch(2u))/2)du =(u/2) +(1/4)sh(2u) =(u/2) +(1/2)ch(u)sh(u) =(1/2)argsh(t) +(1/2)t(√(1+t^2 )) +c =(1/2)ln(t +(√(1+t^2 ))) +(t/2)(√(1+t^2 )) ⇒ ∫_(cotan(θ)) ^(cotan((θ/2))) (√(1+t^2 ))dt =(1/2)[ln(t+(√(1+t^2 ))) +t(√(1+t^2 ))]_(cotanθ) ^(cotan((θ/2))) =(1/2){ln(cotan((θ/2))+(√(1+cotan^2 ((θ/2))))) +cotan((θ/2))(√(1+cotan^2 ((θ/2))))−ln(cotanθ+(√(1+cotanθ)) −cotanθ(√(1+cotan^2 θ)))](Q55053.png)
$$\left.\mathrm{1}\right)\:{we}\:{have}\:{f}\left(\theta\right)=\int_{\mathrm{0}} ^{\mathrm{1}} \sqrt{{x}^{\mathrm{2}\:} +\mathrm{2}{cos}\theta\:{x}\:+\mathrm{1}}{dx} \\ $$$$=\int_{\mathrm{0}} ^{\mathrm{1}} \sqrt{\left({x}+{cos}\theta\right)^{\mathrm{2}} \:+{sin}^{\mathrm{2}} \theta}{d}\theta\:\:{chang}.{x}+{cos}\theta={sin}\theta\:{t} \\ $$$${give}\:{f}\left(\theta\right)=\int_{{coatan}\theta} ^{{cotan}\left(\frac{\theta}{\mathrm{2}}\right)} \:\mid{sin}\theta\mid{sin}\theta\:\sqrt{\mathrm{1}+{t}^{\mathrm{2}} }{dt} \\ $$$${f}\left(\theta\right)=\mid{sin}\theta\mid{sin}\theta\:\:\int_{{cotan}\left(\theta\right)} ^{{cotan}\left(\frac{\theta}{\mathrm{2}}\right)} \sqrt{\mathrm{1}+{t}^{\mathrm{2}} }{dt} \\ $$$${let}\:{find}\:\int\:\:\sqrt{\mathrm{1}+{t}^{\mathrm{2}} }{dt}\:\:\:{changement}\:{t}={sh}\left({u}\right){give} \\ $$$$\int\:\:\sqrt{\mathrm{1}+{t}^{\mathrm{2}} }{dt}\:=\int\:{ch}\left({u}\right){ch}\left({u}\right){du} \\ $$$$=\int\:{ch}^{\mathrm{2}} \left({u}\right){du}\:=\int\:\:\frac{\mathrm{1}+{ch}\left(\mathrm{2}{u}\right)}{\mathrm{2}}{du} \\ $$$$=\frac{{u}}{\mathrm{2}}\:+\frac{\mathrm{1}}{\mathrm{4}}{sh}\left(\mathrm{2}{u}\right)\:=\frac{{u}}{\mathrm{2}}\:+\frac{\mathrm{1}}{\mathrm{2}}{ch}\left({u}\right){sh}\left({u}\right) \\ $$$$=\frac{\mathrm{1}}{\mathrm{2}}{argsh}\left({t}\right)\:+\frac{\mathrm{1}}{\mathrm{2}}{t}\sqrt{\mathrm{1}+{t}^{\mathrm{2}} }\:+{c} \\ $$$$=\frac{\mathrm{1}}{\mathrm{2}}{ln}\left({t}\:+\sqrt{\mathrm{1}+{t}^{\mathrm{2}} }\right)\:+\frac{{t}}{\mathrm{2}}\sqrt{\mathrm{1}+{t}^{\mathrm{2}} }\:\Rightarrow \\ $$$$\int_{{cotan}\left(\theta\right)} ^{{cotan}\left(\frac{\theta}{\mathrm{2}}\right)} \:\sqrt{\mathrm{1}+{t}^{\mathrm{2}} }{dt}\:=\frac{\mathrm{1}}{\mathrm{2}}\left[{ln}\left({t}+\sqrt{\mathrm{1}+{t}^{\mathrm{2}} }\right)\:+{t}\sqrt{\mathrm{1}+{t}^{\mathrm{2}} }\right]_{{cotan}\theta} ^{{cotan}\left(\frac{\theta}{\mathrm{2}}\right)} \\ $$$$=\frac{\mathrm{1}}{\mathrm{2}}\left\{{ln}\left({cotan}\left(\frac{\theta}{\mathrm{2}}\right)+\sqrt{\mathrm{1}+{cotan}^{\mathrm{2}} \left(\frac{\theta}{\mathrm{2}}\right)}\right)\right. \\ $$$$+{cotan}\left(\frac{\theta}{\mathrm{2}}\right)\sqrt{\mathrm{1}+{cotan}^{\mathrm{2}} \left(\frac{\theta}{\mathrm{2}}\right)}−{ln}\left({cotan}\theta+\sqrt{\mathrm{1}+{cotan}\theta}\right. \\ $$$$\left.−{cotan}\theta\sqrt{\mathrm{1}+{cotan}^{\mathrm{2}} \theta}\right) \\ $$$$ \\ $$
Answered by tanmay.chaudhury50@gmail.com last updated on 15/Feb/19
![∫(√(x^2 +2xcosθ+1)) dx ∫(√((x+cosθ)^2 +sin^2 θ)) dx (((x+cosθ))/2)(√((x+cosθ)^2 +sin^2 θ)) +((sin^2 θ)/2)(√((x+cosθ)^2 +sin^2 θ)) +c so answer is ∣(((x+cosθ))/2)(√((x+cosθ)^2 +sin^2 θ)) +((sin^2 θ)/2)(√((x+cosθ)^2 +sin^2 θ)) ∣_0 ^1 [{(((1+cosθ))/2)(√((1+cosθ)^2 +sin^2 θ)) +((sin^2 θ)/2)(√((1+cosθ)^2 +sin^2 θ)) }−{((cosθ)/2)(√(cos^2 θ+sin^2 θ)) +((sin^2 θ)/2)×(√(cos^2 θ+sin^2 θ)) }] [(((1+cosθ))/2)(√(1+2cosθ+1)) +((sin^2 θ)/2)(√(2+2cosθ)) }−{((cosθ)/2)+((sin^2 θ)/2)}] (((1+cosθ))/2)×2cos(θ/2)+((sin^2 θ)/2)×2cos(θ/2)−((cosθ)/2)−((sin^2 θ)/2)] check upto this...](Q54982.png)
$$\int\sqrt{{x}^{\mathrm{2}} +\mathrm{2}{xcos}\theta+\mathrm{1}}\:{dx} \\ $$$$\int\sqrt{\left({x}+{cos}\theta\right)^{\mathrm{2}} +{sin}^{\mathrm{2}} \theta}\:{dx} \\ $$$$\frac{\left({x}+{cos}\theta\right)}{\mathrm{2}}\sqrt{\left({x}+{cos}\theta\right)^{\mathrm{2}} +{sin}^{\mathrm{2}} \theta}\:+\frac{{sin}^{\mathrm{2}} \theta}{\mathrm{2}}\sqrt{\left({x}+{cos}\theta\right)^{\mathrm{2}} +{sin}^{\mathrm{2}} \theta}\:+{c} \\ $$$${so}\:{answer}\:{is} \\ $$$$\mid\frac{\left({x}+{cos}\theta\right)}{\mathrm{2}}\sqrt{\left({x}+{cos}\theta\right)^{\mathrm{2}} +{sin}^{\mathrm{2}} \theta}\:+\frac{{sin}^{\mathrm{2}} \theta}{\mathrm{2}}\sqrt{\left({x}+{cos}\theta\right)^{\mathrm{2}} +{sin}^{\mathrm{2}} \theta}\:\mid_{\mathrm{0}} ^{\mathrm{1}} \\ $$$$\left[\left\{\frac{\left(\mathrm{1}+{cos}\theta\right)}{\mathrm{2}}\sqrt{\left(\mathrm{1}+{cos}\theta\right)^{\mathrm{2}} +{sin}^{\mathrm{2}} \theta}\:+\frac{{sin}^{\mathrm{2}} \theta}{\mathrm{2}}\sqrt{\left(\mathrm{1}+{cos}\theta\right)^{\mathrm{2}} +{sin}^{\mathrm{2}} \theta}\:\right\}−\left\{\frac{{cos}\theta}{\mathrm{2}}\sqrt{{cos}^{\mathrm{2}} \theta+{sin}^{\mathrm{2}} \theta}\:+\frac{{sin}^{\mathrm{2}} \theta}{\mathrm{2}}×\sqrt{{cos}^{\mathrm{2}} \theta+{sin}^{\mathrm{2}} \theta}\:\right\}\right] \\ $$$$\left.\left[\frac{\left(\mathrm{1}+{cos}\theta\right)}{\mathrm{2}}\sqrt{\mathrm{1}+\mathrm{2}{cos}\theta+\mathrm{1}}\:+\frac{{sin}^{\mathrm{2}} \theta}{\mathrm{2}}\sqrt{\mathrm{2}+\mathrm{2}{cos}\theta}\:\right\}−\left\{\frac{{cos}\theta}{\mathrm{2}}+\frac{{sin}^{\mathrm{2}} \theta}{\mathrm{2}}\right\}\right] \\ $$$$\left.\frac{\left(\mathrm{1}+{cos}\theta\right)}{\mathrm{2}}×\mathrm{2}{cos}\frac{\theta}{\mathrm{2}}+\frac{{sin}^{\mathrm{2}} \theta}{\mathrm{2}}×\mathrm{2}{cos}\frac{\theta}{\mathrm{2}}−\frac{{cos}\theta}{\mathrm{2}}−\frac{{sin}^{\mathrm{2}} \theta}{\mathrm{2}}\right] \\ $$$${check}\:\:{upto}\:{this}... \\ $$
