
Previous in Relation and Functions Next in Relation and Functions
Question Number 33359 by caravan msup abdo. last updated on 15/Apr/18
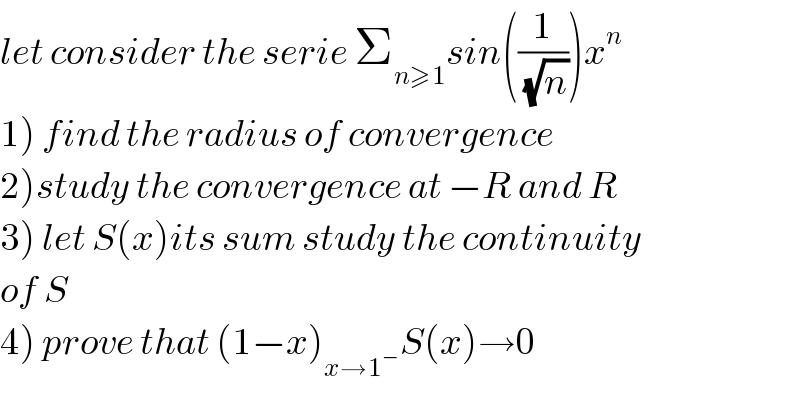
$${let}\:{consider}\:{the}\:{serie}\:\sum_{{n}\geqslant\mathrm{1}} {sin}\left(\frac{\mathrm{1}}{\sqrt{{n}}}\right){x}^{{n}} \\ $$$$\left.\mathrm{1}\right)\:{find}\:{the}\:{radius}\:{of}\:{convergence} \\ $$$$\left.\mathrm{2}\right){study}\:{the}\:{convergence}\:{at}\:−{R}\:{and}\:{R} \\ $$$$\left.\mathrm{3}\right)\:{let}\:{S}\left({x}\right){its}\:{sum}\:{study}\:{the}\:{continuity} \\ $$$${of}\:{S} \\ $$$$\left.\mathrm{4}\right)\:{prove}\:{that}\:\left(\mathrm{1}−{x}\right)_{{x}\rightarrow\mathrm{1}^{−} } {S}\left({x}\right)\rightarrow\mathrm{0} \\ $$
