
Previous in Relation and Functions Next in Relation and Functions
Question Number 64355 by turbo msup by abdo last updated on 17/Jul/19

$${let}\:{F}\left({x}\right)=\int_{{u}\left({x}\right)} ^{{v}\left({x}\left\{\right.\right.} {f}\left({x},{t}\right){dt} \\ $$$${how}\:{to}\:{calculate}\:\:\frac{{dF}}{{dx}}\left({x}\right)? \\ $$
Commented by MJS last updated on 17/Jul/19
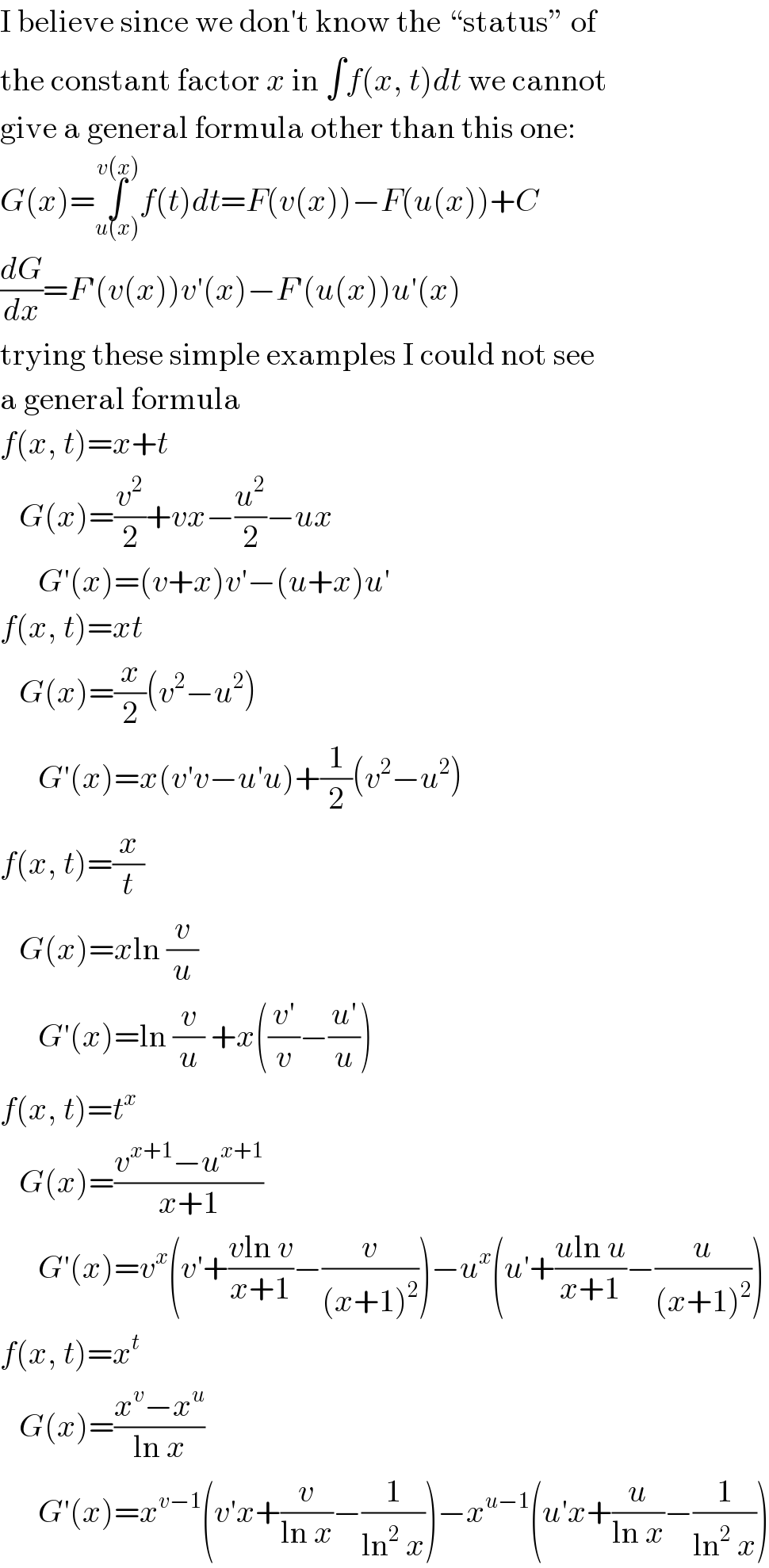
$$\mathrm{I}\:\mathrm{believe}\:\mathrm{since}\:\mathrm{we}\:\mathrm{don}'\mathrm{t}\:\mathrm{know}\:\mathrm{the}\:``\mathrm{status}''\:\mathrm{of} \\ $$$$\mathrm{the}\:\mathrm{constant}\:\mathrm{factor}\:{x}\:\mathrm{in}\:\int{f}\left({x},\:{t}\right){dt}\:\mathrm{we}\:\mathrm{cannot} \\ $$$$\mathrm{give}\:\mathrm{a}\:\mathrm{general}\:\mathrm{formula}\:\mathrm{other}\:\mathrm{than}\:\mathrm{this}\:\mathrm{one}: \\ $$$${G}\left({x}\right)=\underset{{u}\left({x}\right)} {\overset{{v}\left({x}\right)} {\int}}{f}\left({t}\right){dt}={F}\left({v}\left({x}\right)\right)−{F}\left({u}\left({x}\right)\right)+{C} \\ $$$$\frac{{dG}}{{dx}}={F}'\left({v}\left({x}\right)\right){v}'\left({x}\right)−{F}'\left({u}\left({x}\right)\right){u}'\left({x}\right) \\ $$$$\mathrm{trying}\:\mathrm{these}\:\mathrm{simple}\:\mathrm{examples}\:\mathrm{I}\:\mathrm{could}\:\mathrm{not}\:\mathrm{see} \\ $$$$\mathrm{a}\:\mathrm{general}\:\mathrm{formula} \\ $$$${f}\left({x},\:{t}\right)={x}+{t} \\ $$$$\:\:\:{G}\left({x}\right)=\frac{{v}^{\mathrm{2}} }{\mathrm{2}}+{vx}−\frac{{u}^{\mathrm{2}} }{\mathrm{2}}−{ux} \\ $$$$\:\:\:\:\:\:{G}'\left({x}\right)=\left({v}+{x}\right){v}'−\left({u}+{x}\right){u}' \\ $$$${f}\left({x},\:{t}\right)={xt} \\ $$$$\:\:\:{G}\left({x}\right)=\frac{{x}}{\mathrm{2}}\left({v}^{\mathrm{2}} −{u}^{\mathrm{2}} \right) \\ $$$$\:\:\:\:\:\:{G}'\left({x}\right)={x}\left({v}'{v}−{u}'{u}\right)+\frac{\mathrm{1}}{\mathrm{2}}\left({v}^{\mathrm{2}} −{u}^{\mathrm{2}} \right) \\ $$$${f}\left({x},\:{t}\right)=\frac{{x}}{{t}} \\ $$$$\:\:\:{G}\left({x}\right)={x}\mathrm{ln}\:\frac{{v}}{{u}} \\ $$$$\:\:\:\:\:\:{G}'\left({x}\right)=\mathrm{ln}\:\frac{{v}}{{u}}\:+{x}\left(\frac{{v}'}{{v}}−\frac{{u}'}{{u}}\right) \\ $$$${f}\left({x},\:{t}\right)={t}^{{x}} \\ $$$$\:\:\:{G}\left({x}\right)=\frac{{v}^{{x}+\mathrm{1}} −{u}^{{x}+\mathrm{1}} }{{x}+\mathrm{1}} \\ $$$$\:\:\:\:\:\:{G}'\left({x}\right)={v}^{{x}} \left({v}'+\frac{{v}\mathrm{ln}\:{v}}{{x}+\mathrm{1}}−\frac{{v}}{\left({x}+\mathrm{1}\right)^{\mathrm{2}} }\right)−{u}^{{x}} \left({u}'+\frac{{u}\mathrm{ln}\:{u}}{{x}+\mathrm{1}}−\frac{{u}}{\left({x}+\mathrm{1}\right)^{\mathrm{2}} }\right) \\ $$$${f}\left({x},\:{t}\right)={x}^{{t}} \\ $$$$\:\:\:{G}\left({x}\right)=\frac{{x}^{{v}} −{x}^{{u}} }{\mathrm{ln}\:{x}} \\ $$$$\:\:\:\:\:\:{G}'\left({x}\right)={x}^{{v}−\mathrm{1}} \left({v}'{x}+\frac{{v}}{\mathrm{ln}\:{x}}−\frac{\mathrm{1}}{\mathrm{ln}^{\mathrm{2}} \:{x}}\right)−{x}^{{u}−\mathrm{1}} \left({u}'{x}+\frac{{u}}{\mathrm{ln}\:{x}}−\frac{\mathrm{1}}{\mathrm{ln}^{\mathrm{2}} \:{x}}\right) \\ $$
Commented by mathmax by abdo last updated on 17/Jul/19
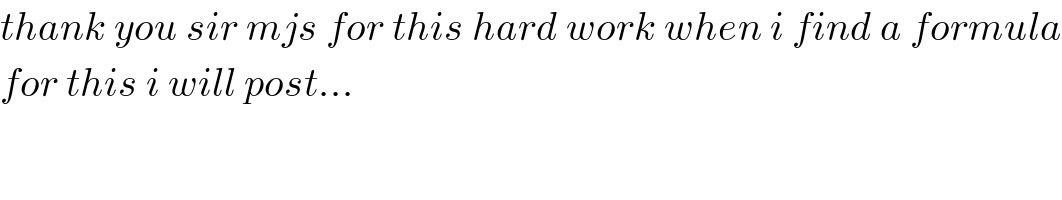
$${thank}\:{you}\:{sir}\:{mjs}\:{for}\:{this}\:{hard}\:{work}\:{when}\:{i}\:{find}\:{a}\:{formula} \\ $$$${for}\:{this}\:{i}\:{will}\:{post}... \\ $$
