
Question Number 34420 by math khazana by abdo last updated on 06/May/18

$${let}\:{A}\:\:=\:{arctanx}\:−{arctany} \\ $$$${give}\:{another}\:{form}\:{of}\:{A}\:{if}\:\:{xy}\neq−\mathrm{1}\:. \\ $$
Commented by math khazana by abdo last updated on 07/May/18
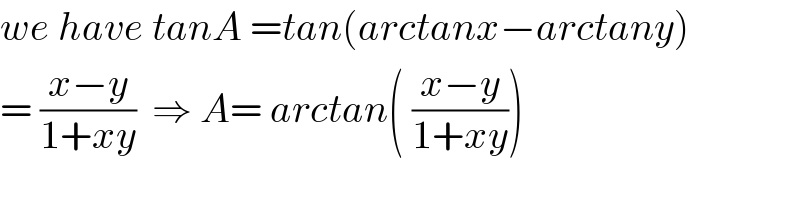
$${we}\:{have}\:{tanA}\:={tan}\left({arctanx}−{arctany}\right) \\ $$$$=\:\frac{{x}−{y}}{\mathrm{1}+{xy}}\:\:\Rightarrow\:{A}=\:{arctan}\left(\:\frac{{x}−{y}}{\mathrm{1}+{xy}}\right) \\ $$
Answered by tanmay.chaudhury50@gmail.com last updated on 07/May/18
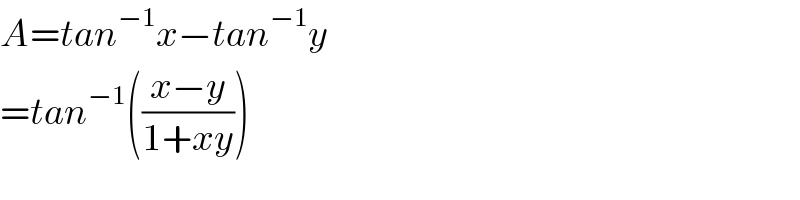
$${A}={tan}^{−\mathrm{1}} {x}−{tan}^{−\mathrm{1}} {y} \\ $$$$={tan}^{−\mathrm{1}} \left(\frac{{x}−{y}}{\mathrm{1}+{xy}}\right) \\ $$
