Question and Answers Forum

All Questions      Topic List

Limits Questions

Previous in All Question      Next in All Question      

Previous in Limits      Next in Limits      

Question Number 34092 by rahul 19 last updated on 30/Apr/18

l_n i_ m_∞ ((((n!))/((nm)^n )))^(1/n)

$$\underset{{n}} {{l}}\underset{} {{i}}\underset{\infty} {{m}}\:\left(\frac{\left({n}!\right)}{\left({nm}\right)^{{n}} }\right)^{\frac{\mathrm{1}}{{n}}} \\ $$

Commented by MJS last updated on 30/Apr/18

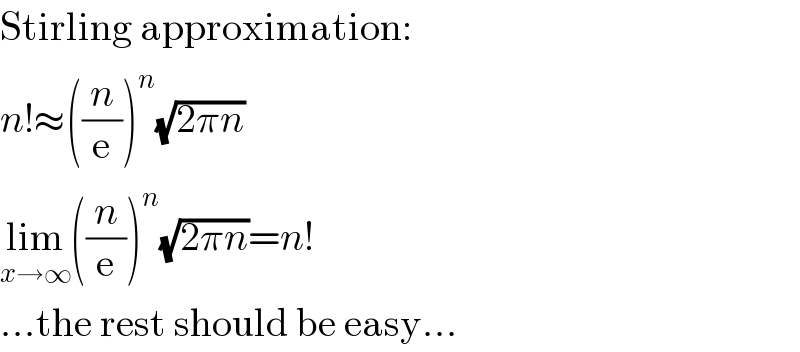
Stirling approximation: n!≈((n/e))^n (√(2πn)) lim_(x→∞) ((n/e))^n (√(2πn))=n! ...the rest should be easy...

$$\mathrm{Stirling}\:\mathrm{approximation}: \\ $$$${n}!\approx\left(\frac{{n}}{\mathrm{e}}\right)^{{n}} \sqrt{\mathrm{2}\pi{n}} \\ $$$$\underset{{x}\rightarrow\infty} {\mathrm{lim}}\left(\frac{{n}}{\mathrm{e}}\right)^{{n}} \sqrt{\mathrm{2}\pi{n}}={n}! \\ $$$$...\mathrm{the}\:\mathrm{rest}\:\mathrm{should}\:\mathrm{be}\:\mathrm{easy}... \\ $$

Commented by rahul 19 last updated on 30/Apr/18

pls do this by ′stirling approximation′ and also do explain what′s that .

$${pls}\:{do}\:\:{this}\:{by}\:'{stirling}\:{approximation}' \\ $$$${and}\:{also}\:{do}\:{explain}\:{what}'{s}\:{that}\:. \\ $$

Commented by tanmay.chaudhury50@gmail.com last updated on 01/May/18

there is a method to solve this type of problem let me share theorem first along with some problem then i shall solve it

$${there}\:{is}\:{a}\:{method}\:{to}\:{solve}\:{this}\:{type}\:{of}\:{problem} \\ $$$${let}\:{me}\:{share}\:{theorem}\:{first}\:{along}\:{with}\:{some} \\ $$$${problem}\:{then}\:{i}\:{shall}\:{solve}\:{it} \\ $$

Commented by tanmay.chaudhury50@gmail.com last updated on 01/May/18

Commented by tanmay.chaudhury50@gmail.com last updated on 01/May/18

x_n =((n!)/((nm)^n )) so x_(n+1) =(((n+1)!)/({(n+1)m}^(n+1) )) (x_n /(x_(n+1) ))=((n!)/((n+1)!)).(({(n+1)m}^(n+1) )/((nm)^n )) =(1/(n+1)).(({{(n+1)m}^n )/((nm)^(n ) )).{(n+1)m} =m.(((n+1)^n )/n^n ) (x_(n+1) /x_n )=(1/m).(n^n /((n+1)^n )) =(1/m).(1/((1+((1 )/n))^n )) lim n→∞(x_(n+1) /x_n ) =(1/(e.m)) that is required ans of the question

$${x}_{{n}} =\frac{{n}!}{\left({nm}\right)^{{n}} }\:\:\:\:{so}\:{x}_{{n}+\mathrm{1}} =\frac{\left({n}+\mathrm{1}\right)!}{\left\{\left({n}+\mathrm{1}\right){m}\right\}^{{n}+\mathrm{1}} } \\ $$$$\frac{{x}_{{n}} }{{x}_{{n}+\mathrm{1}} \:}=\frac{{n}!}{\left({n}+\mathrm{1}\right)!}.\frac{\left\{\left({n}+\mathrm{1}\right){m}\right\}^{{n}+\mathrm{1}} }{\left({nm}\right)^{{n}} } \\ $$$$=\frac{\mathrm{1}}{{n}+\mathrm{1}}.\frac{\left\{\left\{\left({n}+\mathrm{1}\right){m}\right\}^{{n}} \:\right.}{\left({nm}\right)^{{n}\:} }.\left\{\left({n}+\mathrm{1}\right){m}\right\} \\ $$$$={m}.\frac{\left({n}+\mathrm{1}\right)^{{n}} }{{n}^{{n}} } \\ $$$$\frac{{x}_{{n}+\mathrm{1}} }{{x}_{{n}} }=\frac{\mathrm{1}}{{m}}.\frac{{n}^{{n}} }{\left({n}+\mathrm{1}\right)^{{n}} } \\ $$$$=\frac{\mathrm{1}}{{m}}.\frac{\mathrm{1}}{\left(\mathrm{1}+\frac{\mathrm{1}\:}{{n}}\right)^{{n}} } \\ $$$${lim}\:{n}\rightarrow\infty\frac{{x}_{{n}+\mathrm{1}} }{{x}_{{n}} } \\ $$$$=\frac{\mathrm{1}}{{e}.{m}} \\ $$$${that}\:{is}\:{required}\:{ans}\:{of}\:{the}\:{question} \\ $$$$ \\ $$

Commented by rahul 19 last updated on 01/May/18

Thank you sir. well, i think stirling aprroximation is better (as it is short) . Anyways, it′s also a nice method.

$$\mathscr{T}{hank}\:{you}\:{sir}. \\ $$$${well},\:{i}\:{think}\:{stirling}\:{aprroximation} \\ $$$${is}\:{better}\:\left({as}\:{it}\:{is}\:{short}\right)\:.\:{Anyways}, \\ $$$${it}'{s}\:{also}\:{a}\:{nice}\:{method}. \\ $$

Commented by rahul 19 last updated on 01/May/18

pls do post the solutions of the given 5 Ques. also by this theorem.

Commented by math khazana by abdo last updated on 02/May/18

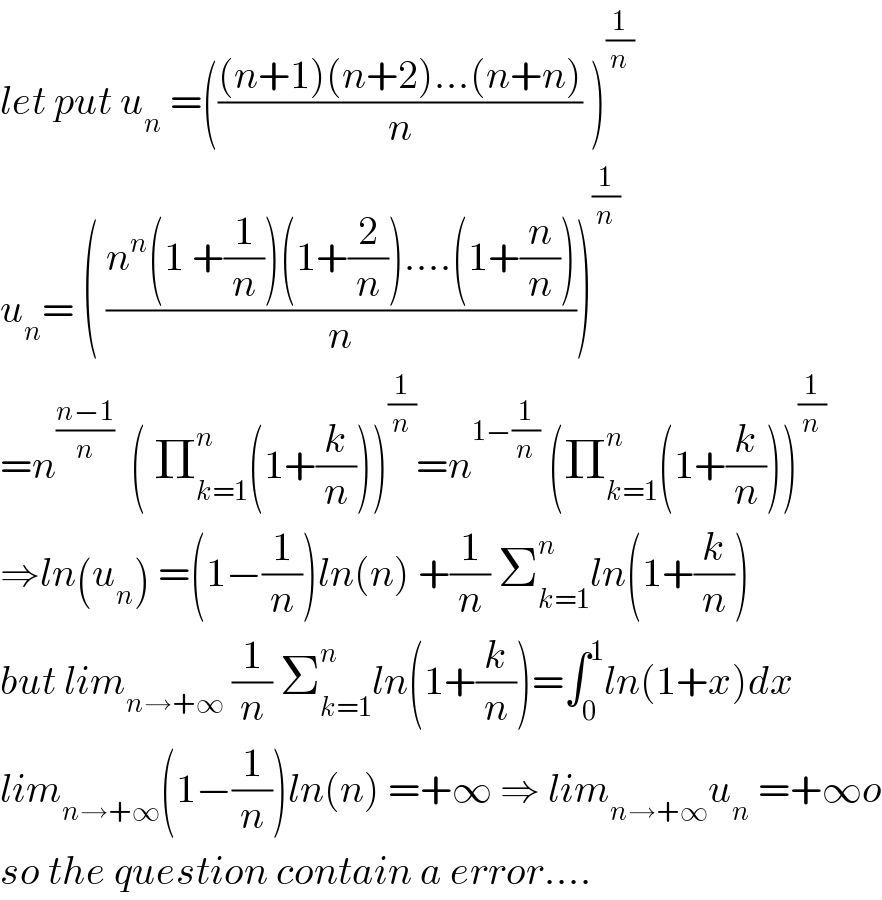
let put u_n =((((n+1)(n+2)...(n+n))/n) )^(1/n) u_n = ( ((n^n (1 +(1/n))(1+(2/n))....(1+(n/n)))/n))^(1/n) =n^((n−1)/n) ( Π_(k=1) ^n (1+(k/n)))^(1/n) =n^(1−(1/n)) (Π_(k=1) ^n (1+(k/n)))^(1/n) ⇒ln(u_n ) =(1−(1/n))ln(n) +(1/n) Σ_(k=1) ^n ln(1+(k/n)) but lim_(n→+∞) (1/n) Σ_(k=1) ^n ln(1+(k/n))=∫_0 ^1 ln(1+x)dx lim_(n→+∞) (1−(1/n))ln(n) =+∞ ⇒ lim_(n→+∞) u_n =+∞o so the question contain a error....

$${let}\:{put}\:{u}_{{n}} \:=\left(\frac{\left({n}+\mathrm{1}\right)\left({n}+\mathrm{2}\right)...\left({n}+{n}\right)}{{n}}\:\right)^{\frac{\mathrm{1}}{{n}}} \\ $$$${u}_{{n}} =\:\left(\:\frac{{n}^{{n}} \left(\mathrm{1}\:+\frac{\mathrm{1}}{{n}}\right)\left(\mathrm{1}+\frac{\mathrm{2}}{{n}}\right)....\left(\mathrm{1}+\frac{{n}}{{n}}\right)}{{n}}\right)^{\frac{\mathrm{1}}{{n}}} \\ $$$$={n}^{\frac{{n}−\mathrm{1}}{{n}}} \:\:\left(\:\prod_{{k}=\mathrm{1}} ^{{n}} \left(\mathrm{1}+\frac{{k}}{{n}}\right)\right)^{\frac{\mathrm{1}}{{n}}} ={n}^{\mathrm{1}−\frac{\mathrm{1}}{{n}}} \:\left(\prod_{{k}=\mathrm{1}} ^{{n}} \left(\mathrm{1}+\frac{{k}}{{n}}\right)\right)^{\frac{\mathrm{1}}{{n}}} \\ $$$$\Rightarrow{ln}\left({u}_{{n}} \right)\:=\left(\mathrm{1}−\frac{\mathrm{1}}{{n}}\right){ln}\left({n}\right)\:+\frac{\mathrm{1}}{{n}}\:\sum_{{k}=\mathrm{1}} ^{{n}} {ln}\left(\mathrm{1}+\frac{{k}}{{n}}\right) \\ $$$${but}\:{lim}_{{n}\rightarrow+\infty} \:\frac{\mathrm{1}}{{n}}\:\sum_{{k}=\mathrm{1}} ^{{n}} {ln}\left(\mathrm{1}+\frac{{k}}{{n}}\right)=\int_{\mathrm{0}} ^{\mathrm{1}} {ln}\left(\mathrm{1}+{x}\right){dx} \\ $$$${lim}_{{n}\rightarrow+\infty} \left(\mathrm{1}−\frac{\mathrm{1}}{{n}}\right){ln}\left({n}\right)\:=+\infty\:\Rightarrow\:{lim}_{{n}\rightarrow+\infty} {u}_{{n}} \:=+\infty{o} \\ $$$${so}\:{the}\:{question}\:{contain}\:{a}\:{error}.... \\ $$

Commented by tanmay.chaudhury50@gmail.com last updated on 03/May/18

Answered by MJS last updated on 30/Apr/18

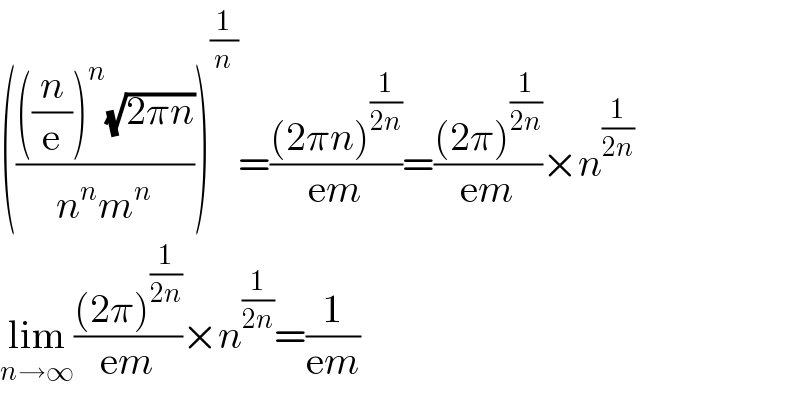
(((((n/e))^n (√(2πn)))/(n^n m^n )))^(1/n) =(((2πn)^(1/(2n)) )/(em))=(((2π)^(1/(2n)) )/(em))×n^(1/(2n)) lim_(n→∞) (((2π)^(1/(2n)) )/(em))×n^(1/(2n)) =(1/(em))

$$\left(\frac{\left(\frac{{n}}{\mathrm{e}}\right)^{{n}} \sqrt{\mathrm{2}\pi{n}}}{{n}^{{n}} {m}^{{n}} }\right)^{\frac{\mathrm{1}}{{n}}} =\frac{\left(\mathrm{2}\pi{n}\right)^{\frac{\mathrm{1}}{\mathrm{2}{n}}} }{\mathrm{e}{m}}=\frac{\left(\mathrm{2}\pi\right)^{\frac{\mathrm{1}}{\mathrm{2}{n}}} }{\mathrm{e}{m}}×{n}^{\frac{\mathrm{1}}{\mathrm{2}{n}}} \\ $$$$\underset{{n}\rightarrow\infty} {\mathrm{lim}}\frac{\left(\mathrm{2}\pi\right)^{\frac{\mathrm{1}}{\mathrm{2}{n}}} }{\mathrm{e}{m}}×{n}^{\frac{\mathrm{1}}{\mathrm{2}{n}}} =\frac{\mathrm{1}}{\mathrm{e}{m}} \\ $$

Commented by rahul 19 last updated on 30/Apr/18

my doubt is : how l_n i_ m_∞ n^(1/(2n)) = 1 ?

$${my}\:{doubt}\:{is}\::\: \\ $$$${how}\:\underset{{n}} {\mathrm{l}}\underset{} {\mathrm{i}}\underset{\infty} {\mathrm{m}}\:{n}^{\frac{\mathrm{1}}{\mathrm{2}{n}}} \:=\:\mathrm{1}\:? \\ $$

Commented by MJS last updated on 30/Apr/18

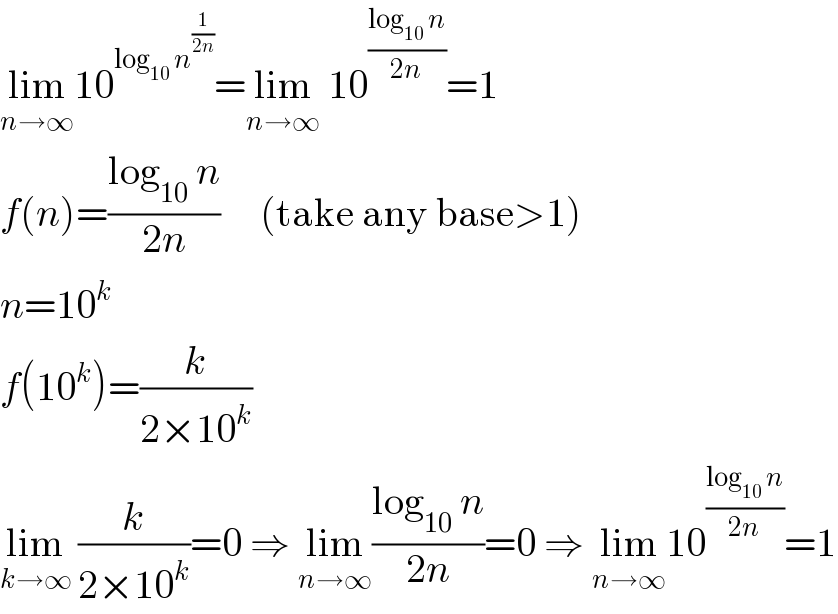
lim_(n→∞) 10^(log_(10) n^(1/(2n)) ) =lim_(n→∞) 10^((log_(10) n)/(2n)) =1 f(n)=((log_(10) n)/(2n)) (take any base>1) n=10^k f(10^k )=(k/(2×10^k )) lim_(k→∞) (k/(2×10^k ))=0 ⇒ lim_(n→∞) ((log_(10) n)/(2n))=0 ⇒ lim_(n→∞) 10^((log_(10) n)/(2n)) =1

$$\underset{{n}\rightarrow\infty} {\mathrm{lim}10}^{\mathrm{log}_{\mathrm{10}} \:{n}^{\frac{\mathrm{1}}{\mathrm{2}{n}}} } =\underset{{n}\rightarrow\infty} {\mathrm{lim}}\:\mathrm{10}^{\frac{\mathrm{log}_{\mathrm{10}} \:{n}}{\mathrm{2}{n}}} =\mathrm{1} \\ $$$${f}\left({n}\right)=\frac{\mathrm{log}_{\mathrm{10}} \:{n}}{\mathrm{2}{n}}\:\:\:\:\:\left(\mathrm{take}\:\mathrm{any}\:\mathrm{base}>\mathrm{1}\right) \\ $$$${n}=\mathrm{10}^{{k}} \\ $$$${f}\left(\mathrm{10}^{{k}} \right)=\frac{{k}}{\mathrm{2}×\mathrm{10}^{{k}} } \\ $$$$\underset{{k}\rightarrow\infty} {\mathrm{lim}}\:\frac{{k}}{\mathrm{2}×\mathrm{10}^{{k}} }=\mathrm{0}\:\Rightarrow\:\underset{{n}\rightarrow\infty} {\mathrm{lim}}\frac{\mathrm{log}_{\mathrm{10}} \:{n}}{\mathrm{2}{n}}=\mathrm{0}\:\Rightarrow\:\underset{{n}\rightarrow\infty} {\mathrm{lim}10}^{\frac{\mathrm{log}_{\mathrm{10}} \:{n}}{\mathrm{2}{n}}} =\mathrm{1} \\ $$

Commented by rahul 19 last updated on 30/Apr/18

Thank you sir !

$$\mathscr{T}\mathrm{hank}\:\mathrm{you}\:\mathrm{sir}\:! \\ $$

Commented by prof Abdo imad last updated on 30/Apr/18

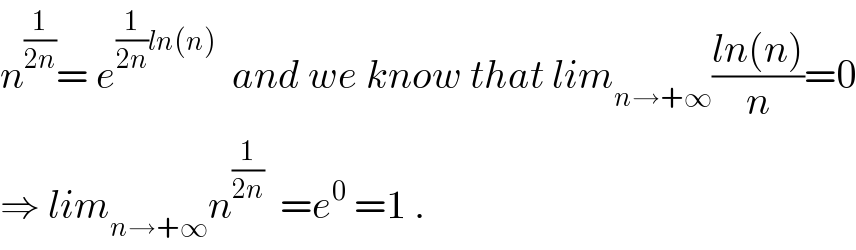
n^(1/(2n)) = e^((1/(2n))ln(n)) and we know that lim_(n→+∞) ((ln(n))/n)=0 ⇒ lim_(n→+∞) n^(1/(2n)) =e^0 =1 .

$${n}^{\frac{\mathrm{1}}{\mathrm{2}{n}}} =\:{e}^{\frac{\mathrm{1}}{\mathrm{2}{n}}{ln}\left({n}\right)} \:\:{and}\:{we}\:{know}\:{that}\:{lim}_{{n}\rightarrow+\infty} \frac{{ln}\left({n}\right)}{{n}}=\mathrm{0} \\ $$$$\Rightarrow\:{lim}_{{n}\rightarrow+\infty} {n}^{\frac{\mathrm{1}}{\mathrm{2}{n}}} \:\:={e}^{\mathrm{0}} \:=\mathrm{1}\:. \\ $$

Commented by rahul 19 last updated on 01/May/18

Thank you sir!

$$\mathscr{T}\mathrm{hank}\:\mathrm{you}\:\mathrm{sir}! \\ $$

Answered by tanmay.chaudhury50@gmail.com last updated on 01/May/18

Terms of Service

Privacy Policy

Contact: info@tinkutara.com