Question and Answers Forum

All Questions      Topic List

None Questions

Previous in All Question      Next in All Question      

Previous in None      Next in None      

Question Number 110539 by Her_Majesty last updated on 29/Aug/20

it seems too hard for many here to post their questions as questions, answers as answers and comments as comments... hereby I introduce the next step: I′ll post answers and the task is, find questions to these answers (1) ζ(3)+πln2 (2) πH_0 (7) (3) true ∀x∈C\Q (4) _2 F_1 ((3/2), (1/5), (2/3), sin^(−1) ((x+1)/(x−1)))

$${it}\:{seems}\:{too}\:{hard}\:{for}\:{many}\:{here}\:{to}\:{post} \\ $$$${their}\:{questions}\:{as}\:{questions},\:{answers}\:{as} \\ $$$${answers}\:{and}\:{comments}\:{as}\:{comments}... \\ $$$${hereby}\:{I}\:{introduce}\:{the}\:{next}\:{step}:\:{I}'{ll}\:{post} \\ $$$${answers}\:{and}\:{the}\:{task}\:{is},\:{find}\:{questions} \\ $$$${to}\:{these}\:{answers} \\ $$$$\left(\mathrm{1}\right)\:\zeta\left(\mathrm{3}\right)+\pi{ln}\mathrm{2} \\ $$$$\left(\mathrm{2}\right)\:\pi{H}_{\mathrm{0}} \left(\mathrm{7}\right) \\ $$$$\left(\mathrm{3}\right)\:{true}\:\forall{x}\in\mathbb{C}\backslash\mathbb{Q} \\ $$$$\left(\mathrm{4}\right)\:_{\mathrm{2}} {F}_{\mathrm{1}} \left(\frac{\mathrm{3}}{\mathrm{2}},\:\frac{\mathrm{1}}{\mathrm{5}},\:\frac{\mathrm{2}}{\mathrm{3}},\:{sin}^{−\mathrm{1}} \frac{{x}+\mathrm{1}}{{x}−\mathrm{1}}\right) \\ $$

Commented by Eric002 last updated on 29/Aug/20

i think only prof. math mind and and prof.∗M∗th+et+s have knowledge of (4) hypergeometric function and they haven′t been around for a long time

$${i}\:{think}\:{only}\:{prof}.\:{math}\:{mind}\:{and}\: \\ $$$${and}\:{prof}.\ast{M}\ast{th}+{et}+{s}\:{have}\:{knowledge} \\ $$$${of}\:\left(\mathrm{4}\right)\:{hypergeometric}\:{function}\:{and}\:{they} \\ $$$${haven}'{t}\:{been}\:{around}\:{for}\:{a}\:{long}\:{time} \\ $$$$ \\ $$

Commented by Dwaipayan Shikari last updated on 29/Aug/20

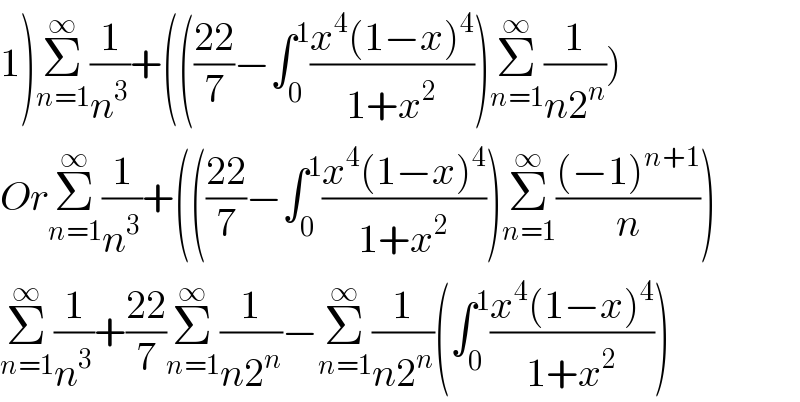
1)Σ_(n=1) ^∞ (1/n^3 )+((((22)/7)−∫_0 ^1 ((x^4 (1−x)^4 )/(1+x^2 )))Σ_(n=1) ^∞ (1/(n2^n ))) OrΣ_(n=1) ^∞ (1/n^3 )+((((22)/7)−∫_0 ^1 ((x^4 (1−x)^4 )/(1+x^2 )))Σ_(n=1) ^∞ (((−1)^(n+1) )/n)) Σ_(n=1) ^∞ (1/n^3 )+((22)/7)Σ_(n=1) ^∞ (1/(n2^n ))−Σ_(n=1) ^∞ (1/(n2^n ))(∫_0 ^1 ((x^4 (1−x)^4 )/(1+x^2 )))

$$\left.\mathrm{1}\right)\underset{{n}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{\mathrm{1}}{{n}^{\mathrm{3}} }+\left(\left(\frac{\mathrm{22}}{\mathrm{7}}−\int_{\mathrm{0}} ^{\mathrm{1}} \frac{{x}^{\mathrm{4}} \left(\mathrm{1}−{x}\right)^{\mathrm{4}} }{\mathrm{1}+{x}^{\mathrm{2}} }\right)\underset{{n}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{\mathrm{1}}{{n}\mathrm{2}^{{n}} }\right) \\ $$$${Or}\underset{{n}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{\mathrm{1}}{{n}^{\mathrm{3}} }+\left(\left(\frac{\mathrm{22}}{\mathrm{7}}−\int_{\mathrm{0}} ^{\mathrm{1}} \frac{{x}^{\mathrm{4}} \left(\mathrm{1}−{x}\right)^{\mathrm{4}} }{\mathrm{1}+{x}^{\mathrm{2}} }\right)\underset{{n}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{\left(−\mathrm{1}\right)^{{n}+\mathrm{1}} }{{n}}\right) \\ $$$$\underset{{n}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{\mathrm{1}}{{n}^{\mathrm{3}} }+\frac{\mathrm{22}}{\mathrm{7}}\underset{{n}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{\mathrm{1}}{{n}\mathrm{2}^{{n}} }−\underset{{n}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{\mathrm{1}}{{n}\mathrm{2}^{{n}} }\left(\int_{\mathrm{0}} ^{\mathrm{1}} \frac{{x}^{\mathrm{4}} \left(\mathrm{1}−{x}\right)^{\mathrm{4}} }{\mathrm{1}+{x}^{\mathrm{2}} }\right) \\ $$

Commented by Dwaipayan Shikari last updated on 29/Aug/20

Infinitely many questions ����

Commented by Her_Majesty last updated on 29/Aug/20

��

Terms of Service

Privacy Policy

Contact: info@tinkutara.com