Question and Answers Forum

All Questions      Topic List

Differentiation Questions

Previous in All Question      Next in All Question      

Previous in Differentiation      Next in Differentiation      

Question Number 196401 by RoseAli last updated on 24/Aug/23

if y=sin x find (d^2 /dy^2 )cos^7 x

$$\mathrm{if}\:{y}=\mathrm{sin}\:{x}\: \\ $$$$\mathrm{find}\:\frac{\boldsymbol{{d}}^{\mathrm{2}} }{\boldsymbol{{d}}{y}^{\mathrm{2}} }\mathrm{co}\boldsymbol{{s}}^{\mathrm{7}} \boldsymbol{{x}} \\ $$

Answered by qaz last updated on 24/Aug/23

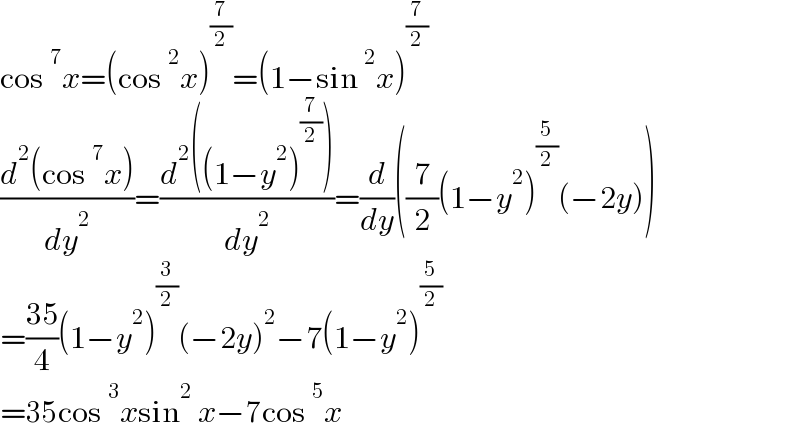
cos^7 x=(cos^2 x)^(7/2) =(1−sin^2 x)^(7/2) ((d^2 (cos^7 x))/dy^2 )=((d^2 ((1−y^2 )^(7/2) ))/dy^2 )=(d/dy)((7/2)(1−y^2 )^(5/2) (−2y)) =((35)/4)(1−y^2 )^(3/2) (−2y)^2 −7(1−y^2 )^(5/2) =35cos^3 xsin^2 x−7cos^5 x

$$\mathrm{cos}\:^{\mathrm{7}} {x}=\left(\mathrm{cos}\:^{\mathrm{2}} {x}\right)^{\frac{\mathrm{7}}{\mathrm{2}}} =\left(\mathrm{1}−\mathrm{sin}\:^{\mathrm{2}} {x}\right)^{\frac{\mathrm{7}}{\mathrm{2}}} \\ $$$$\frac{{d}^{\mathrm{2}} \left(\mathrm{cos}\:^{\mathrm{7}} {x}\right)}{{dy}^{\mathrm{2}} }=\frac{{d}^{\mathrm{2}} \left(\left(\mathrm{1}−{y}^{\mathrm{2}} \right)^{\frac{\mathrm{7}}{\mathrm{2}}} \right)}{{dy}^{\mathrm{2}} }=\frac{{d}}{{dy}}\left(\frac{\mathrm{7}}{\mathrm{2}}\left(\mathrm{1}−{y}^{\mathrm{2}} \right)^{\frac{\mathrm{5}}{\mathrm{2}}} \left(−\mathrm{2}{y}\right)\right) \\ $$$$=\frac{\mathrm{35}}{\mathrm{4}}\left(\mathrm{1}−{y}^{\mathrm{2}} \right)^{\frac{\mathrm{3}}{\mathrm{2}}} \left(−\mathrm{2}{y}\right)^{\mathrm{2}} −\mathrm{7}\left(\mathrm{1}−{y}^{\mathrm{2}} \right)^{\frac{\mathrm{5}}{\mathrm{2}}} \\ $$$$=\mathrm{35cos}\:^{\mathrm{3}} {x}\mathrm{sin}^{\mathrm{2}} \:{x}−\mathrm{7cos}\:^{\mathrm{5}} {x} \\ $$

Answered by mr W last updated on 24/Aug/23

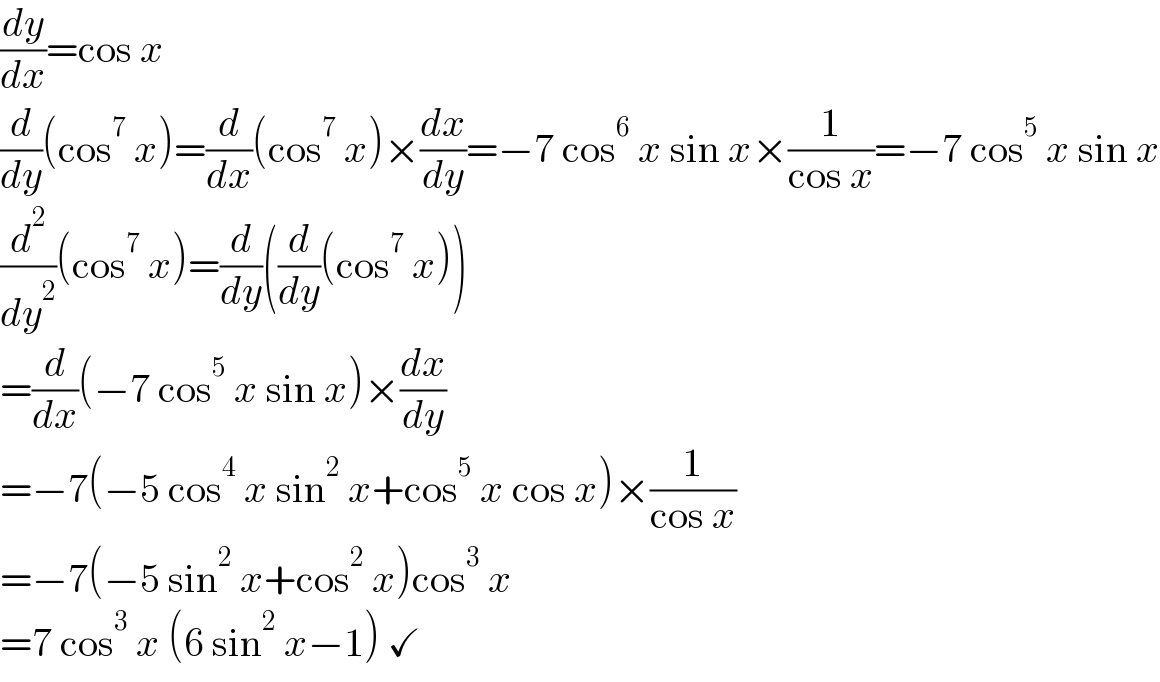
(dy/dx)=cos x (d/dy)(cos^7 x)=(d/dx)(cos^7 x)×(dx/dy)=−7 cos^6 x sin x×(1/(cos x))=−7 cos^5 x sin x (d^2 /dy^2 )(cos^7 x)=(d/dy)((d/dy)(cos^7 x)) =(d/dx)(−7 cos^5 x sin x)×(dx/dy) =−7(−5 cos^4 x sin^2 x+cos^5 x cos x)×(1/(cos x)) =−7(−5 sin^2 x+cos^2 x)cos^3 x =7 cos^3 x (6 sin^2 x−1) ✓

$$\frac{{dy}}{{dx}}=\mathrm{cos}\:{x} \\ $$$$\frac{{d}}{{dy}}\left(\mathrm{cos}^{\mathrm{7}} \:{x}\right)=\frac{{d}}{{dx}}\left(\mathrm{cos}^{\mathrm{7}} \:{x}\right)×\frac{{dx}}{{dy}}=−\mathrm{7}\:\mathrm{cos}^{\mathrm{6}} \:{x}\:\mathrm{sin}\:{x}×\frac{\mathrm{1}}{\mathrm{cos}\:{x}}=−\mathrm{7}\:\mathrm{cos}^{\mathrm{5}} \:{x}\:\mathrm{sin}\:{x} \\ $$$$\frac{{d}^{\mathrm{2}} }{{dy}^{\mathrm{2}} }\left(\mathrm{cos}^{\mathrm{7}} \:{x}\right)=\frac{{d}}{{dy}}\left(\frac{{d}}{{dy}}\left(\mathrm{cos}^{\mathrm{7}} \:{x}\right)\right) \\ $$$$=\frac{{d}}{{dx}}\left(−\mathrm{7}\:\mathrm{cos}^{\mathrm{5}} \:{x}\:\mathrm{sin}\:{x}\right)×\frac{{dx}}{{dy}} \\ $$$$=−\mathrm{7}\left(−\mathrm{5}\:\mathrm{cos}^{\mathrm{4}} \:{x}\:\mathrm{sin}^{\mathrm{2}} \:{x}+\mathrm{cos}^{\mathrm{5}} \:{x}\:\mathrm{cos}\:{x}\right)×\frac{\mathrm{1}}{\mathrm{cos}\:{x}} \\ $$$$=−\mathrm{7}\left(−\mathrm{5}\:\mathrm{sin}^{\mathrm{2}} \:{x}+\mathrm{cos}^{\mathrm{2}} \:{x}\right)\mathrm{cos}^{\mathrm{3}} \:{x} \\ $$$$=\mathrm{7}\:\mathrm{cos}^{\mathrm{3}} \:{x}\:\left(\mathrm{6}\:\mathrm{sin}^{\mathrm{2}} \:{x}−\mathrm{1}\right)\:\checkmark \\ $$

Answered by cortano12 last updated on 24/Aug/23

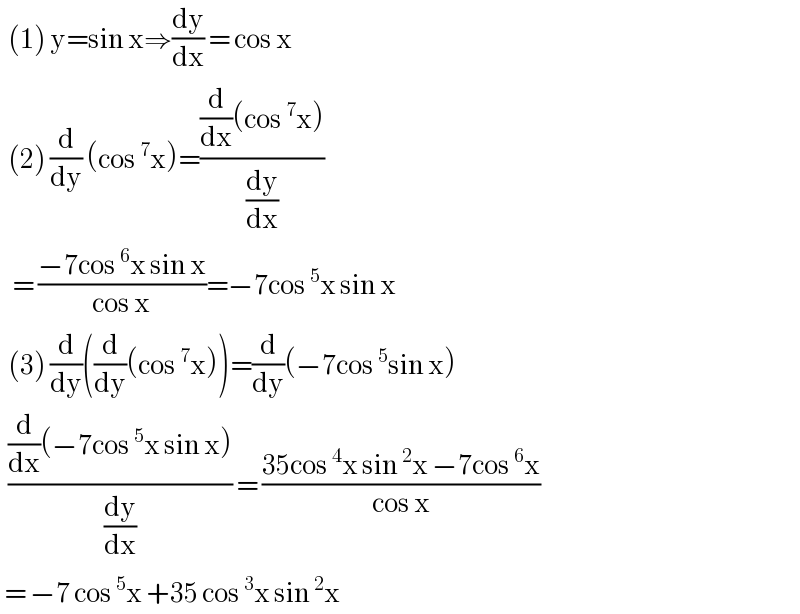
(1) y=sin x⇒(dy/dx) = cos x (2) (d/dy) (cos^7 x)=(((d/dx)(cos^7 x))/(dy/dx)) = ((−7cos^6 x sin x)/(cos x))=−7cos^5 x sin x (3) (d/dy)((d/dy)(cos^7 x))=(d/dy)(−7cos^5 sin x) (((d/dx)(−7cos^5 x sin x))/(dy/dx)) = ((35cos^4 x sin^2 x −7cos^6 x)/(cos x)) = −7 cos^5 x +35 cos^3 x sin^2 x

$$\:\:\left(\mathrm{1}\right)\:\mathrm{y}=\mathrm{sin}\:\mathrm{x}\Rightarrow\frac{\mathrm{dy}}{\mathrm{dx}}\:=\:\mathrm{cos}\:\mathrm{x} \\ $$$$\:\:\left(\mathrm{2}\right)\:\frac{\mathrm{d}}{\mathrm{dy}}\:\left(\mathrm{cos}\:^{\mathrm{7}} \mathrm{x}\right)=\frac{\frac{\mathrm{d}}{\mathrm{dx}}\left(\mathrm{cos}\:^{\mathrm{7}} \mathrm{x}\right)}{\frac{\mathrm{dy}}{\mathrm{dx}}} \\ $$$$\:\:\:=\:\frac{−\mathrm{7cos}\:^{\mathrm{6}} \mathrm{x}\:\mathrm{sin}\:\mathrm{x}}{\mathrm{cos}\:\mathrm{x}}=−\mathrm{7cos}\:^{\mathrm{5}} \mathrm{x}\:\mathrm{sin}\:\mathrm{x} \\ $$$$\:\:\left(\mathrm{3}\right)\:\frac{\mathrm{d}}{\mathrm{dy}}\left(\frac{\mathrm{d}}{\mathrm{dy}}\left(\mathrm{cos}\:^{\mathrm{7}} \mathrm{x}\right)\right)=\frac{\mathrm{d}}{\mathrm{dy}}\left(−\mathrm{7cos}\:^{\mathrm{5}} \mathrm{sin}\:\mathrm{x}\right) \\ $$$$\:\:\frac{\frac{\mathrm{d}}{\mathrm{dx}}\left(−\mathrm{7cos}\:^{\mathrm{5}} \mathrm{x}\:\mathrm{sin}\:\mathrm{x}\right)}{\frac{\mathrm{dy}}{\mathrm{dx}}}\:=\:\frac{\mathrm{35cos}\:^{\mathrm{4}} \mathrm{x}\:\mathrm{sin}\:^{\mathrm{2}} \mathrm{x}\:−\mathrm{7cos}\:^{\mathrm{6}} \mathrm{x}}{\mathrm{cos}\:\mathrm{x}} \\ $$$$\:=\:−\mathrm{7}\:\mathrm{cos}\:^{\mathrm{5}} \mathrm{x}\:+\mathrm{35}\:\mathrm{cos}\:^{\mathrm{3}} \mathrm{x}\:\mathrm{sin}\:^{\mathrm{2}} \mathrm{x}\: \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com