
Question Number 159330 by bounhome last updated on 15/Nov/21
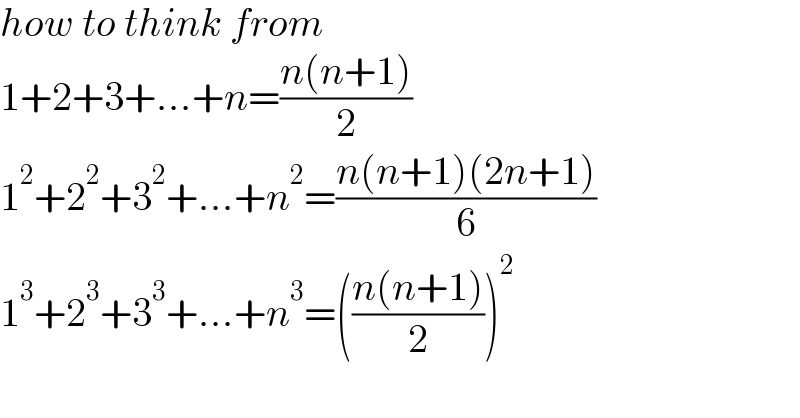
$${how}\:{to}\:{think}\:{from}\: \\ $$$$\mathrm{1}+\mathrm{2}+\mathrm{3}+...+{n}=\frac{{n}\left({n}+\mathrm{1}\right)}{\mathrm{2}} \\ $$$$\mathrm{1}^{\mathrm{2}} +\mathrm{2}^{\mathrm{2}} +\mathrm{3}^{\mathrm{2}} +...+{n}^{\mathrm{2}} =\frac{{n}\left({n}+\mathrm{1}\right)\left(\mathrm{2}{n}+\mathrm{1}\right)}{\mathrm{6}} \\ $$$$\mathrm{1}^{\mathrm{3}} +\mathrm{2}^{\mathrm{3}} +\mathrm{3}^{\mathrm{3}} +...+{n}^{\mathrm{3}} =\left(\frac{{n}\left({n}+\mathrm{1}\right)}{\mathrm{2}}\right)^{\mathrm{2}} \\ $$
Answered by Ar Brandon last updated on 15/Nov/21

$${S}=\mathrm{1}+\mathrm{2}+\mathrm{3}+...\left(\mathrm{n}−\mathrm{2}\right)+\left(\mathrm{n}−\mathrm{1}\right)+\mathrm{n} \\ $$$${S}=\mathrm{n}+\left(\mathrm{n}−\mathrm{1}\right)+\left(\mathrm{n}−\mathrm{2}\right)...+\mathrm{3}+\mathrm{2}+\mathrm{1} \\ $$$$\mathrm{2}{S}=\left(\mathrm{n}+\mathrm{1}\right)+\left(\mathrm{n}+\mathrm{1}\right)+\left(\mathrm{n}+\mathrm{1}\right)+...+\left(\mathrm{n}+\mathrm{1}\right)+\left(\mathrm{n}+\mathrm{1}\right)+\left(\mathrm{n}+\mathrm{1}\right) \\ $$$$\:\:\:\:\:\:=\mathrm{n}\left(\mathrm{n}+\mathrm{1}\right)\Rightarrow{S}=\frac{\mathrm{n}\left(\mathrm{n}+\mathrm{1}\right)}{\mathrm{2}} \\ $$
