Question and Answers Forum

All Questions      Topic List

Relation and Functions Questions

Previous in All Question      Next in All Question      

Previous in Relation and Functions      Next in Relation and Functions      

Question Number 144109 by henderson last updated on 21/Jun/21

hi, everybody ! 1. calculate : I =∫_(𝛑/6) ^( (𝛑/3)) ln(tan x)dx. 2. calculate : lim_(x β†’ e) ((x(√(1βˆ’ln x)))/(xβˆ’e)) .

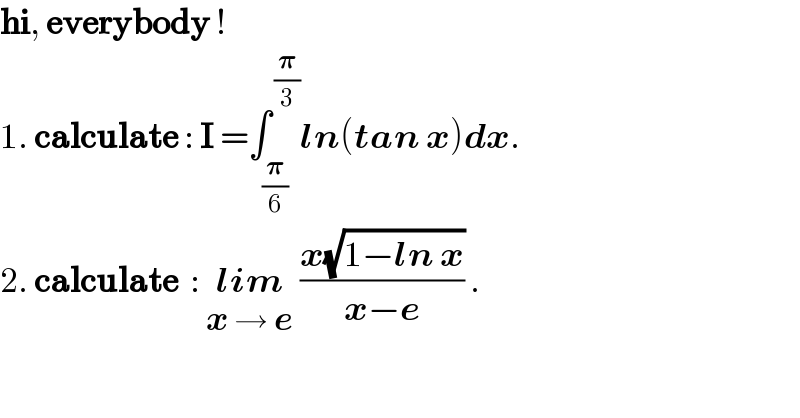
$$\boldsymbol{\mathrm{hi}},\:\boldsymbol{\mathrm{everybody}}\:! \\ $$$$\mathrm{1}.\:\boldsymbol{\mathrm{calculate}}\::\:\boldsymbol{\mathrm{I}}\:=\int_{\frac{\boldsymbol{\pi}}{\mathrm{6}}} ^{\:\frac{\boldsymbol{\pi}}{\mathrm{3}}} \boldsymbol{{ln}}\left(\boldsymbol{{tan}}\:\boldsymbol{{x}}\right)\boldsymbol{{dx}}. \\ $$$$\mathrm{2}.\:\boldsymbol{\mathrm{calculate}}\:\::\:\underset{\boldsymbol{{x}}\:\rightarrow\:\boldsymbol{{e}}} {\boldsymbol{{lim}}}\:\frac{\boldsymbol{{x}}\sqrt{\mathrm{1}βˆ’\boldsymbol{{ln}}\:\boldsymbol{{x}}}}{\boldsymbol{{x}}βˆ’\boldsymbol{{e}}}\:. \\ $$

Commented by bobhans last updated on 22/Jun/21

(2) lim_(xβ†’e) ((e (√(1βˆ’ln x)))/(xβˆ’e)) = lim_(xβ†’e) ((e(((βˆ’(1/x))/(2(√(1βˆ’ln x))))))/1) =βˆ’ ∞

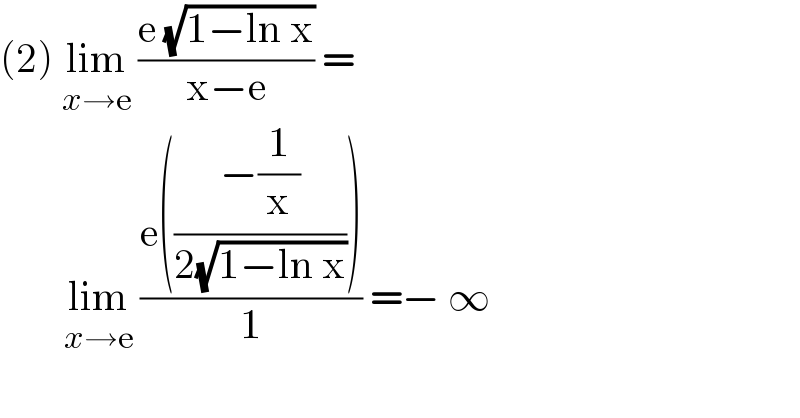
$$\left(\mathrm{2}\right)\:\underset{{x}\rightarrow\mathrm{e}} {\mathrm{lim}}\:\frac{\mathrm{e}\:\sqrt{\mathrm{1}βˆ’\mathrm{ln}\:\mathrm{x}}}{\mathrm{x}βˆ’\mathrm{e}}\:= \\ $$$$\:\:\:\:\:\:\:\:\underset{{x}\rightarrow\mathrm{e}} {\mathrm{lim}}\:\frac{\mathrm{e}\left(\frac{βˆ’\frac{\mathrm{1}}{\mathrm{x}}}{\mathrm{2}\sqrt{\mathrm{1}βˆ’\mathrm{ln}\:\mathrm{x}}}\right)}{\mathrm{1}}\:=βˆ’\:\infty \\ $$$$\:\:\:\:\:\:\:\: \\ $$

Answered by Olaf_Thorendsen last updated on 21/Jun/21

I = ∫_(Ο€/6) ^(Ο€/3) (ln(tanx) dx Let u = (Ο€/2)βˆ’x I = ∫_(Ο€/6) ^(Ο€/3) ln(cotu) du = βˆ’βˆ«_(Ο€/6) ^(Ο€/3) ln(tanx) dx I = βˆ’I β‡’ I = 0

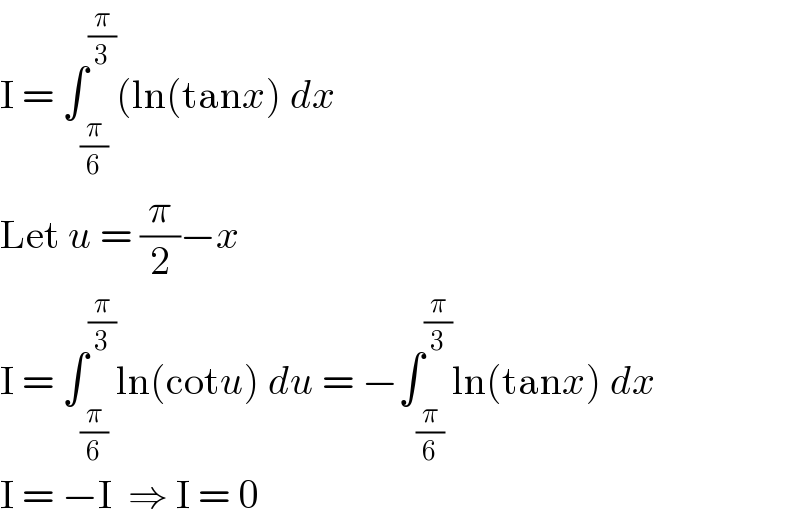
$$\mathrm{I}\:=\:\int_{\frac{\pi}{\mathrm{6}}} ^{\frac{\pi}{\mathrm{3}}} \left(\mathrm{ln}\left(\mathrm{tan}{x}\right)\:{dx}\right. \\ $$$$\mathrm{Let}\:{u}\:=\:\frac{\pi}{\mathrm{2}}βˆ’{x} \\ $$$$\mathrm{I}\:=\:\int_{\frac{\pi}{\mathrm{6}}} ^{\frac{\pi}{\mathrm{3}}} \mathrm{ln}\left(\mathrm{cot}{u}\right)\:{du}\:=\:βˆ’\int_{\frac{\pi}{\mathrm{6}}} ^{\frac{\pi}{\mathrm{3}}} \mathrm{ln}\left(\mathrm{tan}{x}\right)\:{dx} \\ $$$$\mathrm{I}\:=\:βˆ’\mathrm{I}\:\:\Rightarrow\:\mathrm{I}\:=\:\mathrm{0} \\ $$

Answered by mathmax by abdo last updated on 21/Jun/21

1) I=∫_(Ο€/6) ^(Ο€/3) log(tanx)dx changement tanx =t give I=∫_(1/( (√3))) ^(√3) ((logt)/(1+t^2 ))dt =_(t=(1/u)) βˆ’βˆ«_(1/( (√3))) ^(√3) ((βˆ’logu)/(1+(1/u^2 )))(βˆ’(du/u^2 )) =βˆ’βˆ«_(1/( (√3))) ^(√3) ((logu)/(1+u^2 ))=βˆ’I β‡’2I=0 β‡’I=0

$$\left.\mathrm{1}\right)\:\mathrm{I}=\int_{\frac{\pi}{\mathrm{6}}} ^{\frac{\pi}{\mathrm{3}}} \mathrm{log}\left(\mathrm{tanx}\right)\mathrm{dx}\:\:\mathrm{changement}\:\mathrm{tanx}\:=\mathrm{t}\:\mathrm{give} \\ $$$$\mathrm{I}=\int_{\frac{\mathrm{1}}{\:\sqrt{\mathrm{3}}}} ^{\sqrt{\mathrm{3}}} \:\frac{\mathrm{logt}}{\mathrm{1}+\mathrm{t}^{\mathrm{2}} }\mathrm{dt}\:\:=_{\mathrm{t}=\frac{\mathrm{1}}{\mathrm{u}}} \:\:βˆ’\int_{\frac{\mathrm{1}}{\:\sqrt{\mathrm{3}}}} ^{\sqrt{\mathrm{3}}} \:\frac{βˆ’\mathrm{logu}}{\mathrm{1}+\frac{\mathrm{1}}{\mathrm{u}^{\mathrm{2}} }}\left(βˆ’\frac{\mathrm{du}}{\mathrm{u}^{\mathrm{2}} }\right) \\ $$$$=βˆ’\int_{\frac{\mathrm{1}}{\:\sqrt{\mathrm{3}}}} ^{\sqrt{\mathrm{3}}} \:\frac{\mathrm{logu}}{\mathrm{1}+\mathrm{u}^{\mathrm{2}} }=βˆ’\mathrm{I}\:\Rightarrow\mathrm{2I}=\mathrm{0}\:\Rightarrow\mathrm{I}=\mathrm{0} \\ $$$$ \\ $$

Answered by mathmax by abdo last updated on 21/Jun/21

2)1βˆ’logx>0 β‡’logx<1 β‡’x<e f(x)=βˆ’((x(√(1βˆ’logx)))/(eβˆ’x)) changement eβˆ’x=y give f(x)=βˆ’(((eβˆ’y)(√(1βˆ’log(eβˆ’y))))/y)=(((yβˆ’e)(√(1βˆ’log(eβˆ’y))))/y)=g(y) (yβ†’o^+ ) we have log(eβˆ’y)=1+log(1βˆ’(y/e))∼1βˆ’(y/e) β‡’1βˆ’log(eβˆ’y)∼(y/e) β‡’(√(1βˆ’log(eβˆ’y)))∼((√y)/( (√e))) β‡’ g(y)∼((√y)/( (√e)y))(yβˆ’e) β‡’g(y)∼((yβˆ’e)/( (√e)(√y)))β†’βˆ’βˆž (yβ†’0^+ ) β‡’ lim_(xβ†’e^βˆ’ ) f(x)=βˆ’βˆž

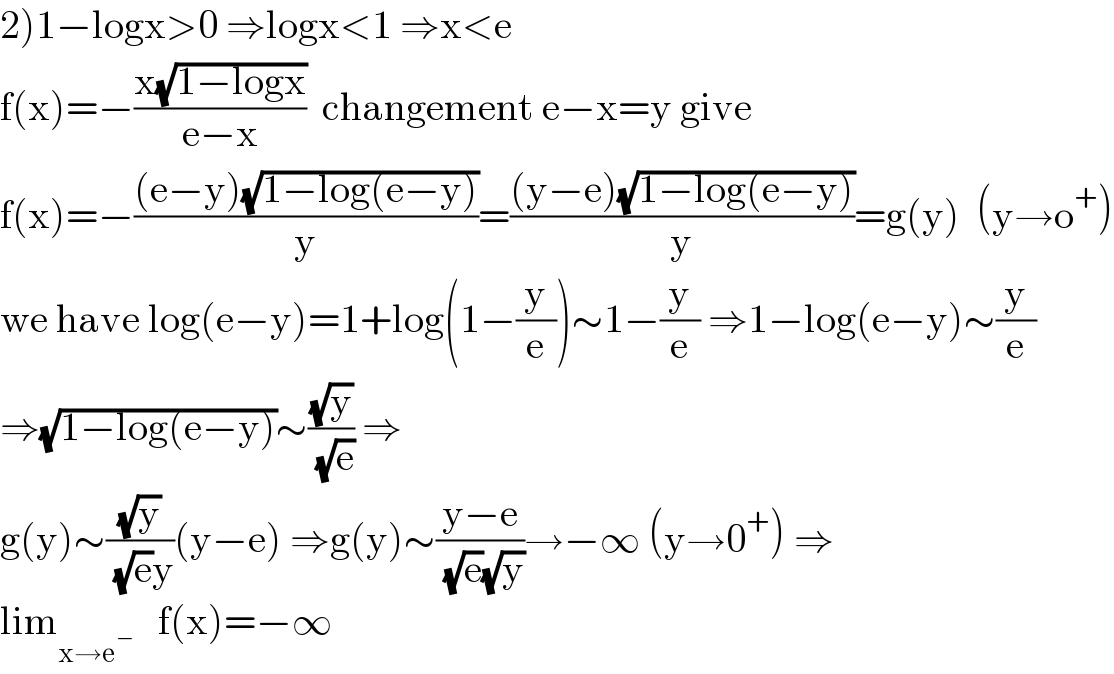
$$\left.\mathrm{2}\right)\mathrm{1}βˆ’\mathrm{logx}>\mathrm{0}\:\Rightarrow\mathrm{logx}<\mathrm{1}\:\Rightarrow\mathrm{x}<\mathrm{e} \\ $$$$\mathrm{f}\left(\mathrm{x}\right)=βˆ’\frac{\mathrm{x}\sqrt{\mathrm{1}βˆ’\mathrm{logx}}}{\mathrm{e}βˆ’\mathrm{x}}\:\:\mathrm{changement}\:\mathrm{e}βˆ’\mathrm{x}=\mathrm{y}\:\mathrm{give} \\ $$$$\mathrm{f}\left(\mathrm{x}\right)=βˆ’\frac{\left(\mathrm{e}βˆ’\mathrm{y}\right)\sqrt{\mathrm{1}βˆ’\mathrm{log}\left(\mathrm{e}βˆ’\mathrm{y}\right)}}{\mathrm{y}}=\frac{\left(\mathrm{y}βˆ’\mathrm{e}\right)\sqrt{\mathrm{1}βˆ’\mathrm{log}\left(\mathrm{e}βˆ’\mathrm{y}\right)}}{\mathrm{y}}=\mathrm{g}\left(\mathrm{y}\right)\:\:\left(\mathrm{y}\rightarrow\mathrm{o}^{+} \right) \\ $$$$\mathrm{we}\:\mathrm{have}\:\mathrm{log}\left(\mathrm{e}βˆ’\mathrm{y}\right)=\mathrm{1}+\mathrm{log}\left(\mathrm{1}βˆ’\frac{\mathrm{y}}{\mathrm{e}}\right)\sim\mathrm{1}βˆ’\frac{\mathrm{y}}{\mathrm{e}}\:\Rightarrow\mathrm{1}βˆ’\mathrm{log}\left(\mathrm{e}βˆ’\mathrm{y}\right)\sim\frac{\mathrm{y}}{\mathrm{e}} \\ $$$$\Rightarrow\sqrt{\mathrm{1}βˆ’\mathrm{log}\left(\mathrm{e}βˆ’\mathrm{y}\right)}\sim\frac{\sqrt{\mathrm{y}}}{\:\sqrt{\mathrm{e}}}\:\Rightarrow \\ $$$$\mathrm{g}\left(\mathrm{y}\right)\sim\frac{\sqrt{\mathrm{y}}}{\:\sqrt{\mathrm{e}}\mathrm{y}}\left(\mathrm{y}βˆ’\mathrm{e}\right)\:\Rightarrow\mathrm{g}\left(\mathrm{y}\right)\sim\frac{\mathrm{y}βˆ’\mathrm{e}}{\:\sqrt{\mathrm{e}}\sqrt{\mathrm{y}}}\rightarrowβˆ’\infty\:\left(\mathrm{y}\rightarrow\mathrm{0}^{+} \right)\:\Rightarrow \\ $$$$\mathrm{lim}_{\mathrm{x}\rightarrow\mathrm{e}^{βˆ’} } \:\:\:\mathrm{f}\left(\mathrm{x}\right)=βˆ’\infty \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com