Question and Answers Forum

All Questions      Topic List

Others Questions

Previous in All Question      Next in All Question      

Previous in Others      Next in Others      

Question Number 66115 by Rio Michael last updated on 09/Aug/19

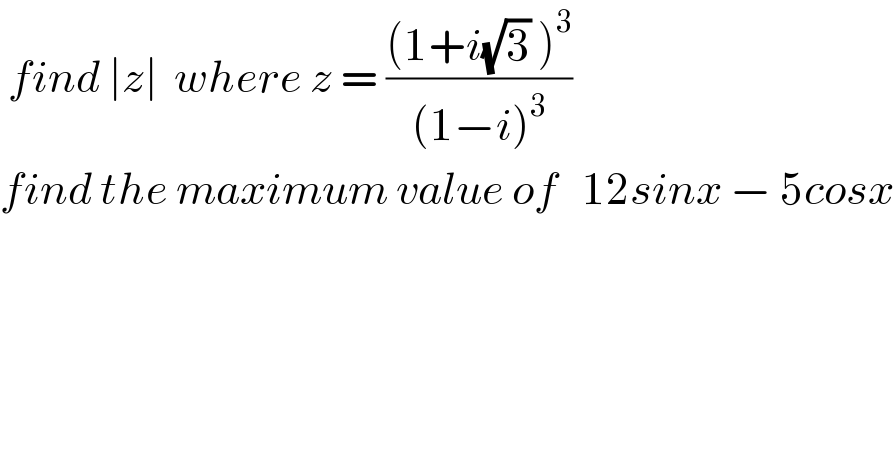
find ∣z∣ where z = (((1+i(√3) )^3 )/((1−i)^3 )) find the maximum value of 12sinx − 5cosx

$$\:{find}\:\mid{z}\mid\:\:{where}\:{z}\:=\:\frac{\left(\mathrm{1}+{i}\sqrt{\mathrm{3}}\:\right)^{\mathrm{3}} }{\left(\mathrm{1}−{i}\right)^{\mathrm{3}} } \\ $$$${find}\:{the}\:{maximum}\:{value}\:{of}\:\:\:\mathrm{12}{sinx}\:−\:\mathrm{5}{cosx} \\ $$

Commented by mathmax by abdo last updated on 09/Aug/19

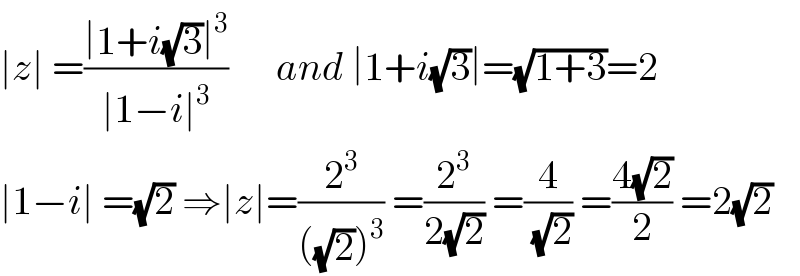
∣z∣ =((∣1+i(√3)∣^3 )/(∣1−i∣^3 )) and ∣1+i(√3)∣=(√(1+3))=2 ∣1−i∣ =(√2) ⇒∣z∣=(2^3 /(((√2))^3 )) =(2^3 /(2(√2))) =(4/(√2)) =((4(√2))/2) =2(√2)

$$\mid{z}\mid\:=\frac{\mid\mathrm{1}+{i}\sqrt{\mathrm{3}}\mid^{\mathrm{3}} }{\mid\mathrm{1}−{i}\mid^{\mathrm{3}} }\:\:\:\:\:\:{and}\:\mid\mathrm{1}+{i}\sqrt{\mathrm{3}}\mid=\sqrt{\mathrm{1}+\mathrm{3}}=\mathrm{2} \\ $$$$\mid\mathrm{1}−{i}\mid\:=\sqrt{\mathrm{2}}\:\Rightarrow\mid{z}\mid=\frac{\mathrm{2}^{\mathrm{3}} }{\left(\sqrt{\mathrm{2}}\right)^{\mathrm{3}} }\:=\frac{\mathrm{2}^{\mathrm{3}} }{\mathrm{2}\sqrt{\mathrm{2}}}\:=\frac{\mathrm{4}}{\sqrt{\mathrm{2}}}\:=\frac{\mathrm{4}\sqrt{\mathrm{2}}}{\mathrm{2}}\:=\mathrm{2}\sqrt{\mathrm{2}} \\ $$

Commented by mathmax by abdo last updated on 09/Aug/19

let f(x)=12sinx−5cosx f is 2π periodic f(x)=0 ⇔x=arctan((5/(12))) f^′ (x) =12cosx +5sinx f^′ (x)=0 ⇔12cosx +5sinx =0 ⇒5sinx =−12cosx ⇒ tanx =−((12)/5) ⇒ x =−arctan(((12)/5)) varation on [−π,π] x −π −arctan(((12)/5)) 0 arctan((5/(12))) π f(x) 5 decr α_0 ? −5 incr 0 inc 5 f(−arctan(((12)/5)))=−12sin(arctan(((12)/5)))−5cos(arctan(((12)/5))) =−12 (((12)/5)/(√(1+(((12)/5))^2 ))) −5 ×(1/(√(1+(((12)/5))^2 ))) =α_0 f(arctan((5/(12))))=12sin(arctan((5/(12))))−5cos(arctan((5/(12)))) =12 ×((5/(12))/(√(1+((5/(12)))^2 )))−5×(1/(√(1+((5/(12)))^2 ))) =0 ⇒max _(x∈R) f(x) =5

$${let}\:{f}\left({x}\right)=\mathrm{12}{sinx}−\mathrm{5}{cosx}\:\:\:{f}\:{is}\:\mathrm{2}\pi\:{periodic}\:\:\:{f}\left({x}\right)=\mathrm{0}\:\Leftrightarrow{x}={arctan}\left(\frac{\mathrm{5}}{\mathrm{12}}\right) \\ $$$${f}^{'} \left({x}\right)\:=\mathrm{12}{cosx}\:+\mathrm{5}{sinx}\: \\ $$$${f}^{'} \left({x}\right)=\mathrm{0}\:\Leftrightarrow\mathrm{12}{cosx}\:+\mathrm{5}{sinx}\:=\mathrm{0}\:\Rightarrow\mathrm{5}{sinx}\:=−\mathrm{12}{cosx}\:\Rightarrow \\ $$$${tanx}\:=−\frac{\mathrm{12}}{\mathrm{5}}\:\Rightarrow\:{x}\:=−{arctan}\left(\frac{\mathrm{12}}{\mathrm{5}}\right)\:{varation}\:{on}\:\left[−\pi,\pi\right] \\ $$$${x}\:\:\:\:\:\:\:−\pi\:\:\:\:\:\:\:\:\:\:−{arctan}\left(\frac{\mathrm{12}}{\mathrm{5}}\right)\:\:\:\:\:\:\mathrm{0}\:\:\:\:\:\:{arctan}\left(\frac{\mathrm{5}}{\mathrm{12}}\right)\:\:\:\:\:\:\:\:\:\:\:\pi \\ $$$${f}\left({x}\right)\:\:\:\mathrm{5}\:\:\:\:\:\:{decr}\:\:\:\:\:\:\:\:\:\:\:\alpha_{\mathrm{0}} \:\:\:\:\:\:\:\:?\:\:\:\:\:−\mathrm{5}\:\:{incr}\:\:\:\:\:\:\:\mathrm{0}\:\:\:\:\:\:\:{inc}\:\:\:\:\:\:\:\:\:\mathrm{5} \\ $$$${f}\left(−{arctan}\left(\frac{\mathrm{12}}{\mathrm{5}}\right)\right)=−\mathrm{12}{sin}\left({arctan}\left(\frac{\mathrm{12}}{\mathrm{5}}\right)\right)−\mathrm{5}{cos}\left({arctan}\left(\frac{\mathrm{12}}{\mathrm{5}}\right)\right) \\ $$$$=−\mathrm{12}\:\frac{\frac{\mathrm{12}}{\mathrm{5}}}{\sqrt{\mathrm{1}+\left(\frac{\mathrm{12}}{\mathrm{5}}\right)^{\mathrm{2}} }}\:−\mathrm{5}\:×\frac{\mathrm{1}}{\sqrt{\mathrm{1}+\left(\frac{\mathrm{12}}{\mathrm{5}}\right)^{\mathrm{2}} }}\:=\alpha_{\mathrm{0}} \\ $$$${f}\left({arctan}\left(\frac{\mathrm{5}}{\mathrm{12}}\right)\right)=\mathrm{12}{sin}\left({arctan}\left(\frac{\mathrm{5}}{\mathrm{12}}\right)\right)−\mathrm{5}{cos}\left({arctan}\left(\frac{\mathrm{5}}{\mathrm{12}}\right)\right) \\ $$$$=\mathrm{12}\:×\frac{\frac{\mathrm{5}}{\mathrm{12}}}{\sqrt{\mathrm{1}+\left(\frac{\mathrm{5}}{\mathrm{12}}\right)^{\mathrm{2}} }}−\mathrm{5}×\frac{\mathrm{1}}{\sqrt{\mathrm{1}+\left(\frac{\mathrm{5}}{\mathrm{12}}\right)^{\mathrm{2}} }}\:=\mathrm{0} \\ $$$$\Rightarrow{max}\:_{{x}\in{R}} {f}\left({x}\right)\:\:=\mathrm{5} \\ $$

Commented by Rio Michael last updated on 09/Aug/19

thank you sir

$${thank}\:{you}\:{sir} \\ $$

Commented by Prithwish sen last updated on 09/Aug/19

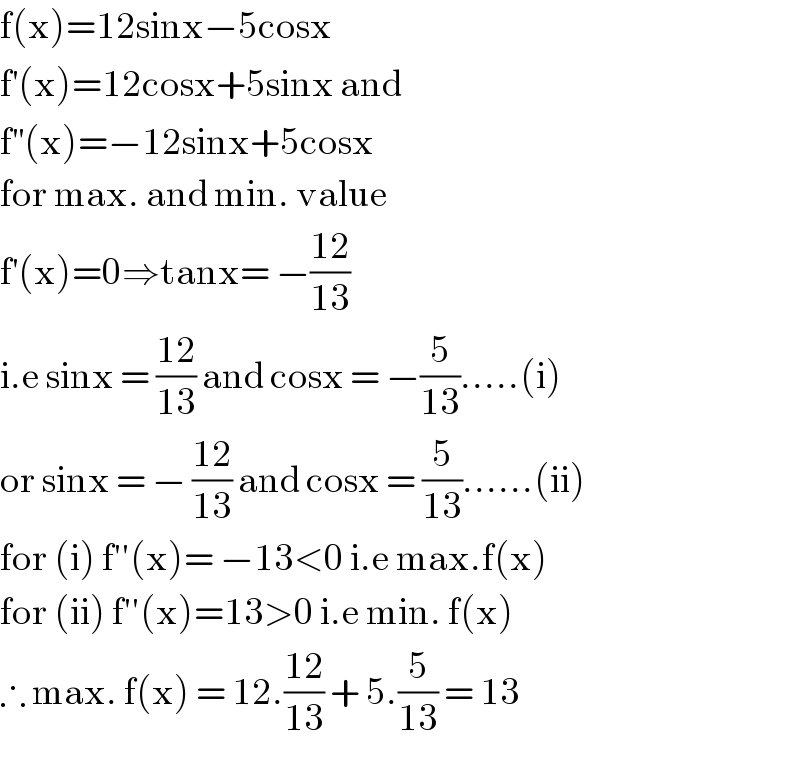
f(x)=12sinx−5cosx f^′ (x)=12cosx+5sinx and f^(′′) (x)=−12sinx+5cosx for max. and min. value f^′ (x)=0⇒tanx= −((12)/(13)) i.e sinx = ((12)/(13)) and cosx = −(5/(13)).....(i) or sinx = − ((12)/(13)) and cosx = (5/(13))......(ii) for (i) f′′(x)= −13<0 i.e max.f(x) for (ii) f′′(x)=13>0 i.e min. f(x) ∴ max. f(x) = 12.((12)/(13)) + 5.(5/(13)) = 13

$$\mathrm{f}\left(\mathrm{x}\right)=\mathrm{12sinx}−\mathrm{5cosx} \\ $$$$\mathrm{f}^{'} \left(\mathrm{x}\right)=\mathrm{12cosx}+\mathrm{5sinx}\:\mathrm{and} \\ $$$$\mathrm{f}^{''} \left(\mathrm{x}\right)=−\mathrm{12sinx}+\mathrm{5cosx} \\ $$$$\mathrm{for}\:\mathrm{max}.\:\mathrm{and}\:\mathrm{min}.\:\mathrm{value} \\ $$$$\mathrm{f}^{'} \left(\mathrm{x}\right)=\mathrm{0}\Rightarrow\mathrm{tanx}=\:−\frac{\mathrm{12}}{\mathrm{13}}\: \\ $$$$\mathrm{i}.\mathrm{e}\:\mathrm{sinx}\:=\:\frac{\mathrm{12}}{\mathrm{13}}\:\mathrm{and}\:\mathrm{cosx}\:=\:−\frac{\mathrm{5}}{\mathrm{13}}.....\left(\mathrm{i}\right) \\ $$$$\mathrm{or}\:\mathrm{sinx}\:=\:−\:\frac{\mathrm{12}}{\mathrm{13}}\:\mathrm{and}\:\mathrm{cosx}\:=\:\frac{\mathrm{5}}{\mathrm{13}}......\left(\mathrm{ii}\right) \\ $$$$\mathrm{for}\:\left(\mathrm{i}\right)\:\mathrm{f}''\left(\mathrm{x}\right)=\:−\mathrm{13}<\mathrm{0}\:\mathrm{i}.\mathrm{e}\:\mathrm{max}.\mathrm{f}\left(\mathrm{x}\right) \\ $$$$\mathrm{for}\:\left(\mathrm{ii}\right)\:\mathrm{f}''\left(\mathrm{x}\right)=\mathrm{13}>\mathrm{0}\:\mathrm{i}.\mathrm{e}\:\mathrm{min}.\:\mathrm{f}\left(\mathrm{x}\right) \\ $$$$\therefore\:\mathrm{max}.\:\mathrm{f}\left(\mathrm{x}\right)\:=\:\mathrm{12}.\frac{\mathrm{12}}{\mathrm{13}}\:+\:\mathrm{5}.\frac{\mathrm{5}}{\mathrm{13}}\:=\:\mathrm{13} \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com