Question and Answers Forum

All Questions      Topic List

Integration Questions

Previous in All Question      Next in All Question      

Previous in Integration      Next in Integration      

Question Number 68037 by mathmax by abdo last updated on 03/Sep/19

find ∫ ((x^2 dx)/((x^3 −8)(x^4 +1)))

$${find}\:\int\:\:\:\frac{{x}^{\mathrm{2}} {dx}}{\left({x}^{\mathrm{3}} −\mathrm{8}\right)\left({x}^{\mathrm{4}} \:+\mathrm{1}\right)} \\ $$

Answered by MJS last updated on 04/Sep/19

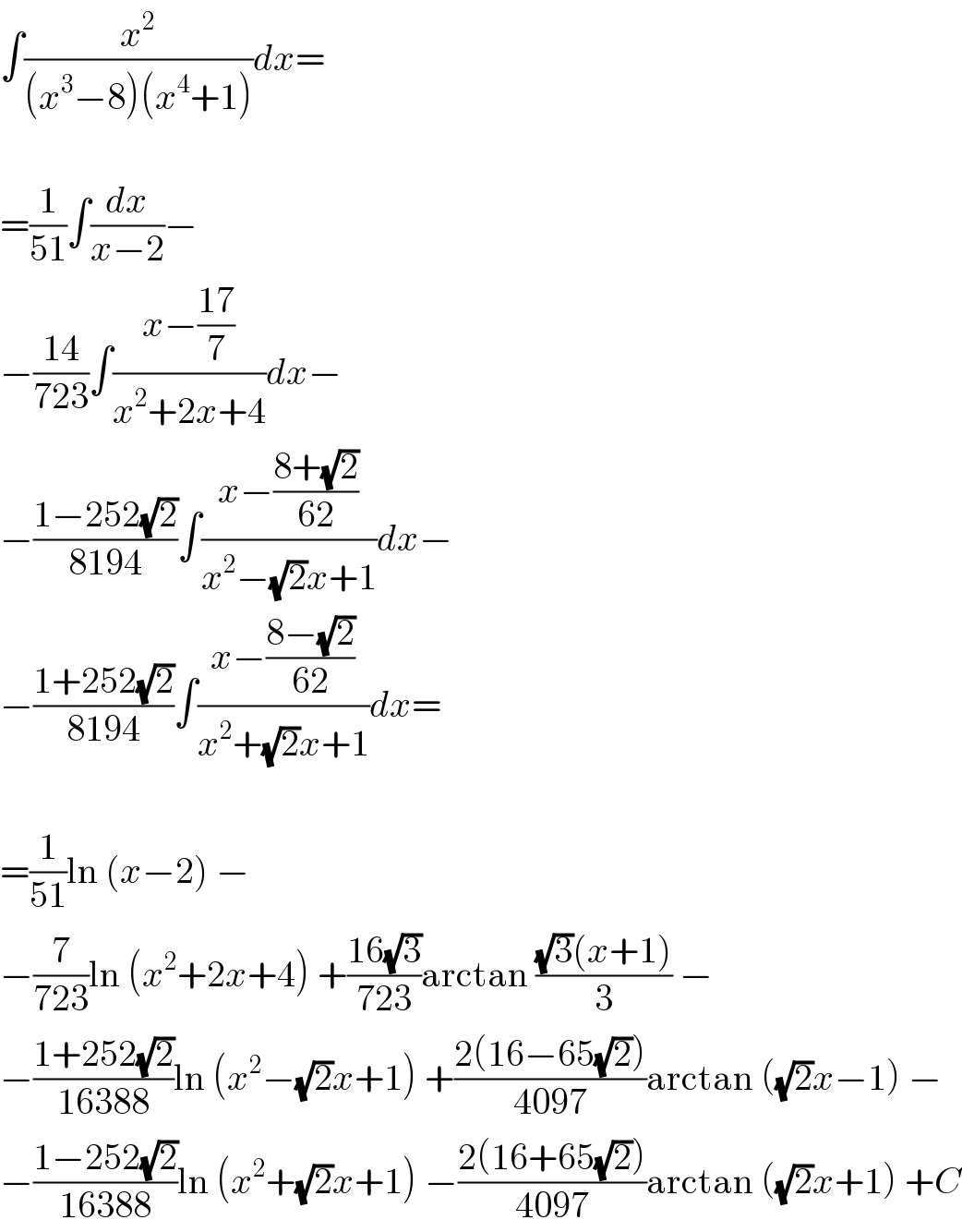
∫(x^2 /((x^3 −8)(x^4 +1)))dx= =(1/(51))∫(dx/(x−2))− −((14)/(723))∫((x−((17)/7))/(x^2 +2x+4))dx− −((1−252(√2))/(8194))∫((x−((8+(√2))/(62)))/(x^2 −(√2)x+1))dx− −((1+252(√2))/(8194))∫((x−((8−(√2))/(62)))/(x^2 +(√2)x+1))dx= =(1/(51))ln (x−2) − −(7/(723))ln (x^2 +2x+4) +((16(√3))/(723))arctan (((√3)(x+1))/3) − −((1+252(√2))/(16388))ln (x^2 −(√2)x+1) +((2(16−65(√2)))/(4097))arctan ((√2)x−1) − −((1−252(√2))/(16388))ln (x^2 +(√2)x+1) −((2(16+65(√2)))/(4097))arctan ((√2)x+1) +C

$$\int\frac{{x}^{\mathrm{2}} }{\left({x}^{\mathrm{3}} −\mathrm{8}\right)\left({x}^{\mathrm{4}} +\mathrm{1}\right)}{dx}= \\ $$$$ \\ $$$$=\frac{\mathrm{1}}{\mathrm{51}}\int\frac{{dx}}{{x}−\mathrm{2}}− \\ $$$$−\frac{\mathrm{14}}{\mathrm{723}}\int\frac{{x}−\frac{\mathrm{17}}{\mathrm{7}}}{{x}^{\mathrm{2}} +\mathrm{2}{x}+\mathrm{4}}{dx}− \\ $$$$−\frac{\mathrm{1}−\mathrm{252}\sqrt{\mathrm{2}}}{\mathrm{8194}}\int\frac{{x}−\frac{\mathrm{8}+\sqrt{\mathrm{2}}}{\mathrm{62}}}{{x}^{\mathrm{2}} −\sqrt{\mathrm{2}}{x}+\mathrm{1}}{dx}− \\ $$$$−\frac{\mathrm{1}+\mathrm{252}\sqrt{\mathrm{2}}}{\mathrm{8194}}\int\frac{{x}−\frac{\mathrm{8}−\sqrt{\mathrm{2}}}{\mathrm{62}}}{{x}^{\mathrm{2}} +\sqrt{\mathrm{2}}{x}+\mathrm{1}}{dx}= \\ $$$$ \\ $$$$=\frac{\mathrm{1}}{\mathrm{51}}\mathrm{ln}\:\left({x}−\mathrm{2}\right)\:− \\ $$$$−\frac{\mathrm{7}}{\mathrm{723}}\mathrm{ln}\:\left({x}^{\mathrm{2}} +\mathrm{2}{x}+\mathrm{4}\right)\:+\frac{\mathrm{16}\sqrt{\mathrm{3}}}{\mathrm{723}}\mathrm{arctan}\:\frac{\sqrt{\mathrm{3}}\left({x}+\mathrm{1}\right)}{\mathrm{3}}\:− \\ $$$$−\frac{\mathrm{1}+\mathrm{252}\sqrt{\mathrm{2}}}{\mathrm{16388}}\mathrm{ln}\:\left({x}^{\mathrm{2}} −\sqrt{\mathrm{2}}{x}+\mathrm{1}\right)\:+\frac{\mathrm{2}\left(\mathrm{16}−\mathrm{65}\sqrt{\mathrm{2}}\right)}{\mathrm{4097}}\mathrm{arctan}\:\left(\sqrt{\mathrm{2}}{x}−\mathrm{1}\right)\:− \\ $$$$−\frac{\mathrm{1}−\mathrm{252}\sqrt{\mathrm{2}}}{\mathrm{16388}}\mathrm{ln}\:\left({x}^{\mathrm{2}} +\sqrt{\mathrm{2}}{x}+\mathrm{1}\right)\:−\frac{\mathrm{2}\left(\mathrm{16}+\mathrm{65}\sqrt{\mathrm{2}}\right)}{\mathrm{4097}}\mathrm{arctan}\:\left(\sqrt{\mathrm{2}}{x}+\mathrm{1}\right)\:+{C} \\ $$

Commented by turbo msup by abdo last updated on 04/Sep/19

thank you sir mjs

$${thank}\:{you}\:{sir}\:{mjs} \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com