
Question Number 182368 by universe last updated on 08/Dec/22

$$\:\:\:\:\mathrm{find}\:\mathrm{volume}\:\mathrm{of}\:\mathrm{region}\:\mathrm{in}\:\:\mathbb{R}^{\mathrm{3}} \:\:\mathrm{given}\:\mathrm{by}\: \\ $$$$\:\:\:\:\mathrm{3}\mid\mathrm{x}\mid\:+\:\mathrm{4}\mid\mathrm{y}\mid\:+\mathrm{3}\mid\mathrm{z}\mid\:\leqslant\mathrm{12}\:\:\mathrm{is} \\ $$
Answered by mr W last updated on 08/Dec/22

$$\frac{\mid{x}\mid}{\mathrm{4}}+\frac{\mid{y}\mid}{\mathrm{3}}+\frac{\mid{z}\mid}{\mathrm{4}}\leqslant\mathrm{1} \\ $$$${V}=\mathrm{8}×\frac{\mathrm{4}×\mathrm{3}×\mathrm{4}}{\mathrm{6}}=\mathrm{64} \\ $$
Commented by universe last updated on 09/Dec/22
$${sorry}\:{sir}\:{i}\:{dont}\:{get}\:{it} \\ $$$${please}\:{explain}\:{little}\:{more} \\ $$
Commented by mr W last updated on 09/Dec/22
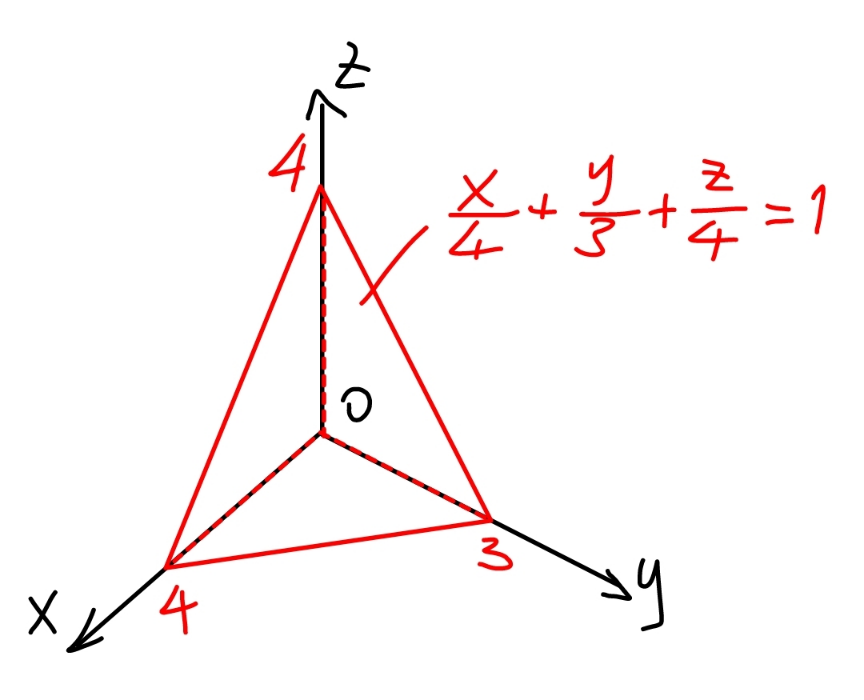
Commented by mr W last updated on 09/Dec/22
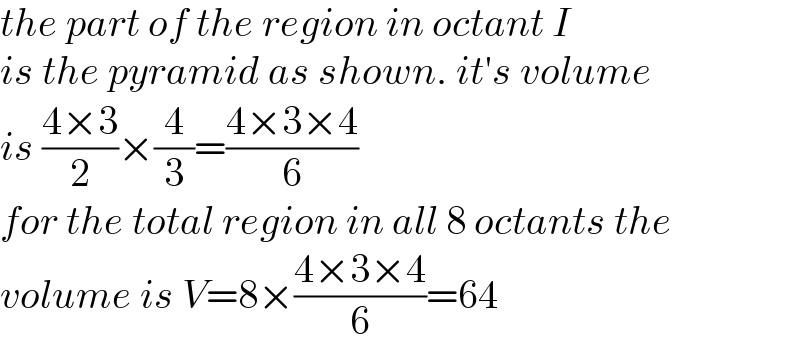
$${the}\:{part}\:{of}\:{the}\:{region}\:{in}\:{octant}\:{I} \\ $$$${is}\:{the}\:{pyramid}\:{as}\:{shown}.\:{it}'{s}\:{volume} \\ $$$${is}\:\frac{\mathrm{4}×\mathrm{3}}{\mathrm{2}}×\frac{\mathrm{4}}{\mathrm{3}}=\frac{\mathrm{4}×\mathrm{3}×\mathrm{4}}{\mathrm{6}} \\ $$$${for}\:{the}\:{total}\:{region}\:{in}\:{all}\:\mathrm{8}\:{octants}\:{the} \\ $$$${volume}\:{is}\:{V}=\mathrm{8}×\frac{\mathrm{4}×\mathrm{3}×\mathrm{4}}{\mathrm{6}}=\mathrm{64} \\ $$
Commented by mr W last updated on 09/Dec/22
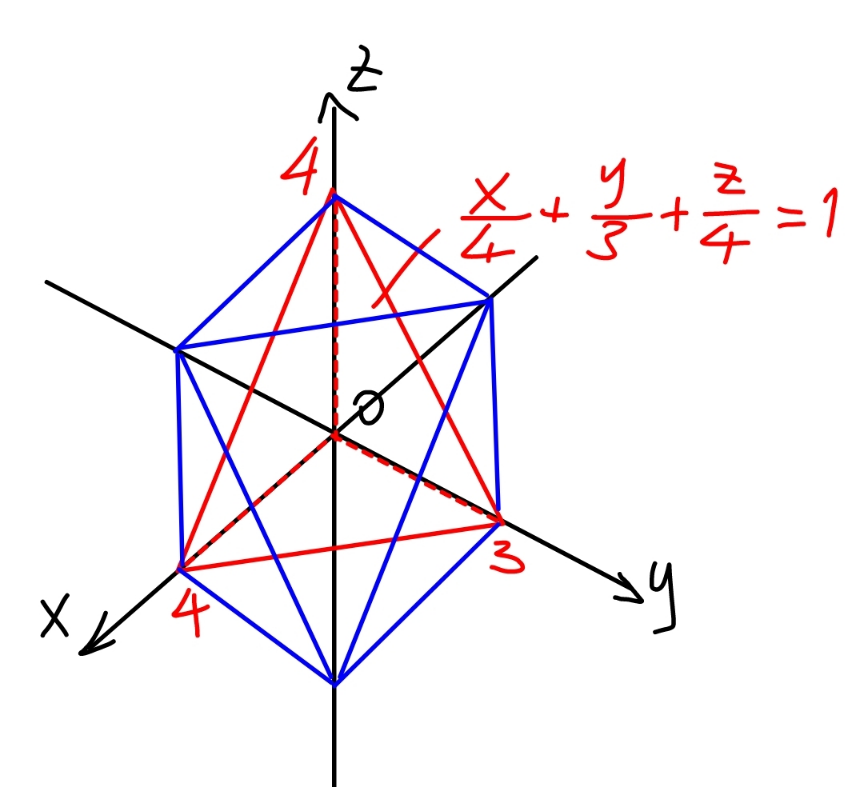
Commented by universe last updated on 09/Dec/22

$${thanks}\:{sir} \\ $$
